The length of the diameter of the circle which touches the x-axis at the point (1, 0) and passes through the point (2, 3)
103
35
65
53
The correct Answer is:A
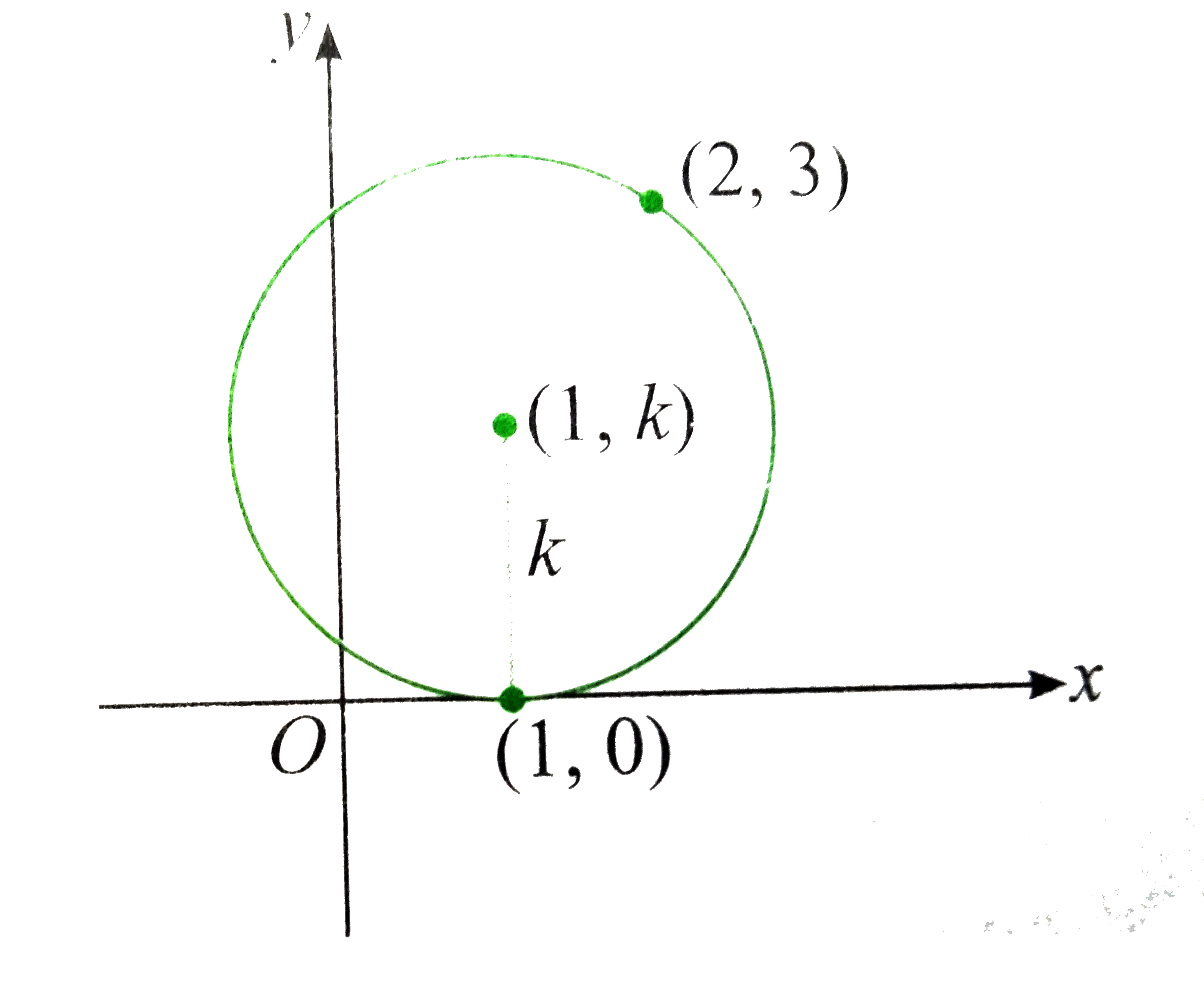
Circle touches x-axis at (1,0).
So, center of circle is (1,k) and radius is k.
Hence , equation of circle is :
(x−1)2+(y−k)2=k2
It passes through the point (2,3), so
(2−1)2+(3−k)2=k2
∴1+9−6k+k2=k2
∴k=5/3
∴ diameter =2k=10/3
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - JEE ADVANCED (Single Correct Answer Type)
3 Videos
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - LINKED COMPREHENSION TYPE (For problem 1 and 2)
2 Videos
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - ARCHIVES (JEE MAIN)
1 Videos
- BINOMIAL THEOREMBOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
- CIRCLESBOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
Similar Questions
Knowledge Check
The length of the radius of the circle which touches the x - axis at the point (1, 0) and passes through the point (2, 3) is
A103 unitsB35 unitsC65 unitsD53 unitsThe length of the diameter of the circle which passes through the point (2,3) and touches the x-axis at the point (1,0) is
A53B65C103D35
Find the equation of the circle which touches the axis of x and passes through the two points (1,-2) and (3,-4)
View SolutionSum of the abscissa and ordinate of the centre of the circle touching the line 3x+y+2=0 at the point (−1,1) and passing through the point (3,5) is-
View SolutionA circle having its centre in the first quadrant touches the y-axis at the point (0,2) and passes through the point (1,0). Find the equation of the circle
View SolutionEquation of the smaller circle that touches the circle x2+y2=1 and passes through the point (4,3) is
View SolutionEquation of the smaller circle that touches the circle x2+y2=1 and passes through the point (4,3) is
View SolutionThe equation of radical axis of two circles is x+y=1. One of the circles has the ends ofa diameter at the points (1,−3)and(4,1) and the other passes through the point (1, 2).Find the equating of these circles.
View Solution
