The point of intersection of the tangents at the point P on the ellipse x2a2+y2b2=1 and its corresponding point Q on the auxiliary circle meet on the line x=ae (b) x=0 y=0 (d) none of theseSolution in Bengali
x=a/e
x=0
y=0
none of these
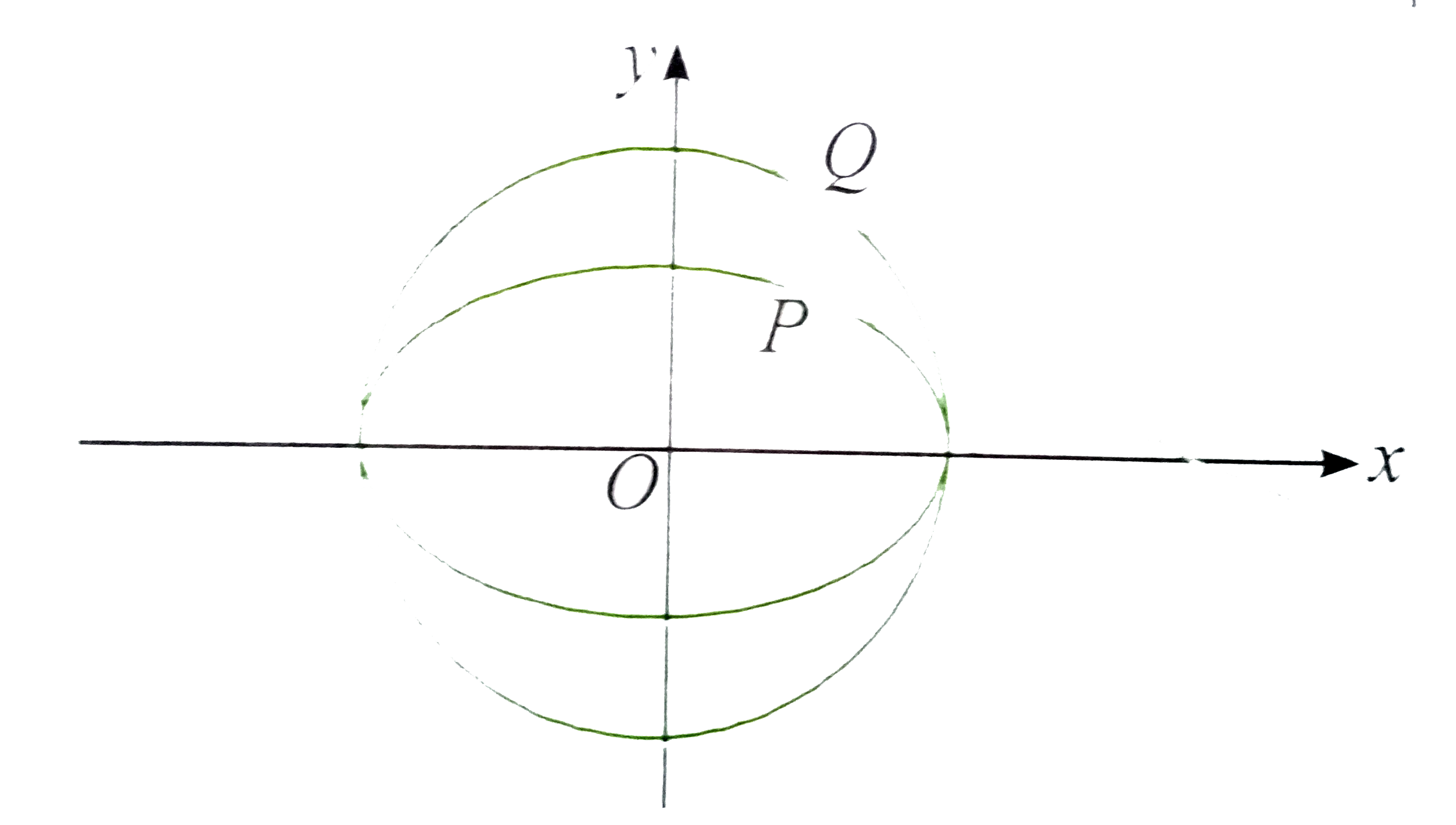
Tangent to the ellipse at point P(acosθ,asinθ) is xacosthea+ybsinθ=1(1)
Tangent (1) and (2) intersect at (a/cosθ,0) which lies on y=0
Similar Questions
If the circle x^(2)+y^(2)=1 A variable tangent of the ellipse x^(2)+2y^(2)=4 Is cut at points P and Q. Then the point of intersection of the tangent lines drawn at P and Q is
04:43View SolutionIf the tangent to the ellipse x2+2y2=1 at point P(1√2,12) meets the auxiliary circle at point RandQ , then find the points of intersection of tangents to the circle at QandR.
03:24View SolutionThe point of intersection of the tangents at the point P on the ellipse x2a2+y2b2=1 and its corresponding point Q on the auxiliary circle meet on the line (a) x=ae (b) x=0 (c) y=0 (d) none of these
02:38View Solutionदीर्घवृत्त x2a2+y2b2=1 के बिन्दु P पर तथा सहायक वृत्त पर इसके संगत बिन्दु Q पर स्पर्श रेखाओं के प्रतिच्छेद बिन्दु निम्न रेखा पर मिलेंगे -
05:54View SolutionThe locus of the point of intersection of tangents to the ellipse x2a2+y2b2=1 which meet at right , is
05:15View SolutionThe point of intersection of the tangents at the point P on the ellipse (x^2)/(a^2)+(y^2)/(b^2)=1 and its corresponding point Q on the auxiliary circle meet on the line (a) x=a/e (b) x=0 (c) y=0 (d) none of these
09:17View SolutionIf the tangent to the ellipse x^2+2y^2=1 at point P(1/(sqrt(2)),1/2) meets the auxiliary circle at point R and Q , then find the points of intersection of tangents to the circle at Q and Rdot
03:21View SolutionThe point of intersection of the tangents at the point P on the ellipse (x^2)/(a^2)+(y^2)/(b^2)=1 and its corresponding point Q on the auxiliary circle meet on the line (a) x=a/e (b) x=0 (c) y=0 (d) none of these
03:37View Solution

