Tangents are drawn to the ellipse and the circle at the points where a common ordinate cuts them (on the same side of the x-axis). Then the greatest acute angle between these tangents is given by (b) (d) Solution in Bengali
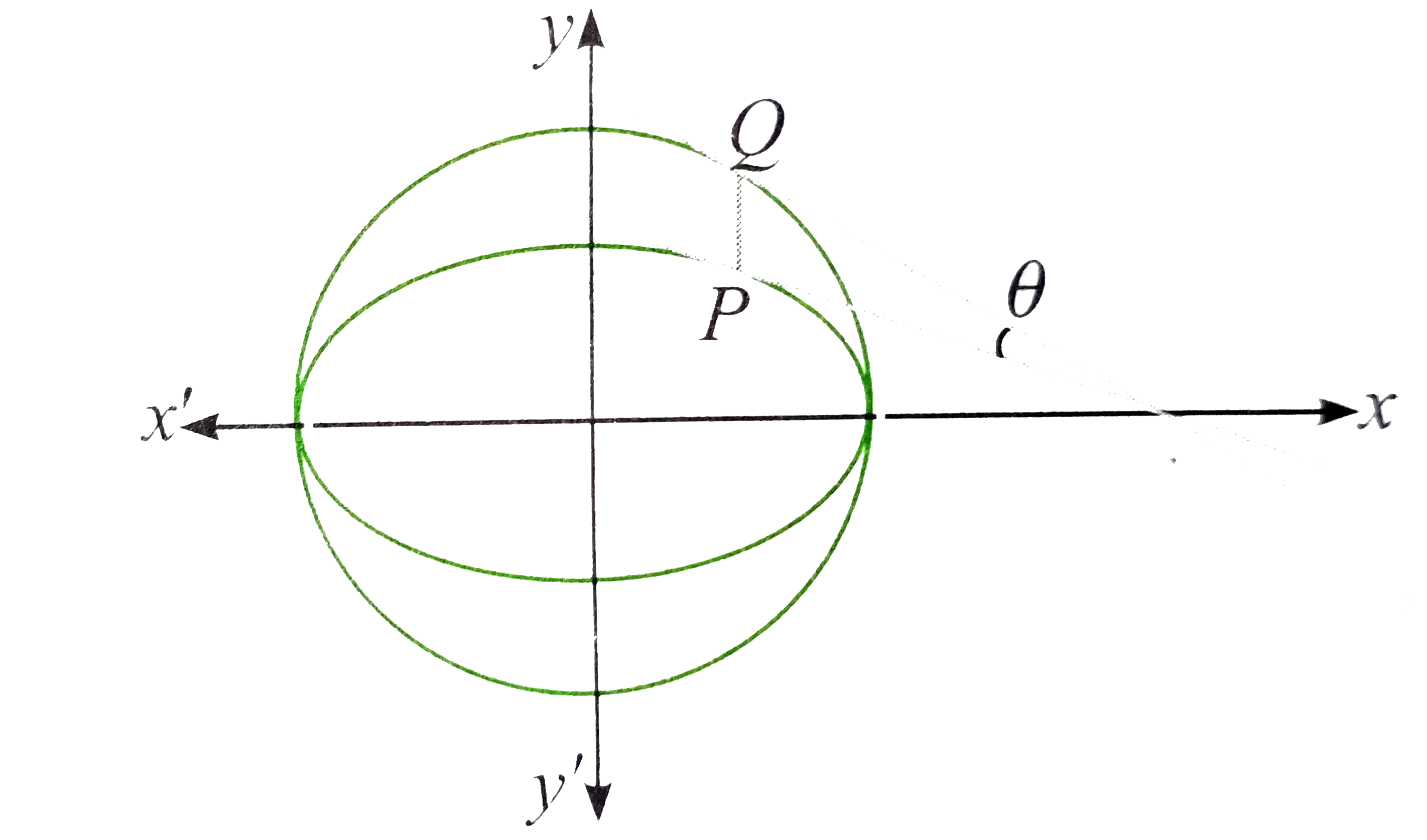
Tanent to the ellipse at is
Tangent to the circle at is
Now, the angle between the tangents is . Then,
Now, the greatest value of the above expression is when . Therefore,
Similar Questions
ddx{2√a2−b2Tan−1(√a−ba+btan(x)2)}=
04:53View SolutionIf y=2√a2−b2tan−1[√a−ba+btanx2]
View SolutionIf y=2√a2−b2tan−1[√a−ba+btanx2], prove that dydx=1a+bcosx,a>b>0.
06:14View SolutionIf two different tangents of y2=4x are the normals to x2=4by, then (a)|b|>12√2 (b) |b|<12√2 (c)|b|>1√2 (d) |b|<1√2
02:39View SolutionTangents are drawn to the ellipse x2a2+y2b2=1,(a>b), and the circle x2+y2=a2 at the points where a common ordinate cuts them (on the same side of the x-axis). Then the greatest acute angle between these tangents is given by (A) tan−1(a−b2√ab) (B) tan−1(a+b2√ab) (C) tan−1(2ab√a−b) (D) tan−1(2ab√a+b)
10:04View SolutionFind the tangent of the angle between the lines whose intercepts on the axes are respectively a,−b and b,−a ............... A) tan−1a2+b2ab B) tan−1b2−a22 C) tan−1b2−a22ab D) tan−1b2−a2ab
04:49View SolutionIf y=2/sqrt(a^2-b^2)tan^-1[sqrt((a-b)/(a+b))tan(x/2)] then (d_2y)/(dx^2)|(x=pi/2)|
09:26View SolutionIf two different tangents of y2=4x are the normals to x2=4by, then (a)|b|>12√2 (b) |b|<12√2 (c)|b|>1√2 (d) |b|<1√2
05:33View Solution

