If the ellipse x2a2+y2b2=1 is inscribed in a rectangle whose length to breadth ratio is 2:1 , then the area of the rectangle is 4.a2+b27 (b) 4.a2+b23 12.a2+b25 (d) 8.a2+b25Solution in Bengali
4⋅a2+b27
4⋅a2+b23
12⋅a2+b25
8⋅a2+b25
Since mutually perpendicualar tangents can be drawn from the vartices of the reactangle , all the vertices of the rectangle should lie on the director circle x2+y2=a2+b2
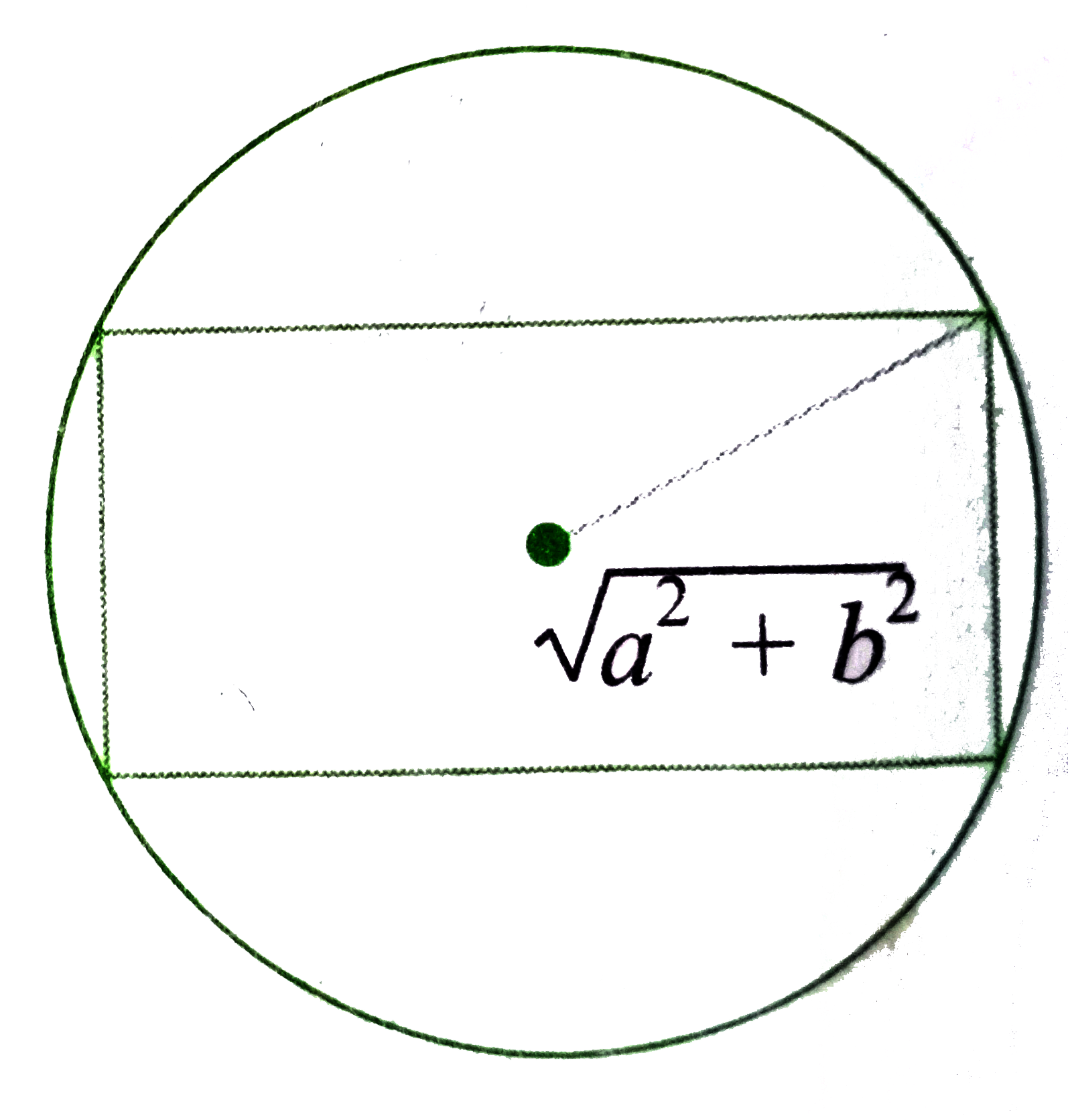
Let breadht =2l and length =4l . then,
l2+(2l)2=a2+b2
or l2=a2+b25
or Area of 4l×2l=8(a2+b25)
Similar Questions
If the ellipse x2a2+y2b2=1 is inscribed in a rectangle whose length to breadth ratio is 2 :1 then the area of the rectangle is
02:41View SolutionFind the area of the greatest rectangle that can be inscribed in an ellipse (x^(2))/(a^(2)) + (y^(2))/(b^(2)) = 1 .
04:41View SolutionIf ellipses (x^(2))/(a^(2))+(y^(2))/(b^(2))=1 Is drawn inside a rectangle whose length and width ratio is 2: 1, then the area of the rectangle is
04:04View SolutionFind the area of the greatest rectangle that can be inscribed in an ellipse x2a2+y2b2=1.
05:43View SolutionIf the ellipse x2a2+y2b2=1 is inscribed in a rectangle whose length to breadth ratio is 2:1 , then the area of the rectangle is 4.a2+b27 (b) 4.a2+b23 12.a2+b25 (d) 8.a2+b25
02:11View SolutionArea of the greatest rectangle that can be inscribed in the ellipse x^2/a^2+y^2/b^2=1 is
04:24View SolutionIf the ellipse x2a2+y2b2=1 is inscribed in a rectangle whose length to breadth ratio is 2:1 , then the area of the rectangle is (a)4.a2+b27 (b) 4.a2+b23 (c)12.a2+b25 (d) 8.a2+b25
03:30View SolutionFind the area of the greatest rectangle that can be inscribed in the ellipse (x^2)/(a^2)+(y^2)/(b^2)=1.
04:06View Solution

