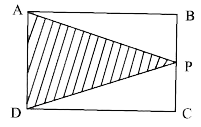
ABCD is a parallelogram P and R are two points on AB such that the area of parallelogram ABCD is 8 times the area of Δ DPR. If PR = 5 cm, then CD is equal to
10 cm
5 cm
20 cm
12 cm
The correct Answer is:C
To solve the problem step by step, we need to find the length of CD in the parallelogram ABCD given that the area of parallelogram ABCD is 8 times the area of triangle DPR and that PR = 5 cm.
1. Understand the Given Information:
- ABCD is a parallelogram.
- P and R are points on AB.
- Area of parallelogram ABCD = 8 × Area of triangle DPR.
- Length of PR = 5 cm.
2. Area of Parallelogram:
- The formula for the area of a parallelogram is:
Area of parallelogram=Base×Height
- Let the base be AB and the height be h. Thus:
Area of parallelogram ABCD=AB×h
3. Area of Triangle DPR:
- The formula for the area of a triangle is:
Area of triangle=12×Base×Height
- Here, the base is PR (which is 5 cm) and the height is also h (same height as the parallelogram). Thus:
Area of triangle DPR=12×PR×h=12×5×h=5h2
4. Set Up the Equation:
- According to the problem, the area of parallelogram ABCD is 8 times the area of triangle DPR:
AB×h=8×(5h2)
5. Simplify the Equation:
- Simplifying the right side:
AB×h=8×5h2=20h
- Now, we can cancel h from both sides (assuming h ≠ 0):
AB=20 cm
6. Find CD:
- In a parallelogram, opposite sides are equal. Therefore, CD is equal to AB:
CD=AB=20 cm
Final Answer:
CD = 20 cm.
- ABCD is a parallelogram.
- P and R are points on AB.
- Area of parallelogram ABCD = 8 × Area of triangle DPR.
- Length of PR = 5 cm.
- The formula for the area of a parallelogram is:
Area of parallelogram=Base×Height
- Let the base be AB and the height be h. Thus:
Area of parallelogram ABCD=AB×h
- The formula for the area of a triangle is:
Area of triangle=12×Base×Height
- Here, the base is PR (which is 5 cm) and the height is also h (same height as the parallelogram). Thus:
Area of triangle DPR=12×PR×h=12×5×h=5h2
- According to the problem, the area of parallelogram ABCD is 8 times the area of triangle DPR:
AB×h=8×(5h2)
- Simplifying the right side:
AB×h=8×5h2=20h
- Now, we can cancel h from both sides (assuming h ≠ 0):
AB=20 cm
- In a parallelogram, opposite sides are equal. Therefore, CD is equal to AB:
CD=AB=20 cm
CD = 20 cm.
Topper's Solved these Questions
- BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - PERIMETER AND AREA EXERCISE - SELF ASSESSMENT SHEET10videos
PERIMETER AND AREA
- BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - PERIMETER AND AREA EXERCISE - QUESTION BANK - 21(B)21videos
PERIMETER AND AREA
- BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - PERCENTAGE AND ITS APPLICATIONSEXERCISE - Self Assessment Sheet - 1010videos
PERCENTAGE AND ITS APPLICATIONS
- BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - POLYGONS AND QUADRILATERALSEXERCISE - Self Assessment Sheet10videos
POLYGONS AND QUADRILATERALS
Similar Questions
Knowledge Check
- Question 1 - Select One
In the figure, the area of parallelogram ABCD is
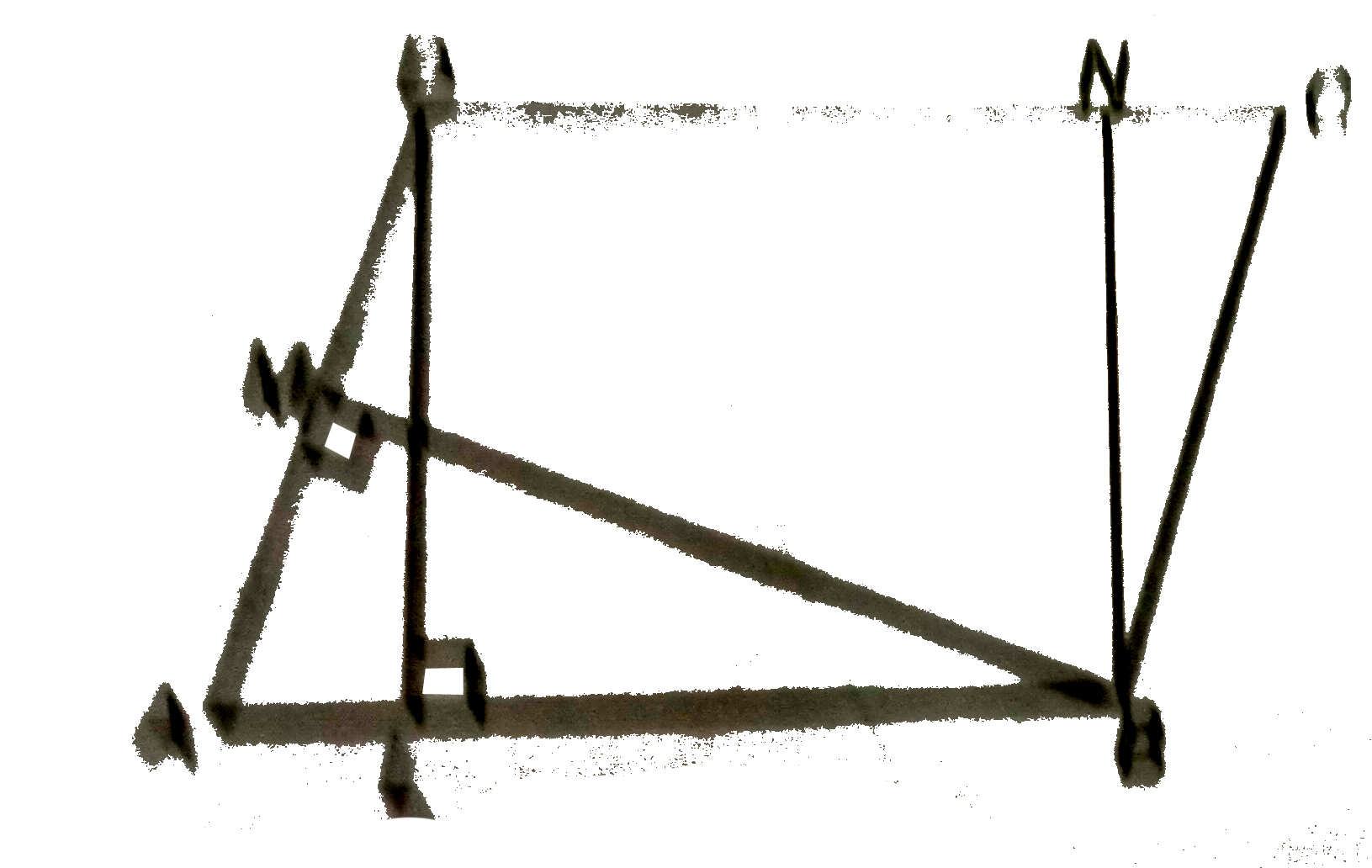 AAB×BMBBC×BNCDC×DLDAD×DL
AAB×BMBBC×BNCDC×DLDAD×DL - Question 2 - Select One
ABCD is a parallelogram, E and F are the mid-points of BC and CD. Find the ratio of area of parallelogram ABCD and ΔAEF
A2:1B4:1C8:3D8:1 - Question 3 - Select One
ABCD is a parallelogram and m ∠DAB=30∘, BC=20 cm and AB=20 cm. Find the area of parallelogram:
A150cm2B200cm2C400cm2D260cm2
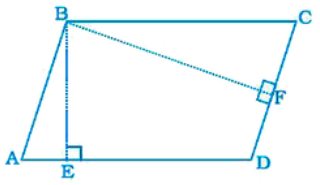
Area of parallelogram ABCD (Fig. 9.16) is not equal to
 View Solution
View Solution
What is the area of the parallelogram ABCD as shown in the given figure?View SolutionLet LMNP be a parallelogram and NR be perpendicular to LP. If the area of the parallelogram is six times the area of triangle RNP and RP = 6 cm, what is LR equal to ?
View SolutionABCD is a parallelogram. P and Q are mid points of BC & CD. Find the area of ΔAPQ if area of ΔABC is 12.
View SolutionABCD is a parallelogram, ∠DAB=30∘ BC = 20 cm and AB = 40cm. Find the area of parallelogram :
View Solution