2 cm of rain has fallen on a square kilometer of land. Assuming that 50% of the rain drops could have been collected and contained in a pool having a 100m×10m base, by what level would the water level in the pool have increased?
15 m
20 m
10 m
25 m
The correct Answer is:C
To solve the problem, we need to follow these steps:
Step 1: Calculate the volume of rain that fell on the land.
- The area of the land is given as 1 square kilometer, which is equal to 1,000,000m2.
- The height of the rain is given as 2 cm, which is equal to 0.02m.
- The volume of rain that fell can be calculated using the formula:
Volume=Area×Height
Volume=1,000,000m2×0.02m=20,000m3
Step 2: Calculate the volume of rain that can be collected.
- Since only 50% of the rain can be collected, we calculate:
Collected Volume=0.5×20,000m3=10,000m3
Step 3: Calculate the dimensions of the pool.
- The base area of the pool is given as 100m×10m.
- Therefore, the base area of the pool is:
Base Area=100m×10m=1,000m2
Step 4: Calculate the increase in water level in the pool.
- To find the increase in water level, we use the formula:
Increase in Height=Collected VolumeBase Area
Increase in Height=10,000m31,000m2=10m
Final Answer:
The water level in the pool would have increased by 10 meters.
---
- The area of the land is given as 1 square kilometer, which is equal to 1,000,000m2.
- The height of the rain is given as 2 cm, which is equal to 0.02m.
- The volume of rain that fell can be calculated using the formula:
Volume=Area×Height
Volume=1,000,000m2×0.02m=20,000m3
- Since only 50% of the rain can be collected, we calculate:
Collected Volume=0.5×20,000m3=10,000m3
- The base area of the pool is given as 100m×10m.
- Therefore, the base area of the pool is:
Base Area=100m×10m=1,000m2
- To find the increase in water level, we use the formula:
Increase in Height=Collected VolumeBase Area
Increase in Height=10,000m31,000m2=10m
The water level in the pool would have increased by 10 meters.
Topper's Solved these Questions
- VOLUME AND SURFACE AREA OF A CUBE AND A CUBOIDBOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - VOLUME AND SURFACE AREA OF A CUBE AND A CUBOIDEXERCISE - SELF ASSESSMENT SHEET - 23
10 Videos
- VOLUME AND SURFACE AREA OF A CUBE AND A CUBOIDBOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - VOLUME AND SURFACE AREA OF A CUBE AND A CUBOIDEXERCISE - UNIT TEST - 5
20 Videos
- VOLUME AND SURFACE AREA OF A CUBE AND A CUBOIDBOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - VOLUME AND SURFACE AREA OF A CUBE AND A CUBOIDEXERCISE - UNIT TEST - 5
20 Videos
- TRIANGLESBOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - TRIANGLESEXERCISE - Self Assessment Sheet - 18
10 Videos
Similar Questions
The rain which falls on a roof 18m long and 16.5m wide is allowed to be stored in a cylindrical tank 8m in diameter. If it rains 10cm on a day, what is the rise of water level in the tank due to it?
View SolutionAssuming ideal gas behaviour, how many atoms of Ar are contained in a typical human breath of 0.5 litre at 1.0 bar and 37∘C ? Air consists of 1% Ar atoms. Assuming that the argon atoms from the last breath of Plato have been distributed randomly throughout the atmosphere (5×1018m3) , how long would it take to breathe one of these atoms? A typical adult breath rate is 10min−1
View Solution
Knowledge Check
2 cm of rain has fallen on a square km of land. Assuming that 50% of the raindrops could have been collected and contaied in a pool having a 100m×10m base, by what level would the water level in the pool have increased ?
A1kmB10mC10cmD1mA projectile is projected at an angles of 37∘ to the horizontal. Had it been projected at 53∘, its time of flight on level ground would have been 1 sec more. At what angles should it be projected so that its range is 10 m less than either of the situations ?
A26.5∘B30∘C18∘D15∘In the figure, the cross-sectional area of the smaller tube is a and that of the larger tube is 2a. A block of mass m is kept in the smaller tube having the base area that of the tube. The difference between water levels of the two tubes is
 AP0ρg+maρBP0ρg+m2aρCmaρDm2ρa
AP0ρg+maρBP0ρg+m2aρCmaρDm2ρa
A housing society used to collect rain water from the foof of its building 22m×20m to a cylindical vessel having diameter of base 2 m and height 3.5 m and them poump this water into the main water tank so that all members can use it. On a particular day the rain water collected from the roof just filled the cylindrical vessel. Then, find the rain water collected from the roof just filled the cylindrical vessel. Then, find the rainfall in centimetre.
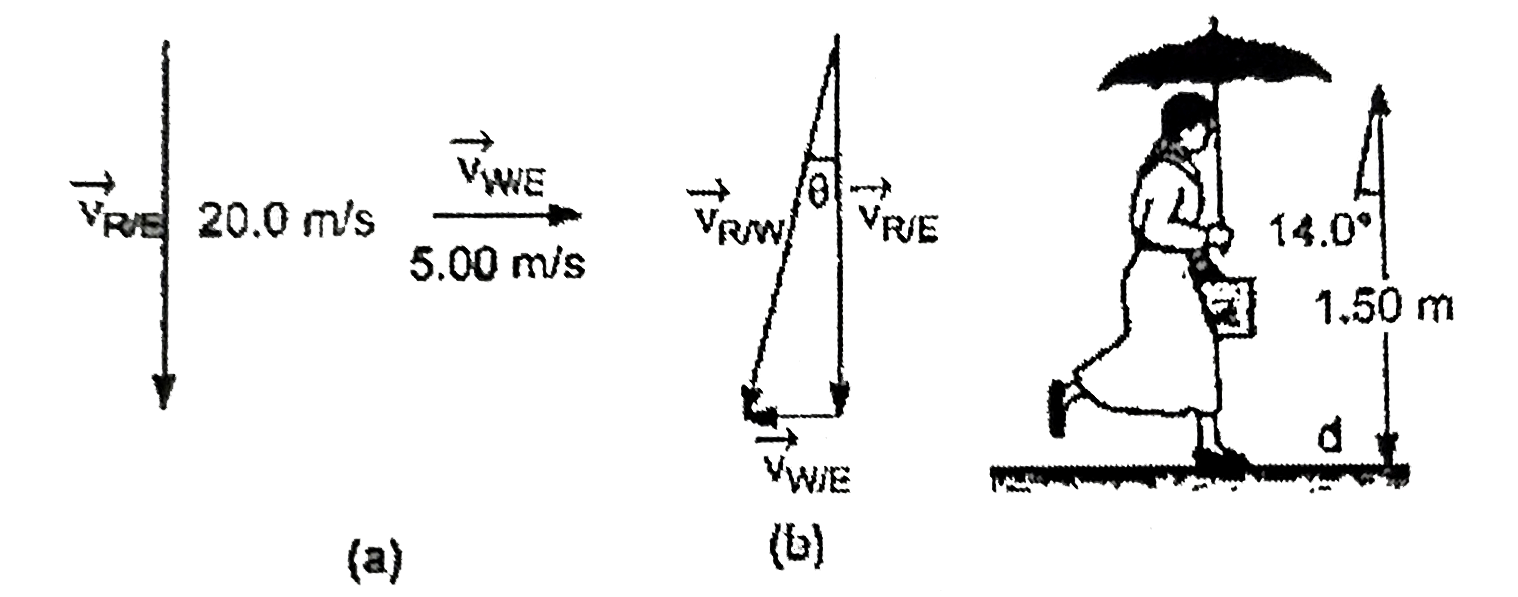
View SolutionA woman is running through rain at a speed of 5.00 m/s. Rain is falling vertically at a speed of 20.0 m/s. (a) What is the velocity of the rain relative to the woman? (b) How far in front of her would an umbrella have to extend to keep the rain off if she holds the umbrella 1.50 m above her feet?
View SolutionCalculate the excess pressure withing a bubble of air of radius 0.1 mm in water. If te bubble had been formed 10 cm below the water surface on a day when the atmospheric pressure was 1.013×103Pa then what would have been the total pressure inside the bubble? surface tension of water =73×10−3N/m
View SolutionA plane is in level flight at constant speed and each of the two wings has an area of 25m2. If the speed of the air on the upper and lower surfaces of the wing are 270kmh−1 and 234kmh−1 respectively, then the mass of the plane is (take the density of the air = 1kgm−3)
View SolutionOn a rainy day, 60 cm of rain is recorded in a region. What is the volume of water collected in an open and empty rectangular water tank that measures 12 m (length) ×10m (width) and 50 cm (depth) ?
View Solution
