A longitudinal wave is travelling along a spiral spring with a velocity of 2 mis. If the time period of the wave is 0.2s, fmd the wave length.
To find the wavelength of a longitudinal wave traveling along a spiral spring, we can follow these steps:
1. Identify the Given Information:
- Velocity (v) of the wave = 2 m/s
- Time period (T) of the wave = 0.2 s
2. Calculate the Frequency (f):
- The frequency can be calculated using the formula:
f=1T
- Substituting the value of the time period:
f=10.2=5 Hz
3. Use the Wave Equation to Find Wavelength (λ):
- The relationship between velocity (v), frequency (f), and wavelength (λ) is given by the equation:
v=f×λ
- Rearranging the formula to solve for wavelength (λ):
λ=vf
4. Substitute the Values:
- Now substitute the values of velocity and frequency into the equation:
λ=2 m/s5 Hz=0.4 m
5. Final Answer:
- The wavelength (λ) of the wave is 0.4 meters.
- Velocity (v) of the wave = 2 m/s
- Time period (T) of the wave = 0.2 s
- The frequency can be calculated using the formula:
f=1T
- Substituting the value of the time period:
f=10.2=5 Hz
- The relationship between velocity (v), frequency (f), and wavelength (λ) is given by the equation:
v=f×λ
- Rearranging the formula to solve for wavelength (λ):
λ=vf
- Now substitute the values of velocity and frequency into the equation:
λ=2 m/s5 Hz=0.4 m
- The wavelength (λ) of the wave is 0.4 meters.
Similar Questions
A wave travels along the positive x-direction with a speed of 20ms−1. The amplitude of the wave is 0.20 cm and the wavelength 2.0 cm. (a) Write a suitable wave equation which describes this wave. (b) What is the displacement and velocity of the particle at x = 2.0 cm at time t = 0 according to the wave equation written ? Can you get different values of this quantity if the wave equation is written in a different fashion ?
View SolutionA wave of time period 10 ms travels with a velocity of 1.5ms−1. If the time period of another wave is 6 ms, find its velocity and also find the ratio of their velocityies. (Assume that the wavelength of the two waves is same)
View Solution
Knowledge Check
Longitudinal waves cannot travel through
AvacuumBsolidsCliquidsDgasesA source of longitudinal waves vibrates 320 times in two seconds. If the velocity of this wave in the air is 240ms−1 , find the wavelength of the wave .
A1 mB1.5 mC2 mD2.5 mA progressive wave is travelling along the positive direction of the x-axis. For this wave, amplitude = 5 cm, period = 0.2 s and wavelength = 30 cm
The equation of the wave using SI units is given byAy=0.05sin2π(t0.2−x0.3)By=0.5sin2π(t0.2−x30)Cy=5sin2π(t0.2+x3)Dy=0.05sin2π(x0.3+t0.2)
A wave of time period 10 ms travels with a velocity of 1.5ms−1. If the time period of another wave is 6 ms, find its velocity and also find the ratio of their velocityies. (Assume that the wavelength of the two waves is same)
View SolutionIf 4 waves are produced in 2s, then the time period of the wave is _______
View SolutionThe longitudinal waves travel in a coiled spring at a rate of 4m/s. The distance between two consecutive compressions in 20cm. Find:
(i) Wavelength of the wave (ii) Frequency of the wave
View SolutionA wave represented by the given equationY=Asin(10πx+15πt+π3) , where x is in meter and t is in second. The expression represents
(1) A wave travelling in the negative X direction with a velocity of 1.5 m/sec
(2) A wave travelling in the negative X direction with a wavelength of 0.2 m
(3) A wave travelling in the positive X direction with a velocity of 1.5 m/sec.
(4) A wave travelling in the positive X direction with a wavelength of 0.2 m
View SolutionThe graph shows a wave at t=0 travelling to the right with a velocity of 4m/s. The equation that best represents the wave is
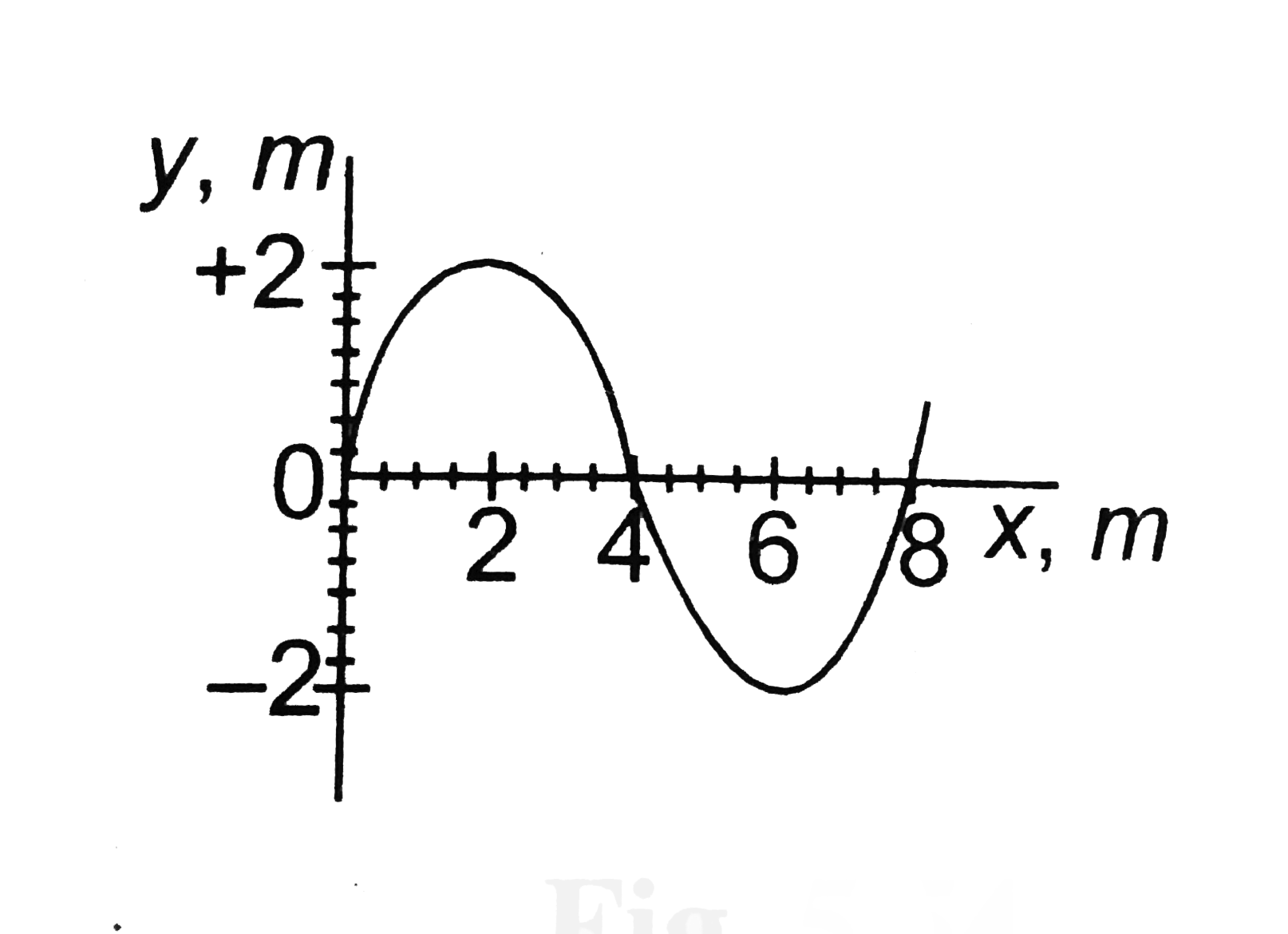
View Solution
