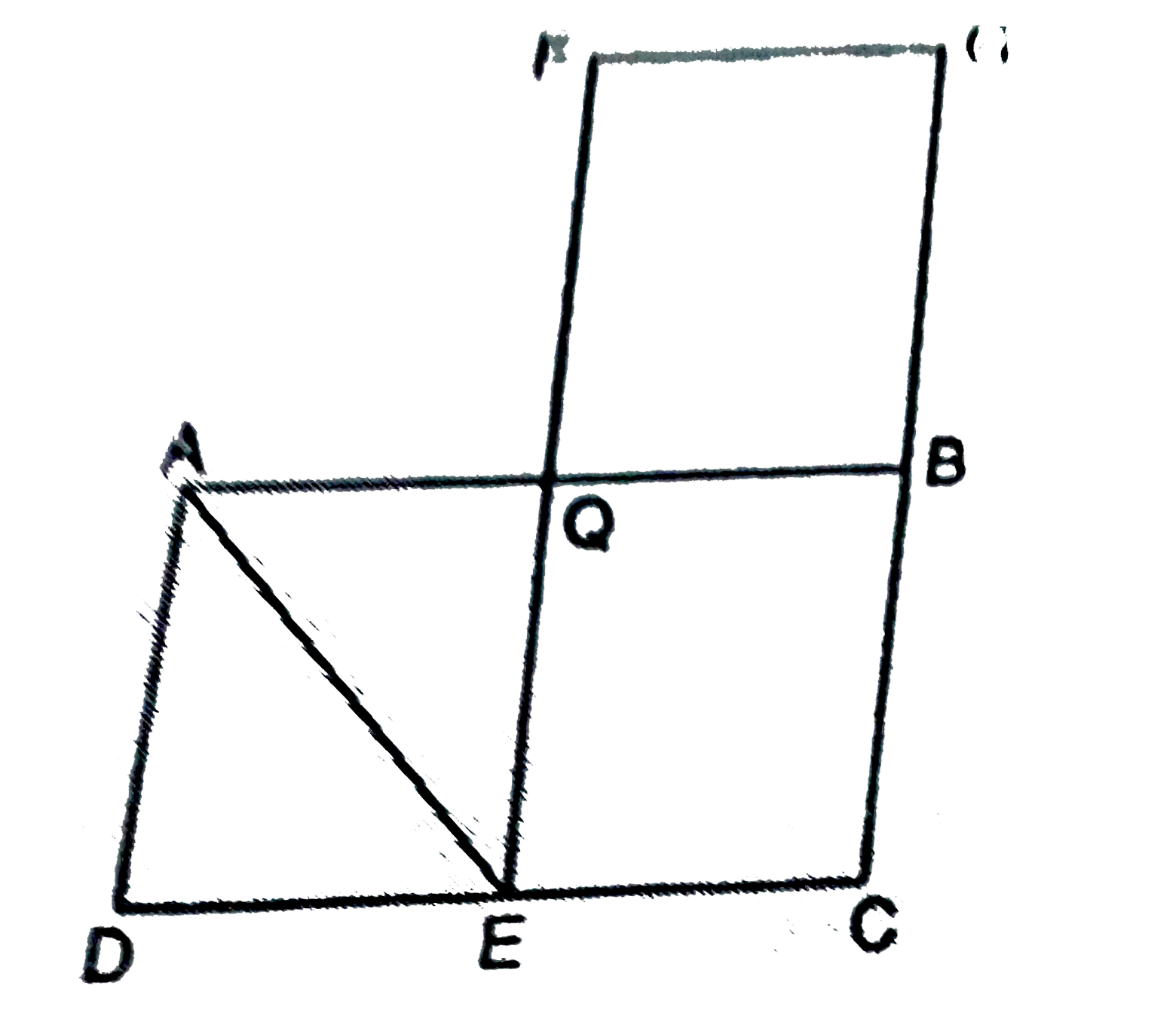
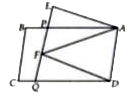
In the given figure, ABCD and ABFE are parallelograms such that ar(quad. EABC) =19 cm2 and ar(||gm ABCD) = 28 cm2. Then, ar(△BCF) is 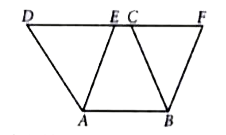
4cm2
4.5cm2
9cm2
8cm2
The correct Answer is:C
Step by step video & image solution for In the given figure, ABCD and ABFE are parallelograms such that ar(quad. EABC) =19 cm""^2 and ar(||gm ABCD) "= 28 cm"^2. Then, ar(triangleBCF) is by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- FOOTSTEPS TOWARDS (JEE MAIN)BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS (JEE MAIN)EXERCISE - Section B (Numerical Value Type Questions)
10 Videos
- COORDINATE GEOMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - COORDINATE GEOMETRYEXERCISE - Olympiad/HOTS Corner
15 Videos
- FOOTSTEPS TOWARDS CBSE BOARDBOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS CBSE BOARDEXERCISE - PART-B (SECTION-IV)
4 Videos
Similar Questions
Knowledge Check
In the given figure, ABCD and ABEF are parallelograms such that ar(quad. EABC) 17cm2 and ar(||gm ABCD) =25cm2. Then ar(△BCF) = ?
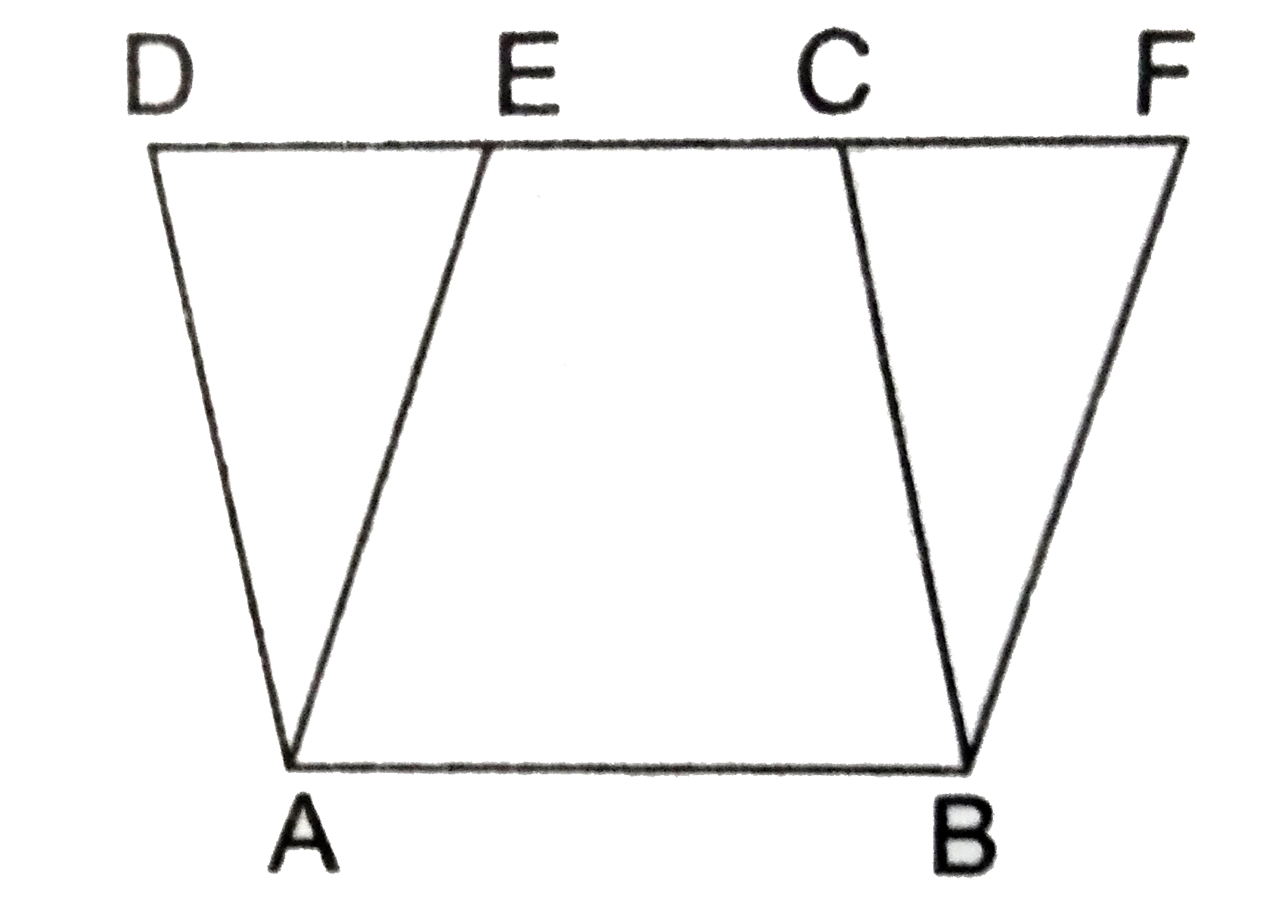 A4cm2B4.8cm2C6cm2D8cm2
A4cm2B4.8cm2C6cm2D8cm2In the given figure, ABCD and AEFD a re two parallelograms.
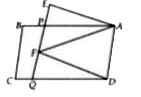
ar(ΔPEA) =Aar(∣∣gmΔPEA)Bar(ΔPFD)Car(ΔQFD)Dar(∣∣gmΔCQPB)In the given figure, ABCD and AEFD a re two parallelograms.
(ar(ΔAPE))/(ar(ΔPFA)) =A(ar(ΔQFD))/(ar(ΔPFD))B(ar(ΔAEF))/(ar(ΔPFD))C(ar(ΔQFD))/(ar(ΔAEF))DNone of these
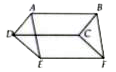
In the figure, ABCD, DCFE and ABFE are parallelograms. Show that ar(ΔADE) = ar(ΔBCF).
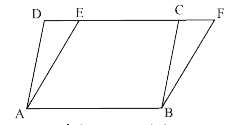
View SolutionIn the figure ABCD and ABFE are Parallelograms then find ar (ΔBCF).
If ar (ABCE)=18cm2
ar (ABCD)=25cm2
View SolutionIn figure, ABCD and AEFD are two parallelograms. Prove that ar(ΔPEA)=ar(ΔQFD).
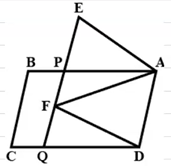
View SolutionIn figure, ABCD and AEFD are two parallelograms. Prove that ar(ΔPEA)=ar(ΔQFD).
View SolutionIn the given figure, ABCD is a ||gm in which DL⊥AB. IF AB = 10 cm and DL = 4 cm then the ar(||gm ABCD) = ?
View Solution