Which of the following figures satisfy the given conditions ?
Figure (i) Faces : 4, Edges : 6, Vertices : 4
Figure (ii) Faces : 5, Edges : 9, Vertices : 6




The correct Answer is:C
To solve the question, we need to analyze the two figures based on the given conditions regarding their faces, edges, and vertices.
1. Understanding the First Figure:
- We need to check if there exists a 3D shape with 4 faces, 6 edges, and 4 vertices.
- A common shape that fits this description is a triangular pyramid (also known as a tetrahedron).
- A triangular pyramid has:
- Faces: 4 (1 triangular base + 3 triangular sides)
- Edges: 6 (3 edges of the base + 3 edges connecting the apex to each vertex of the base)
- Vertices: 4 (1 apex + 3 vertices of the base)
Therefore, the first figure satisfies the conditions.
2. Understanding the Second Figure:
- Now, we need to check if there exists a 3D shape with 5 faces, 9 edges, and 6 vertices.
- A shape that fits this description is a triangular prism.
- A triangular prism has:
- Faces: 5 (2 triangular bases + 3 rectangular lateral faces)
- Edges: 9 (3 edges of one triangle + 3 edges of the other triangle + 3 edges connecting the two triangles)
- Vertices: 6 (3 vertices from one triangle + 3 vertices from the other triangle)
Therefore, the second figure also satisfies the conditions.
Conclusion:
Both figures satisfy the given conditions:
- Figure (i): Triangular Pyramid (4 faces, 6 edges, 4 vertices)
- Figure (ii): Triangular Prism (5 faces, 9 edges, 6 vertices)
- We need to check if there exists a 3D shape with 4 faces, 6 edges, and 4 vertices.
- A common shape that fits this description is a triangular pyramid (also known as a tetrahedron).
- A triangular pyramid has:
- Faces: 4 (1 triangular base + 3 triangular sides)
- Edges: 6 (3 edges of the base + 3 edges connecting the apex to each vertex of the base)
- Vertices: 4 (1 apex + 3 vertices of the base)
- Now, we need to check if there exists a 3D shape with 5 faces, 9 edges, and 6 vertices.
- A shape that fits this description is a triangular prism.
- A triangular prism has:
- Faces: 5 (2 triangular bases + 3 rectangular lateral faces)
- Edges: 9 (3 edges of one triangle + 3 edges of the other triangle + 3 edges connecting the two triangles)
- Vertices: 6 (3 vertices from one triangle + 3 vertices from the other triangle)
Both figures satisfy the given conditions:
- Figure (i): Triangular Pyramid (4 faces, 6 edges, 4 vertices)
- Figure (ii): Triangular Prism (5 faces, 9 edges, 6 vertices)
Topper's Solved these Questions
- UNDERSTANDING ELEMENTARY SHAPES Book:MTG IIT JEE FOUNDATIONChapter:UNDERSTANDING ELEMENTARY SHAPES Exercise:EXERCISE - SUBJECTIVE PROBLEMS (INTEGER/NUMERICAL VALUE TYPE)
Explore 10 Videos
- SYMMETRYBook:MTG IIT JEE FOUNDATIONChapter:SYMMETRYExercise:Olympiad/HOTS Corner
Explore 13 Videos
- WHOLE NUMBERS Book:MTG IIT JEE FOUNDATIONChapter:WHOLE NUMBERS Exercise:OLYMPIAD/HOTS CORNER (FILL IN THE BLANKS)
Explore 5 Videos
Similar Questions
Knowledge Check
Which of the following is the adjacent vertices of the given figure?
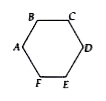 AA and CBB and ECD and E,DF and D
AA and CBB and ECD and E,DF and DWhich three-dimensional figure has 5 faces, 8 edges and 5 vertices ?
A B
B C
C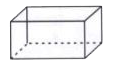 D
D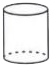
Can a polyhedron have 10 faces, 20 edges and 15 vertices? Check using Euler's formula.
AYesBNoCCannot be determinedDNone of the above
A polyhedron can have 10 faces, 20 edges and 15 vertices.
View SolutionFind the equation of the ellipse that satisfies the given conditions: Vertices (±6,0), foci (±4,0)
View SolutionFind the equation for the ellipse that satisfies the given conditions:Vertices (±6,0), foci (±4,0)
View SolutionIn each of the following find the equations of the hyperbola satisfying the given condition: vertices (0,±6), e=53
View SolutionFind the coordinates of the vertices of the given figures.
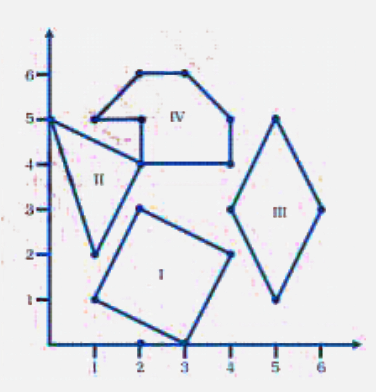
View Solution