A book of 1,456 pages has 9,95,904 words in it. The number of words in each page is ____.
584
684
675
595
To find the number of words in each page of the book, we need to divide the total number of words by the total number of pages. Here’s a step-by-step solution:
Step 1: Identify the total number of words and pages
- Total words = 9,95,904
- Total pages = 1,456
Step 2: Set up the division
We need to calculate the number of words per page by dividing the total number of words by the total number of pages:
Words per page=Total wordsTotal pages=9,95,9041,456
Step 3: Perform the division
Now, we will divide 9,95,904 by 1,456.
1. Divide 9,95,904 by 1,456:
- 1,456 goes into 9,95,904.
- First, we see how many times 1,456 fits into the first part of 9,95,904.
- 1,456 fits into 9,95,904 approximately 684 times.
2. Calculate the product:
- Multiply 1,456 by 684 to check:
1,456×684=9,95,904
3. Subtract to find the remainder:
- After multiplying, we subtract:
9,95,904−9,95,904=0
- This means there is no remainder.
Step 4: Conclusion
The number of words in each page is:
Words per page=684
Thus, the answer is 684 words per page.
---
- Total words = 9,95,904
- Total pages = 1,456
We need to calculate the number of words per page by dividing the total number of words by the total number of pages:
Words per page=Total wordsTotal pages=9,95,9041,456
Now, we will divide 9,95,904 by 1,456.
- 1,456 goes into 9,95,904.
- First, we see how many times 1,456 fits into the first part of 9,95,904.
- 1,456 fits into 9,95,904 approximately 684 times.
- Multiply 1,456 by 684 to check:
1,456×684=9,95,904
- After multiplying, we subtract:
9,95,904−9,95,904=0
- This means there is no remainder.
The number of words in each page is:
Words per page=684
Topper's Solved these Questions
- RATIO AND PROPORTIONBOOK - MTG IIT JEE FOUNDATIONCHAPTER - RATIO AND PROPORTIONEXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS)(LEVEL - 2)
14 Videos
- RATIO AND PROPORTIONBOOK - MTG IIT JEE FOUNDATIONCHAPTER - RATIO AND PROPORTIONEXERCISE - EXERCISE (MATCHING THE FOLLOWING)
3 Videos
- RATIO AND PROPORTIONBOOK - MTG IIT JEE FOUNDATIONCHAPTER - RATIO AND PROPORTIONEXERCISE - NCERT SECTION (EXERCISE 12.3)
12 Videos
- PREP TEST -1BOOK - MTG IIT JEE FOUNDATIONCHAPTER - PREP TEST -1EXERCISE - SECTION-B
35 Videos
- SYMMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SYMMETRYEXERCISE - Olympiad/HOTS Corner
13 Videos
Similar Questions
In a book with page numbers from 1 to 100, sme pages are torn off. The sum of the numbers on the remaining pages is 4949. How many pages are torn off ?
View SolutionA 60-page book has n lines per page. If the number of lines were reduced by 3 in each page, the number of pages would have to be increased by 10 to give the same writing space. What is the value of n ?
View Solution
Knowledge Check
From a book of 20 pages, one page has been disappeared. The sum of rest pages numbers is 195. Find numbers on both sides of left page.
A9, 10B5,6C11,12D7,8A page in a book can have at most 300 words. X = Number of misprints on a page.
Then the random variable isAnot discreteBnot continuousCdiscreteDcontinuousIf the cost of printing a book of 320 leaves with 21 lines on each page and on an average 11 words in each line is 19 , find the cost of printing a book with 297 leaves, 28 lines on each page and 10 words in each line.
A'2238B'2038C'2138D'2134
Directions: The following bar graph shows the number of pages in five different books (viz. A, B, C, D and E). Study the graph carefully and answer the following questions.
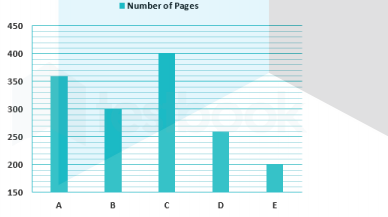
In book B, every even number page contains 75 words and the odd number pages contain 60 words. A person read the first 43 pages and stop reading and, on another day, read the last 216 pages. Find how many words remain unread.
View SolutionIn the first proof reading of a book containing 300 pages the following distribution of misprints was obtained: No. of misprints per page (x) : 0 1 2 3 4 5 No. of pages (f) : 154 95 36 9 5 1 Find the average number of misprints per page.
View SolutionA book with 480 pages is to have its pages numbered in the usual fashion. How many digits will this need?
View SolutionIn word you can force a page break_______.
View SolutionIn word you can force a page break_______.
View Solution
