Name the quadrilaterals which have both line and rotational symmetry of order more than 1.
To solve the question of naming the quadrilaterals that have both line and rotational symmetry of order more than 1, we can follow these steps:
Step 1: Understand the Definitions
- Line Symmetry: A shape has line symmetry if it can be divided into two identical halves by a line (the line of symmetry).
- Rotational Symmetry: A shape has rotational symmetry if it can be rotated (less than a full circle) about a central point and still look the same. The order of rotational symmetry indicates how many times it matches its original shape during a full 360-degree rotation.
Step 2: Identify Quadrilaterals with Both Symmetries
1. Square:
- Line Symmetry: A square has 4 lines of symmetry (two diagonals and two midlines).
- Rotational Symmetry: A square has rotational symmetry of order 4 (it looks the same after a rotation of 90°, 180°, 270°, and 360°).
2. Rhombus:
- Line Symmetry: A rhombus has 2 lines of symmetry (the diagonals).
- Rotational Symmetry: A rhombus has rotational symmetry of order 2 (it looks the same after a rotation of 180° and 360°).
3. Rectangle:
- Line Symmetry: A rectangle has 2 lines of symmetry (the midlines).
- Rotational Symmetry: A rectangle has rotational symmetry of order 2 (it looks the same after a rotation of 180° and 360°).
4. Kite:
- Line Symmetry: A kite has 1 line of symmetry (the line through the unequal angles).
- Rotational Symmetry: A kite does not have rotational symmetry of order more than 1.
Step 3: List the Quadrilaterals
Based on the analysis, the quadrilaterals that have both line and rotational symmetry of order more than 1 are:
- Square
- Rhombus
- Rectangle
Conclusion
The quadrilaterals that have both line and rotational symmetry of order more than 1 are:
1. Square
2. Rhombus
3. Rectangle
- Line Symmetry: A shape has line symmetry if it can be divided into two identical halves by a line (the line of symmetry).
- Rotational Symmetry: A shape has rotational symmetry if it can be rotated (less than a full circle) about a central point and still look the same. The order of rotational symmetry indicates how many times it matches its original shape during a full 360-degree rotation.
1. Square:
- Line Symmetry: A square has 4 lines of symmetry (two diagonals and two midlines).
- Rotational Symmetry: A square has rotational symmetry of order 4 (it looks the same after a rotation of 90°, 180°, 270°, and 360°).
- Line Symmetry: A rhombus has 2 lines of symmetry (the diagonals).
- Rotational Symmetry: A rhombus has rotational symmetry of order 2 (it looks the same after a rotation of 180° and 360°).
- Line Symmetry: A rectangle has 2 lines of symmetry (the midlines).
- Rotational Symmetry: A rectangle has rotational symmetry of order 2 (it looks the same after a rotation of 180° and 360°).
- Line Symmetry: A kite has 1 line of symmetry (the line through the unequal angles).
- Rotational Symmetry: A kite does not have rotational symmetry of order more than 1.
Based on the analysis, the quadrilaterals that have both line and rotational symmetry of order more than 1 are:
- Square
- Rhombus
- Rectangle
The quadrilaterals that have both line and rotational symmetry of order more than 1 are:
1. Square
2. Rhombus
3. Rectangle
Topper's Solved these Questions
- SYMMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SYMMETRYEXERCISE - Exercise (Multiple Choice Questions)
25 Videos
- SYMMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SYMMETRYEXERCISE - Exercise (Match the following)
1 Video
- SYMMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SYMMETRYEXERCISE - NCERT SECTION (Exercise -13.2)
1 Video
- SIMPLE EQUATIONSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SIMPLE EQUATIONSEXERCISE - Olympiad /Hots Corner
18 Videos
- THE TRIANGLE AND ITS PROPERTIES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - THE TRIANGLE AND ITS PROPERTIES EXERCISE - Olympiad/Hots Corner
20 Videos
Similar Questions
Name the quadrilateral which has both line and rotational symmetry of order more than 2.
View SolutionDraw, wherever possible, a rough sketch of:
(i) a triangle with both line and rotational symmetries of order more than 1.
(ii) a triangle with only line symmetry and no rotational symmetry of order more than 1.
(iii) a quadrilateral with a rotational symmetry of order more than 1 but not a line symmetry.
(iv) a quadrilateral with line symmetry but not a rotational symmetry of order more than 1.
View Solution
Knowledge Check
Which of the following have both line symmetry and rotational symmetry of order more than 1?
AABHCCDZA square has a rotational symmetry of order
A1B2C3D4
The given figure has rotational symmetry of order
View SolutionDoes the fig have rotational symmetry?

View SolutionDraw a rough sketch of a quadrilateral with a rotational symmetry of order more than 1 but no line of symmetry.
View SolutionWhich of the following figures have rotational symmetry of order more than 1?
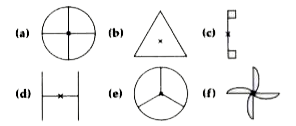
View SolutionWhich of the following figures have rotational symmetry of order more than 1:
View SolutionDefine order of rotational symmetry.
View Solution
