Which of the following is(are) correct for H atom?
(i) 1s<2s<2p<3s<3p
(ii) 1s<2s=2p<3s=3p
(iii) 1s<2p<3d<4s
(iv) 1s<2s<4s<3d
The correct choice is
(ii), (iii)
(i), (iv)
(i), (iii)
(ii), (iv)
The correct Answer is:B
(i), (iv)
Topper's Solved these Questions
- BOOK - CENGAGE CHEMISTRYCHAPTER - ATOMIC STRUCTUREEXERCISE - CHALLENGING EXERCISE3videos
ATOMIC STRUCTURE
- BOOK - CENGAGE CHEMISTRYCHAPTER - ACIDS, BASES AND SALTSEXERCISE - Olympiad And NTSE Level exercises10videos
ACIDS, BASES AND SALTS
- BOOK - CENGAGE CHEMISTRYCHAPTER - CHEMICAL ARITHMETIC EXERCISE - MANDATORY EXERCISE (EXERCISE SET III) (OLYMPIAD AND NTSE LEVEL EXCERCISES)10videos
CHEMICAL ARITHMETIC
Similar Questions
Knowledge Check
- Question 1 - Select One
If s(5x−4)−2<2x−1<4x−2, then the value of x is
A1B0C−1D2 - Question 2 - Select One
Four elements P,Q,R & S have ground state electronic configuration as:
P→1s22s22p63s23p3Q→1s22s22p63s23p1R→1s22s22p63s23p63d104s24p3S→1s22s22p63s23p63d104s24p1
Comment which of the following option represent the correct order of true (T) & false (F) statement I size of P< size of Q II size of R< size of S
III size of P< size of R (appreciable difference) IV size of Q< size of S (appreciable difference)ATTTTBTTTFCFFTTDTTFF - Question 3 - Select One
Which of the following statements (s) is/are TRUE ?
I. 23√5<32√5<54√3
II. 32√5<23√3<74√5AOnly IBOnly IICBoth I and IIDNeither I nor II
The order of energy of orbitals is s<p<d<f
View SolutionThe order of energy of orbitals is s<p<d<f
View SolutionRecord the following sets of compounds according to increasing pKa(=−logKa)
(a).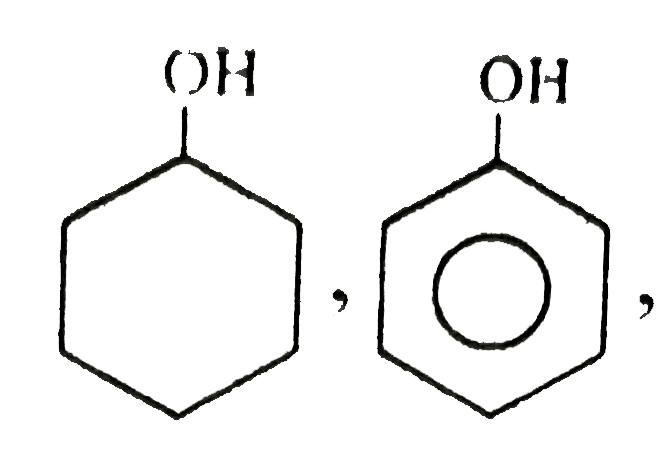
cyclohexane carboxylic acid
(A). 3<2<1
(B). 3>2>1
(C). 1<2<3
(D). 3<1<2
(b). 1-butyne, 1-butene, butane
(A). 3<2<1
(B). 3<2<1
(C). 1<3<2
(D). 3<2>1
(c). Propanoic acid, 3-bromopropanoic acid, 2-nitropropanoic acid
(A). 3lt2lt1
(B). 3gt2gt1
(C). 1lt2lt3
(D). 3lt1lt2View SolutionA line l passing through the origin is perpendicular to the lines
1:(3+t)ˆi+(−1+2t)ˆj+(4+2t)ˆk−∞<t<∞and1_(2):(3+2s)ˆi+(3+2s)ˆi+(3+2s)ˆj+(2+s)ˆk,−∞<s<∞
Then the coordinate(s) of the point(s) on 12 at a distance of √17 from the point of intersection of 1 and 11 is (are)View SolutionA line l passing through the origin is perpendicular to the lines
1:(3+t)ˆi+(−1+2t)ˆj+(4+2t)ˆk−∞<t<∞and1_(2):(3+2s)ˆi+(3+2s)ˆi+(3+2s)ˆj+(2+s)ˆk,−∞<s<∞
Then the coordinate(s) of the point(s) on 12 at a distance of √17 from the point of intersection of 1 and 11 is (are)View Solution