P and Q are the mid-points of the sides AB and BC, respectively, of $Δ A B C$ , right angled at B. Then:

$A Q^{2} + C P^{2} = A C^{2}$

$A Q^{2} + C P^{2} = \frac{4}{5} A C^{2}$

$A Q^{2} + C P^{2} = \frac{4}{3} A C^{2}$

$A Q^{2} + C P^{2} = \frac{5}{4} A C^{2}$

Text Solution

Verified by Experts

The correct Answer is:D

Updated on:21/07/2023

L and M are the mid points of AB and BC respectively of $Δ A B C$ , right angled at B . $4 L C^{2} =$

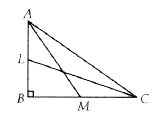A $A B^{2} + 4 B C^{2}$
B $B C^{2} + 4 A B^{2}$
C $A C^{2} + 4 A B^{2}$
DNone of these
$P$ and $Q$ are points on the sides $C A$ and $C B$ respectively of $A B C$ , right angled at $C$ . Prove that $A Q^{2} + B P^{2} = A B^{2} + P Q^{2}$ .
A $B C^{2} + P Q^{2}$
B $A B^{2} + P C^{2}$
C $A B^{2} + P Q^{2}$
D $B C^{2} + A C^{2}$
If P, Q, R are the mid -points of the sides AB, BC, CA respectively of a $Δ A B C and \to a . \to p \to q$ are the position vectors of A.P.Q respectively, then what is the position vector of R?
AA. $2 \to a - (\to p - \to q)$
BB. $(\to p - \to q) - 2 \to a$
CC. $\to a - (\to p - \to q)$
DD. $\frac{\to a}{2} - \frac{(\to p - \to q)}{2}$