The basic addition facts in the tens number system are given below :

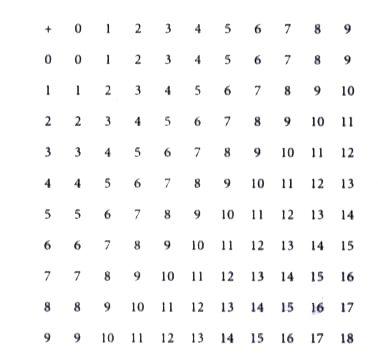
If the typical element is denoted by $a_{i j}$ , what are the following
$a_{1, 6}, a_{3, 7}, a_{5, 5}, a_{6, 1}, a_{10, 8}$

Text Solution

Verified by Experts

The correct Answer is: $a_{16} = 5, 8, 8, 5, 16$

If $a_{1}, a_{2}, a_{3}, \dots ., a_{9}$ are in GP, then what is the value of the following determinant?
$∣ ∣ ∣ ∣ ∣ \begin{matrix} {ln a}_{1}, {ln a}_{2}, {ln a}_{3} {ln a}_{4}, {ln a}_{5}, {ln a}_{6} {ln a}_{7}, {ln a}_{8}, {ln a}_{9} \end{matrix} ∣ ∣ ∣ ∣ ∣$
A0
B1
C2
D4
The number of integral solutions to the system of equations $a_{1} + a_{2} + a_{3} + a_{4} + a_{5} = 25$ and $a_{1} + a_{2} + a_{3}$ 10 are
A $2^{8} + 2^{5}$
B $2^{10} + 2^{5}$
C $2^{25} + 2^{10}$
DNone of these
If $A = ⎡ ⎢ ⎣ \begin{matrix} 1 & 1 & 0 2 & 1 & 5 1 & 2 & 1 \end{matrix} ⎤ ⎥ ⎦ and a_{i j}$ denote the cofactor of element $A_{i j}$ , then $a_{11} A_{21} + a_{21} A_{22} + a_{13} A_{23} =$
A $1$
B $0$
C $- 1$
D $2$