Consider triangle ABC as shown in the figure

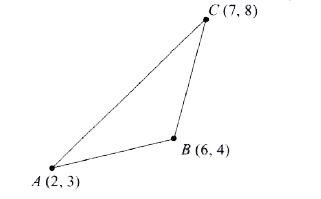
The new vertices of triangle when it undergoes the transformation $(\begin{matrix} X Y \end{matrix}) = ⎛ ⎝ \begin{matrix} \frac{- 1}{3} & 1 1 & \frac{- 1}{3} \end{matrix} ⎞ ⎠ (\begin{matrix} x y \end{matrix})$ will be

A

$A' \equiv (\frac{7}{3}, 1), B' \equiv (2, \frac{14}{3}), C' \equiv (\frac{17}{3}, \frac{13}{3})$

B

$A' \equiv (\frac{7}{3}, 1), B' \equiv (\frac{14}{3}, 2), C' \equiv (\frac{17}{3}, \frac{13}{3})$

C

$A' \equiv (\frac{7}{3}, 1), B' \equiv (2, \frac{14}{3}), C' \equiv (\frac{13}{3}, \frac{13}{3})$

D

$A' \equiv (1, \frac{7}{3}), B' \equiv (2, \frac{14}{3}), C' \equiv (\frac{17}{3}, \frac{13}{3})$

Text Solution

Verified by Experts

The correct Answer is:A

|

Updated on:21/07/2023

Class 10 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If all the vertices of a triangle undergo the same transfomation $(\begin{matrix} X Y \end{matrix}) = (\begin{matrix} \frac{1}{2} & \frac{- 1}{2} 1 & 1 \end{matrix}) (\begin{matrix} x y \end{matrix})$ what will be the effect on the area of the triangle ?
AIt will remain unchanged
BIt will become half
CIt will become 0
DIt will get double
Question 2 - Select One
Consider the $Δ A B C$ with vertices A( - 2 , 3) , B (2 , 1) and C (1 ,2)
What is the Centroid of the $Δ A B C$ ?
A $(\frac{1}{3}, 2)$
B $(2, 2)$
C $(- 2, 2)$
D $(2, - 2)$
Question 3 - Select One
Consider the $Δ A B C$ with vertices A( - 2 , 3) , B (3 , 1) and C (1 ,2)
What is the centroid of the $Δ A B C$ ?
A $(\frac{2}{3}, 2)$
B $(\frac{1}{3}, 2)$
C $(1, \frac{2}{3})$
D $(\frac{1}{2}, 3)$

CENGAGE-TRANSFORMATIONS-Test yourself (Level 1) M.C.Q

Home
Ask Doubt
Profile