Solve The equation
Statement -1 x2+(2m+1)x+(2n+1)=0 where m and n are integers, cannot have any rational roots.
Statement - 2 The quantity (2m+1)2−4(2n+1), where m,n∈I, can never be a perfect square.Solution in Punjabi
Statement -1 is true, Statement -2 is true, Statement -2 is a correct explanation for Statement-1
Statement -1 is true, Statement -2 is true, Statement -2 is not a correct explanation for Statement -1
Statement -1 is true, Statement -2 is false
Statement -1 is false, Statement -2 is true
The correct Answer is:A
We have x2+(2m+1)x+(2n+1)=0…….i
m,nεI
∴D=b2−4ac
=(2m+1)2−4(2n+1)
is never be a perfect square.
Therefore, the roots of Eq. (i) can never be integers. Hence the roots of Eq. (i) cannot have any rational as a=1,b,cεI. Hence both statement are true and statements-2 is a correct explanatioin of Statement -1.
Topper's Solved these Questions
- BOOK - ARIHANT MATHSCHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Subjective Type Questions)24videos
THEORY OF EQUATIONS
- BOOK - ARIHANT MATHSCHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)35videos
THEORY OF EQUATIONS
- BOOK - ARIHANT MATHSCHAPTER - THEORY OF EQUATIONSEXERCISE - MATCH TYPE2videos
THEORY OF EQUATIONS
- BOOK - ARIHANT MATHSCHAPTER - THE STRAIGHT LINESEXERCISE - The Straight Lines Exercise 8 : (Questions Asked in Previous 13 years Exams)1videos
THE STRAIGHT LINES
- BOOK - ARIHANT MATHSCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Three Dimensional Coordinate System Exercise 12 : Question Asked in Previous Years Exam2videos
THREE DIMENSIONAL COORDINATE SYSTEM
Similar Questions
For what values of m the equation (1+m)x2−2(1+3m)x+(1+8m)=0 has (m∈R)
(i) both roots are equal03:24View SolutionIn the quadratic equation ax2+bx+c=0. if δ=b2−4ac and α+β,α2+β2,α3+β3 are in G.P. and α,β are the roots of ax2+bx+c=0
02:59View Solution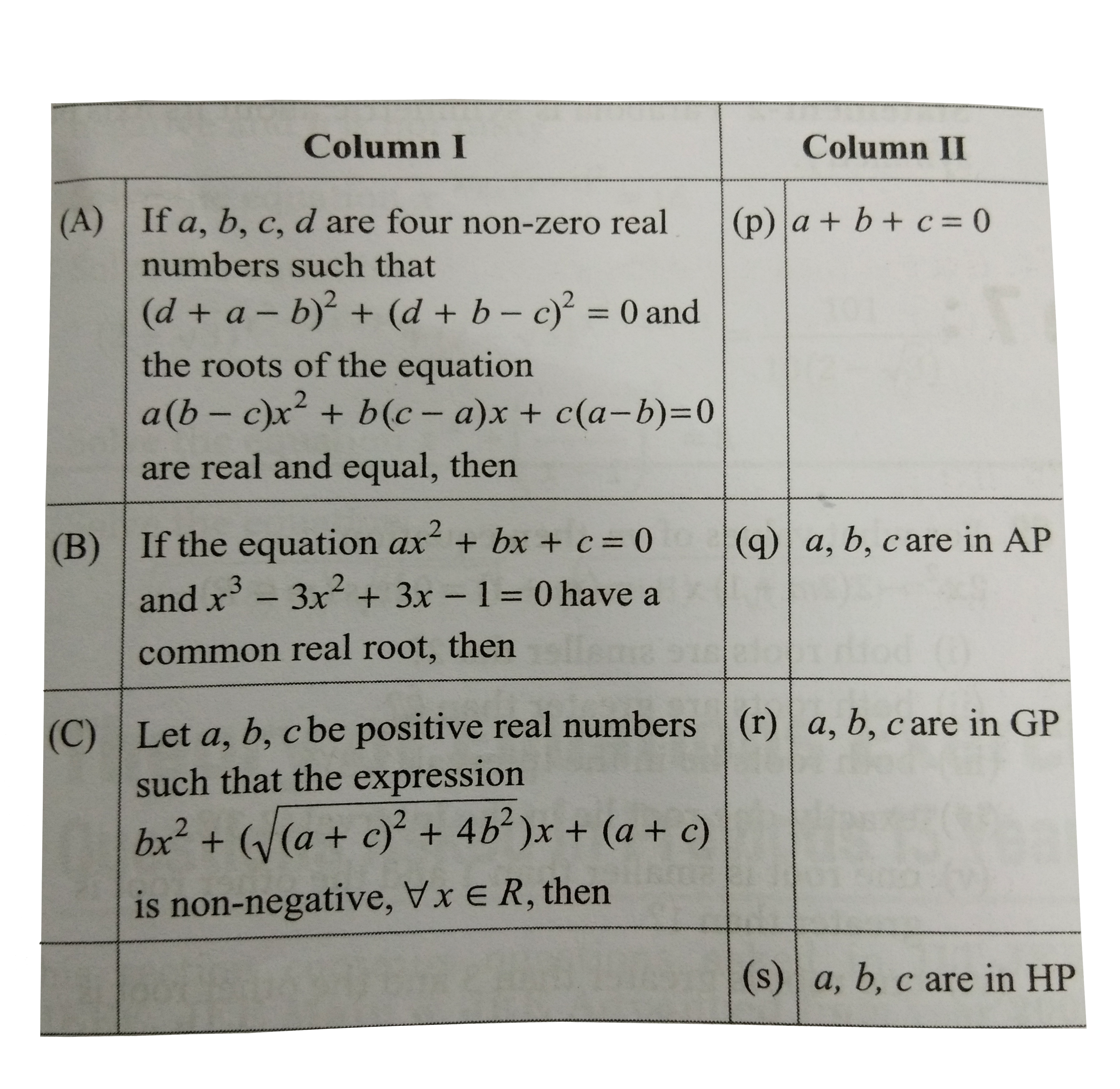
05:09View SolutionSolve the following system of inequalities graphically
x+5y≤25,2x+2y≥2,2x−2y≤0,x≥0,y≥003:49View SolutionConsider the line L1:x−12=y−1=z+31,L2:x−41=y+31=z+32 find the angle between them.
01:17View SolutionThe integral value of m for which the root of the equation mx2+(2m−1)x+(m−2)=0 are rational are given by the expression [where n is integer]
(A)n2
(B) n(n+2)
(C) n(n+1)
(D) none of these03:00View SolutionIf (x-a)^(2m)(x-b)^(2n+1), where m and n are positive integers and a>b, is the derivative of a function f then-
03:07View SolutionIf 2m+n2m−n=16anda=2110 then (a2m+n−p)2(am−2n+2p)−1=
View SolutionIf Sn denotes the sum of first n terms of an A.P. <an> such that SmSn=m2n2, thenaman= a.2m+12n+1 b. 2m−12n−1 c. m−1n−1 d. m+1n+1
03:27View SolutionIf (1+i1−i)x=1 then (A) x=2n+1, where n is any positive ineger (B) x=4n, where n is any positive integer (C) x=2n where n is any positive integer (D) x=4n+1 where n is any positive integer
03:11View SolutionStatement -1 The equation
x2+(2m+1)x+(2n+1)=0 where m,nεI canot have any rational roots.
Statement -2 The quantity (2m+1)2−4(2n+1), where m,nεI can never be perfect square.04:12View SolutionSolve the equation sin2n−1x+2cos2n−1x=2,where n∈N.
03:16View SolutionIf f'(x)=(x−a)2n(x−b)2m+1, where m,n∈N, then :
02:09View SolutionThe integral value of m for which the root of the equation mx2+(2m−1)x+(m−2)=0 are rational are given by the expression [where n is integer]
(A)n2
(B) n(n+2)
(C) n(n+1)
(D) none of these02:48View SolutionIf 2m+n2m−n=16anda=2110 then (a2m+n−p)2(am−2n+2p)−1=
02:02View SolutionIf Sn denotes the sum of first n terms of an A.P. <an> such that SmSn=m2n2, thenaman= a.2m+12n+1 b. 2m−12n−1 c. m−1n−1 d. m+1n+1
04:10View Solution