ABCD is a convex quadrilateral and 3, 4, 5, and 6 points are marked on the sides AB, BC, CD, and DA, respectively. The number of triangles with vertices on different sides is Solution in Punjabi
270
220
282
None of these
The correct Answer is:D
Step by step video & image solution for ABCD is a convex quadrilateral and 3, 4, 5, and 6 points are marked on the sides AB, BC, CD, and DA, respectively. The number of triangles with vertices on different sides is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PERMUTATIONS AND COMBINATIONS BOOK - ARIHANT MATHSCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Exercise For Session 7
5 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - ARIHANT MATHSCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Exercise (Single Option Correct Type Questions)
30 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - ARIHANT MATHSCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Exercise For Session 5
18 Videos
- PARABOLABOOK - ARIHANT MATHSCHAPTER - PARABOLAEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
35 Videos
- PROBABILITYBOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
55 Videos
Similar Questions
The letters of the word 'DELHI' are arranged in all possible ways as in a dictionary, the rank of the word 'DELHI' is
View SolutionA lady gives a dinner party to 5 guests to be selected from nine friends. The number of ways of forming the party of 5, given that two of the friends will not attend the party together, is
View SolutionThere are 4 oranges, 5 apples and 6 mangoes in a fruit basket and all fruits of the same kind are identical. In how many ways can a person make a selection of fruits from among the fruits in the basket?
View SolutionLet A and B be two finite sets such that n(A) =85, n(B) = 65and n(A ∪ B) = 135, find n(A ∩ B)?
View Solutionfind the derivative of sin x cos x
View SolutionABCD is a rhombus and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
View SolutionABCD is a quadrilateral; P,Q,RandS are the points of trisection of side AB,BC,CDandDA respectively and are adjacent to AandC ; prove that PQRS is parallelogram.
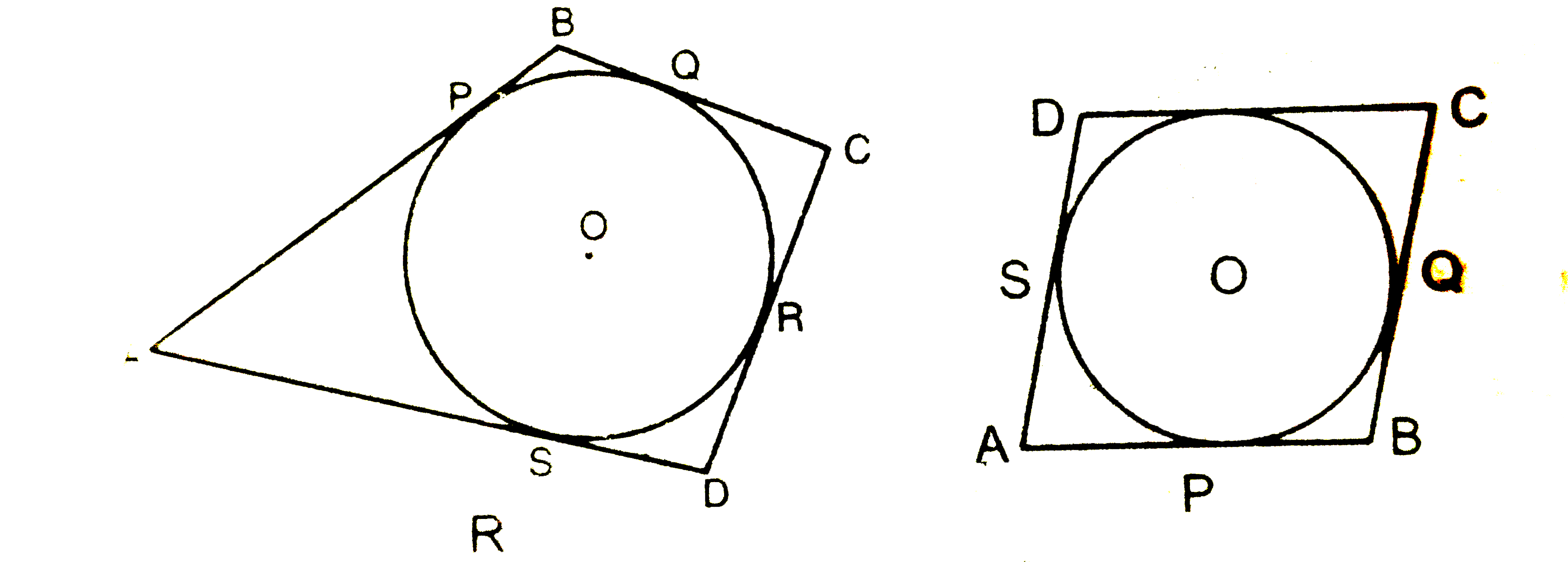
View SolutionIn Fig.2, a quadrilateral ABCD is drawn to circumscribe a circle, with centre O, in such a way that the sides AB, BC, CD and DA touch the circle at the points P, Q, R and S respectively. Prove that AB + CD = BC + DA.
View SolutionP, Q , R and S are respectively the mid-points of the sides AB, BC, CD and DA of a quadrilateral ABCD in which AC = BD. Prove that PQRS is a rhombus.
View Solutionएक वृत्त, एक चतुर्भुज ABCD की चारों भुजाओं को अन्तः स्पर्श करता है। सिद्ध कीजिए कि-
AB + CD = AD + BC.
(अथवा) आकृति, में एक चतुर्भुज ABCD, O केन्द्र वाले वृत्त के परिगत इस प्रकार बनाई गई है कि भुजाएँ AB, BC, CD, तथा DA वृत्त को क्रमशः बिन्दुओं P, Q, R तथा S पर स्पर्श करती हैं। सिद्ध कीजिए कि
AB + CD = BC + DA
View SolutionIf ABCD is a cyclic quadrilateral with AB = 6 , BC = 4, CD = 5, DA= 3 and ∠ABC=θ, then cos θ =
View SolutionABCD চতুর্ভুজের AB, BC, CD এবং DA বাহুগুলির ওপর 3, 4, 5 এবং 6 টি বিন্দু আছে। ওই বিন্দুগুলিকে শীর্ষবিন্দু ধরে গঠিত ত্রিভুজের সংখ্যা নির্ণয় করো।
View SolutionP, Q , R and S are respectively the mid-points of the sides AB, BC, CD and DA of a quadrilateral ABCD in which AC = BD. Prove that PQRS is a rhombus.
View SolutionIn Fig.2, a quadrilateral ABCD is drawn to circumscribe a circle, with centre O, in such a way that the sides AB, BC, CD and DA touch the circle at the points P, Q, R and S respectively. Prove that AB + CD = BC + DA.
View SolutionConsider a rectangle ABCD having 5, 7, 6, 9 points in the interior of the line segments AB, CD, BC, DA respectively. Let α be the number of triangles having these points from different sides as vertices and β be the number of quadrilaterals having these points from different sides as vertices. Then (β−α) is equal to :
View Solutionएक चतुर्भुज ABCD , जिसके रेखा खंडों AB , CD , BC , DA के अंदर क्रमशः 5,7,6,9 बिंदु है। का विचार कीजिए। माना α उन त्रिभुजों की संख्या है , जिनके शीर्ष भिन्न भुजाओं पर ये बिंदु है तथा β उन चतुर्भुजों की संख्या है। जिनके शीर्ष भिन्न भुजाओं पर ये बिंदु है। तो (β−α) बराबर है:
View Solution
