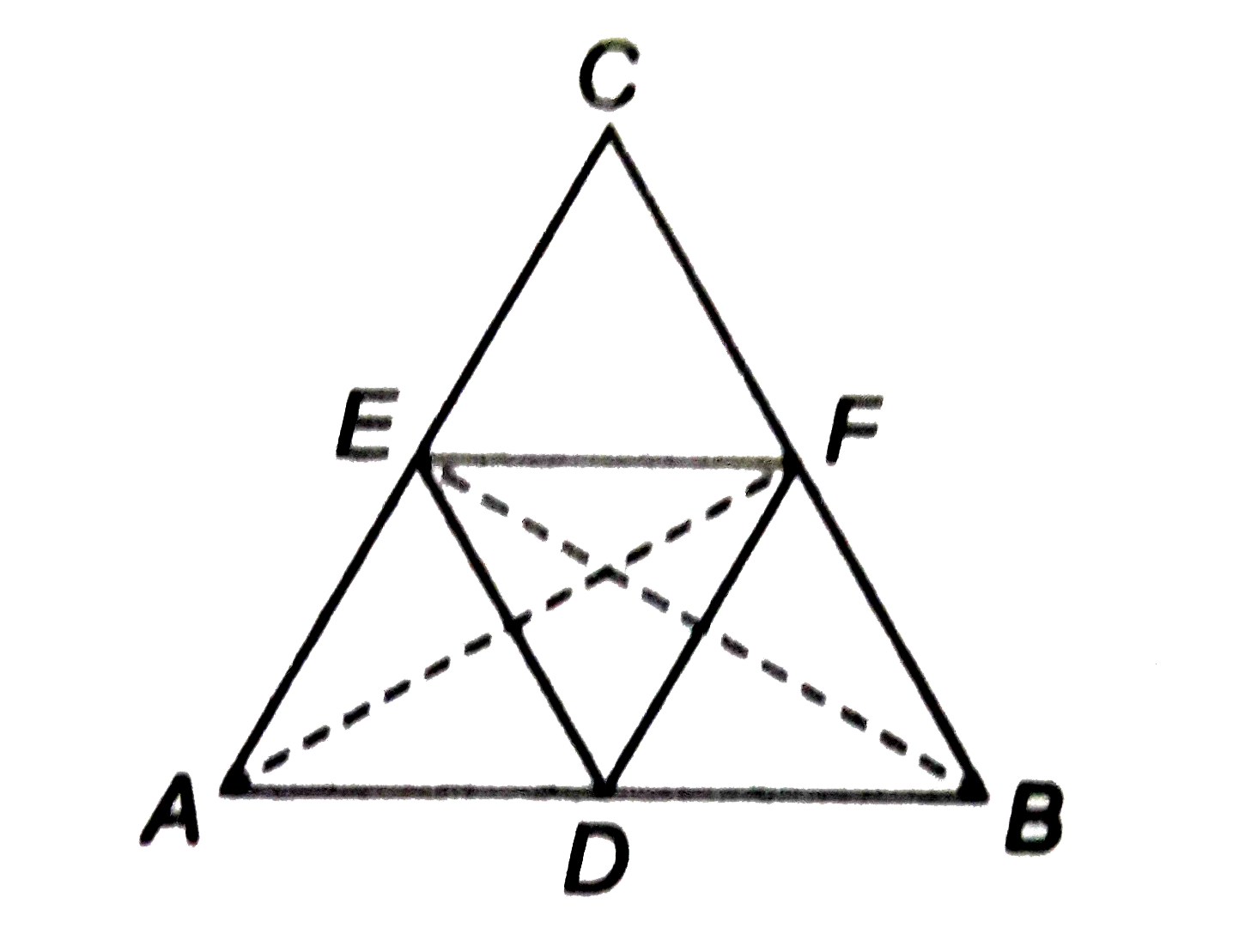
If D, E and F are respectively, the mid-points of AB, AC and BC in ΔABC, then BE + AF is equal toSolution in Punjabi
DC
12BF
2BF
32BF
The correct Answer is:A
BE+AF=OE−OB+OF−OA
=OA+OC2−OB+OB+OC2−OA
=OC−OA+OB2=OC−OD=DC
Topper's Solved these Questions
- BOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise For Session 17videos
VECTOR ALGEBRA
- BOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise For Session 217videos
VECTOR ALGEBRA
- BOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)18videos
TRIGONOMETRIC FUNCTIONS AND IDENTITIES
Similar Questions
Classify the following measure as scalar and vector: 10Kg
03:46View SolutionIf a=2ˆi−ˆj+2ˆkandb=−ˆi+ˆj−ˆk, then find a+b. Also, find a unit vector along a+b.
07:56View SolutionLet αandβ be non-zero real numbers such that 2(cosβ−cosα)+cosαcosβ=1. Then which of the following is/are true ?
01:36View SolutionIf D, E, F are respectively the mid-points of AB, AC and BC respectively in a ΔABC, then −−→BE+−−→AF=
02:00View Solution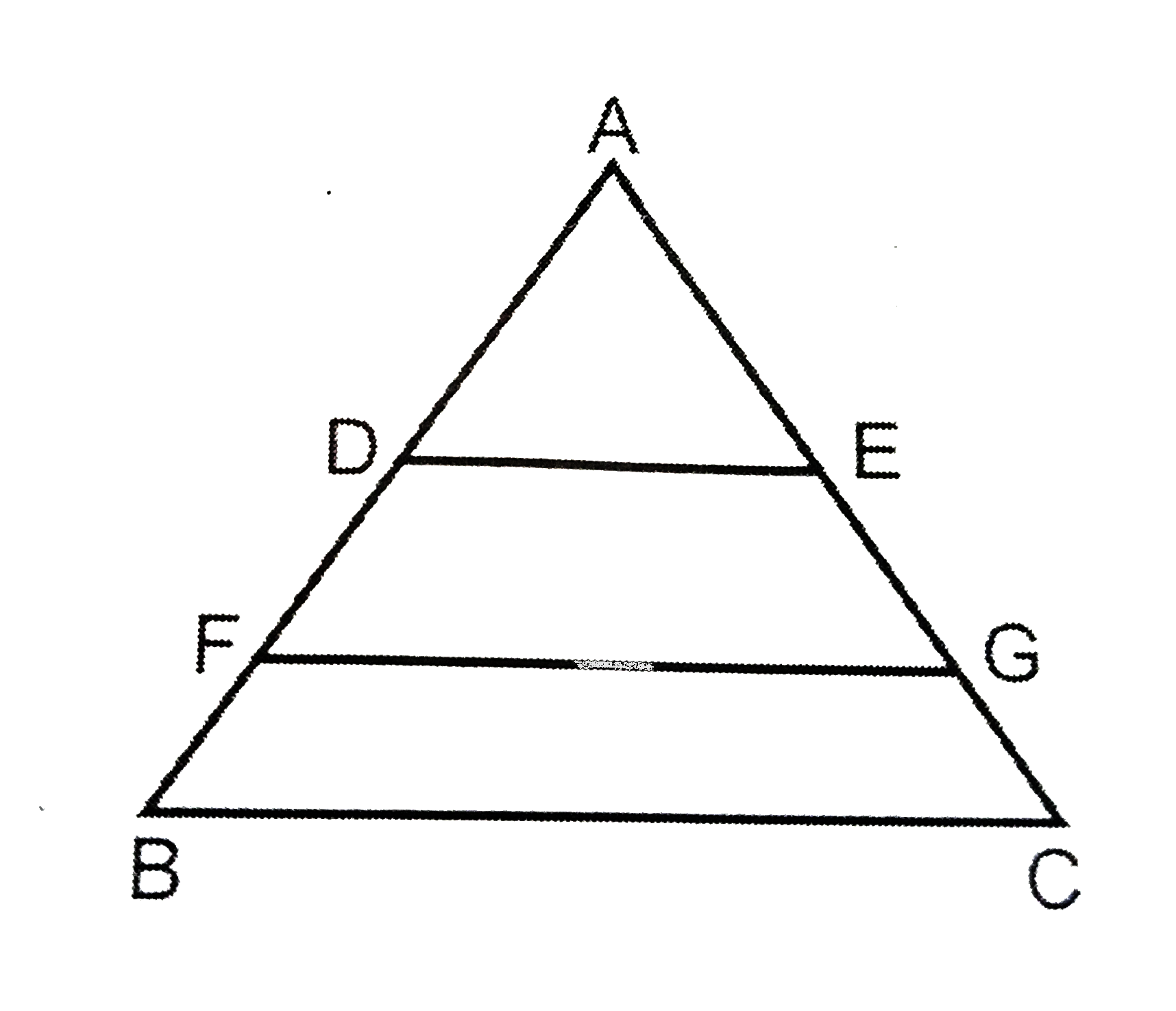
In ΔABC,D and E are the mid-points of AB and AC. Again, F and G are the mid-points of DB and EC, If BC=12,what is the length of FG?03:30View SolutionIf the mid-points of AB, BC and CA of the ΔABC are D, E and F respectively, P and Q are also the mid-points of DE and EF respectively. Then PQ =
View Solution12 defective pens are accidentally mixed with 132 good ones. It is not possible to just look at a pen and tell whether or not it is defective. One pen is taken out at random from this lot. Determine the probability that the pen taken out is a good one.
06:28View SolutionD, E and F are the mid-points of the sides AB, AC and BC of the ΔABC. Prove that DE and AF bisects each other.
04:26View SolutionIf D,E and F are respectively the mid points of AB, AC and BC in △ABC, then −BE+−AF is
05:52View SolutionIf D, E and F are respectively the mid - points of sides BC, AC and AB of a ΔABC. If EF=3cm,FD=4cm and AB=10cm, then DE,BCandCA respectively will be equal to :
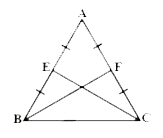
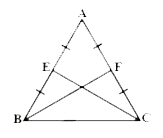
02:15View SolutionE and F are respectively the mid-points of equal sides AB and AC of ΔABC (see figure)
Show that BF = CE.
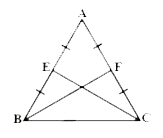
01:59View SolutionE and F are respectively the mid-points of equal sides AB and AC of ΔABC (see figure)
Show that BF = CE.
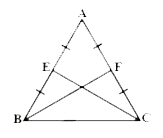
05:08View SolutionE and F are respectively the mid-points of equal sides AB and AC of ΔABC (see figure)
Show that BF = CE.
04:02View SolutionE and F are respectively the mid-points of equal sides AB and AC of ΔABC (see figure)
Show that BF = CE.
02:51View Solutionयदि किसी ΔABC में भुजाओं AB, AC तथा BC के मध्य बिन्दु क्रमश: D, E, F हैं, तब −−→BE+−−→AF=
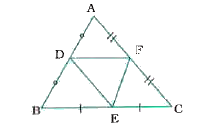
03:19View SolutionD, E and F are respectively the mid-points of sides AB, BC and CA of ΔABC. Find the ratio of the areas of ΔDEF and ΔABC.
06:23View Solution