If [x] dnote the greatest integer less than or equal to x then the equation has no solution in Solution in Punjabi
R
The correct Answer is:A, B, C, D
Step by step video & image solution for If [x] dnote the greatest integer less than or equal to x then the equation sin x=[1+sin x ]+[1-cos x ][ has no solution in by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise (Passage Based Questions)
13 Videos
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Trigonometric Equations and Inequations Exercise 3 :Passage Based Questions
1 Videos
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise (Single Option Correct Type Questions)
60 Videos
- THREE DIMENSIONAL COORDINATE SYSTEMBOOK - ARIHANT MATHSCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Three Dimensional Coordinate System Exercise 12 : Question Asked in Previous Years Exam
2 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
18 Videos
Similar Questions
If number of solution and sum of solution of the equation 3sin2x−7sinx+2=0,x∈[0,2π] are respectively N and S and fn(θ)=sinnθ+cosnθ. On the basis of above information , answer the following questions.
Value of N is
View SolutionIf an angle and a side of a right angle triangle is known , then rest of the sides and angles can be found as follows
In ΔABC(figure ), if ∠B=90∘,∠C=θandBC=x, then AB=xtanθandAC=xsecθ .
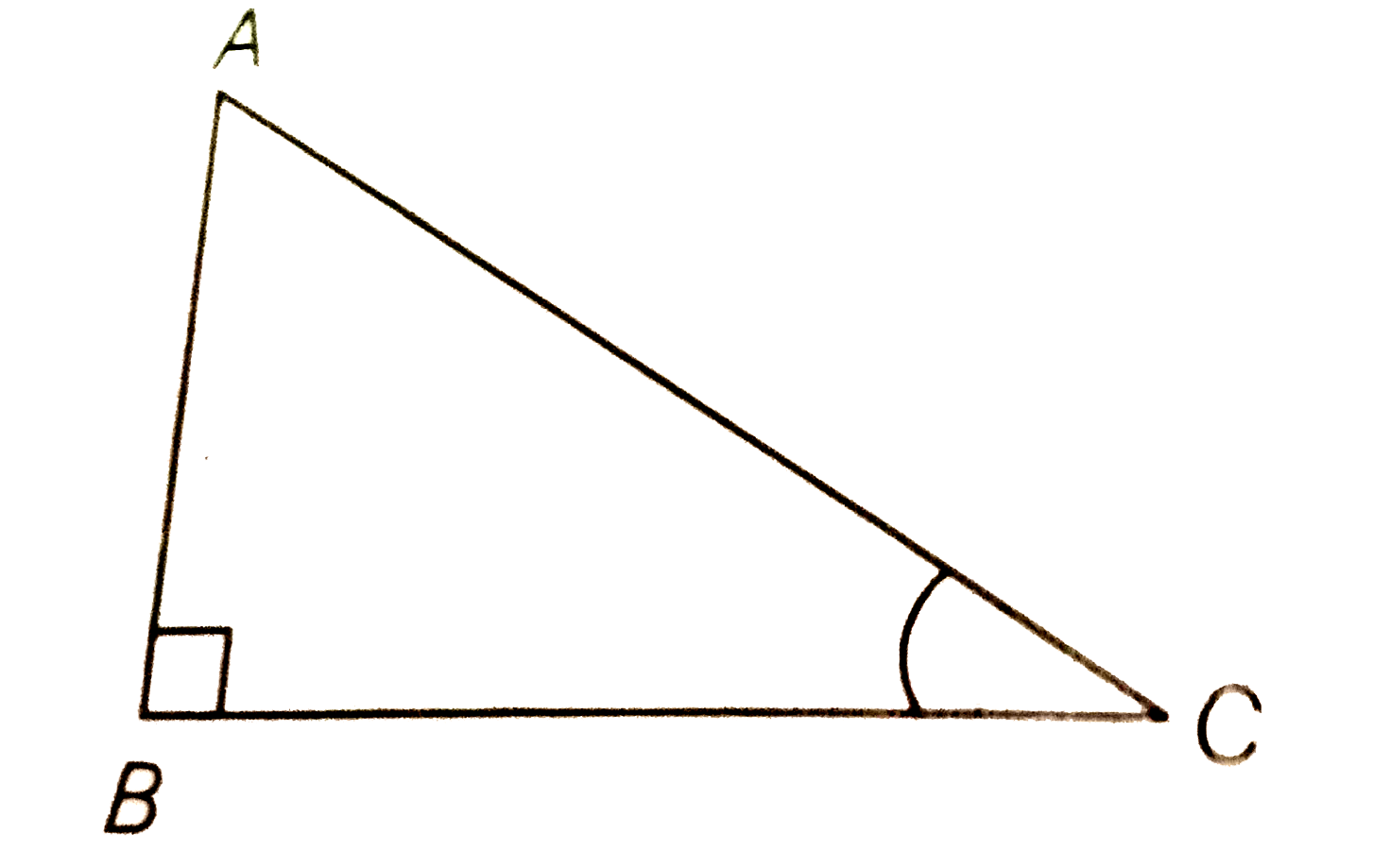
Now, consider in isosceles triangle PQR (Figure 2),
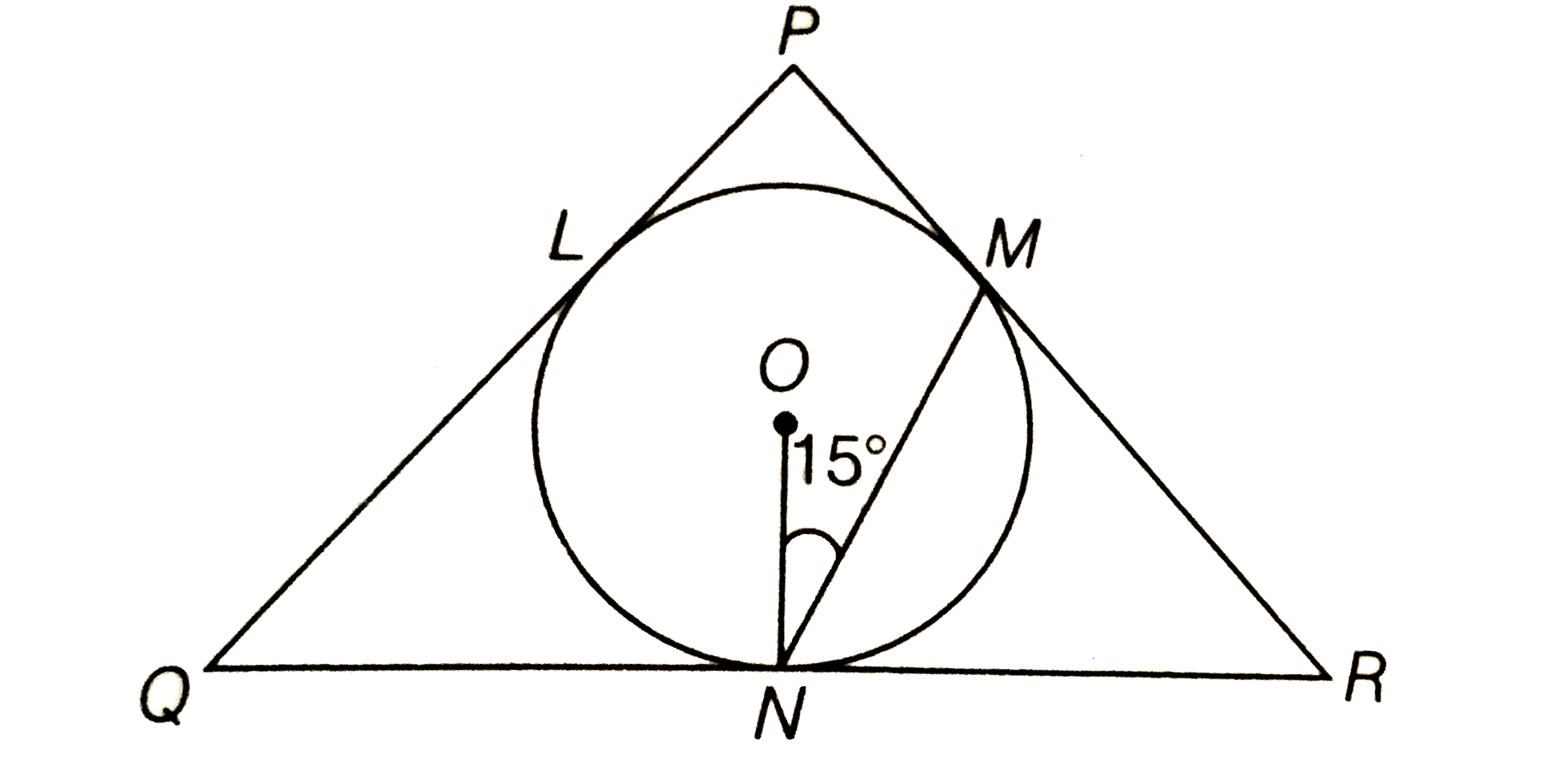
Where PQ=PR and 20N=√3
On the basis of the above information answer the question
The angle of triangle PQR are
View SolutionShow that sinx−sinycosx+cosy=tan(x−y2)
View SolutionConsider the line L1:x−12=y−1=z+31,L2:x−41=y+31=z+32 find the angle between them.
View SolutionLet αandβ be non-zero real numbers such that 2(cosβ−cosα)+cosαcosβ=1. Then which of the following is/are true ?
View SolutionThe solutions of the equation 2x-2[x]=1, where [x]= the greatest integer less than or equal to x, are
View Solutionif f(x)=(sin(4 pi[x]))/(1+[x]^(2)), where [x] is the greatest integer less than or equal to x ,
View SolutionThe equation sin x=[1+sin x]+[1-cos x) has (where [x] is the greatest integer less than or equal to 'x')
View SolutionIf [.] denotes the greatest integer less than or equal to x and (.) denotes the least integer greater than or equal to x, then domain of the function f(x)=sin−1{x+[x]+(x)} is
View SolutionIf [x] denotes the greatest integer less than or equal to x, then the solutions of the equation 2x-2[x]=1 are
View SolutionIf the sum of the greatest integer less than or equal to x and the least integer than or equal to x is 5, then the solution set for x is
View Solutionif [x] denotes the greatest integer less than or equal to x, than limx→0x[x]sin|x|, is
View SolutionShow that the equation , sinx=[1+sinx]+[1−cosx] has no solution for x∈R. ( where [.] represents greatest integers functions ).
View SolutionIf [x] represents the greatest integer less than or equal to x, what is the solution to the equation 1−2[x]=−3 ?
View SolutionIf [y] is the greatest integer less than or equal to y then solution set of [sin−1(cos−1(sin−1(cot−1x)))]=1 is
View SolutionThe equation of sin=[1+sinx]+[1−cosx} has (where [x] is the greatest integer less then or equal to x)
View Solution
