Let Sn=1+2+3++n and Pn=S2S2−1.S3S3−1.S4S4−1...SnSn−1 Where n∈N,(n≥2). Then (lim)n→∞Pn=____Solution in Punjabi
The correct Answer is:∴limn→∞Pn=limn→∞3nn+2=3
Step by step video & image solution for Let S_n=1+2+3++n and P_n=(S_2)/(S_2-1)dot(S_3)/(S_3-1)dot(S_4)/(S_4-1)...(S_n)/(S_n-1) Where n in N ,(ngeq2)dot Then ("lim")_(n→oo)P_n=______ by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LIMITSBOOK - ARIHANT MATHSCHAPTER - LIMITSEXERCISE - SOLVED EXAMPLES
1 Videos
- LIMITSBOOK - ARIHANT MATHSCHAPTER - LIMITSEXERCISE - Exercise (Single Option Correct Type Questions)
38 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - ARIHANT MATHSCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
8 Videos
- LOGARITHM AND THEIR PROPERTIESBOOK - ARIHANT MATHSCHAPTER - LOGARITHM AND THEIR PROPERTIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
4 Videos
Similar Questions
To evaluate limx→a[f(x)], we must analyse the f(x) in right hand neighbourhood as well as in left hand neighbourhood of x=a. E.g. In case of continuous function, we may come across followign cases.
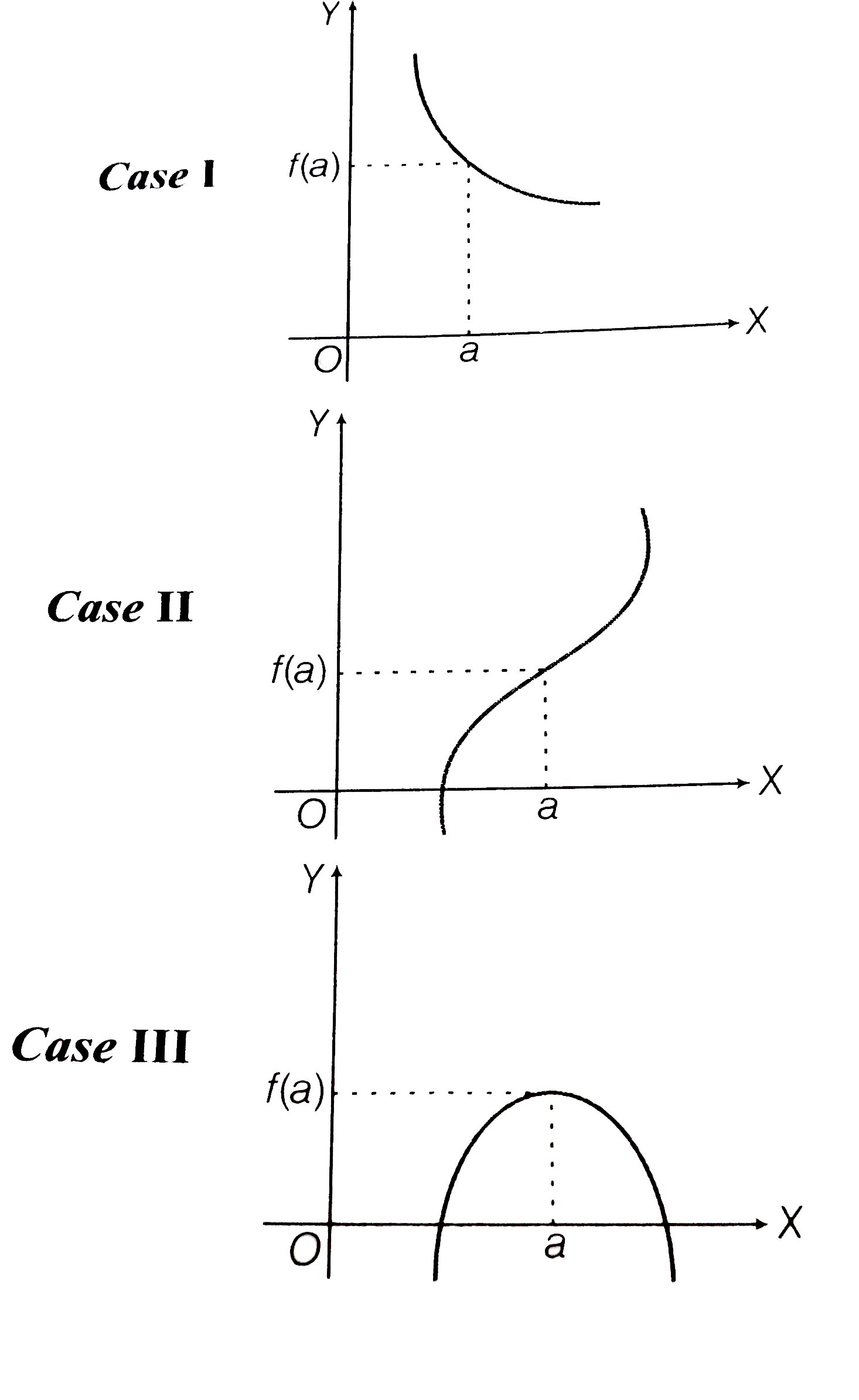
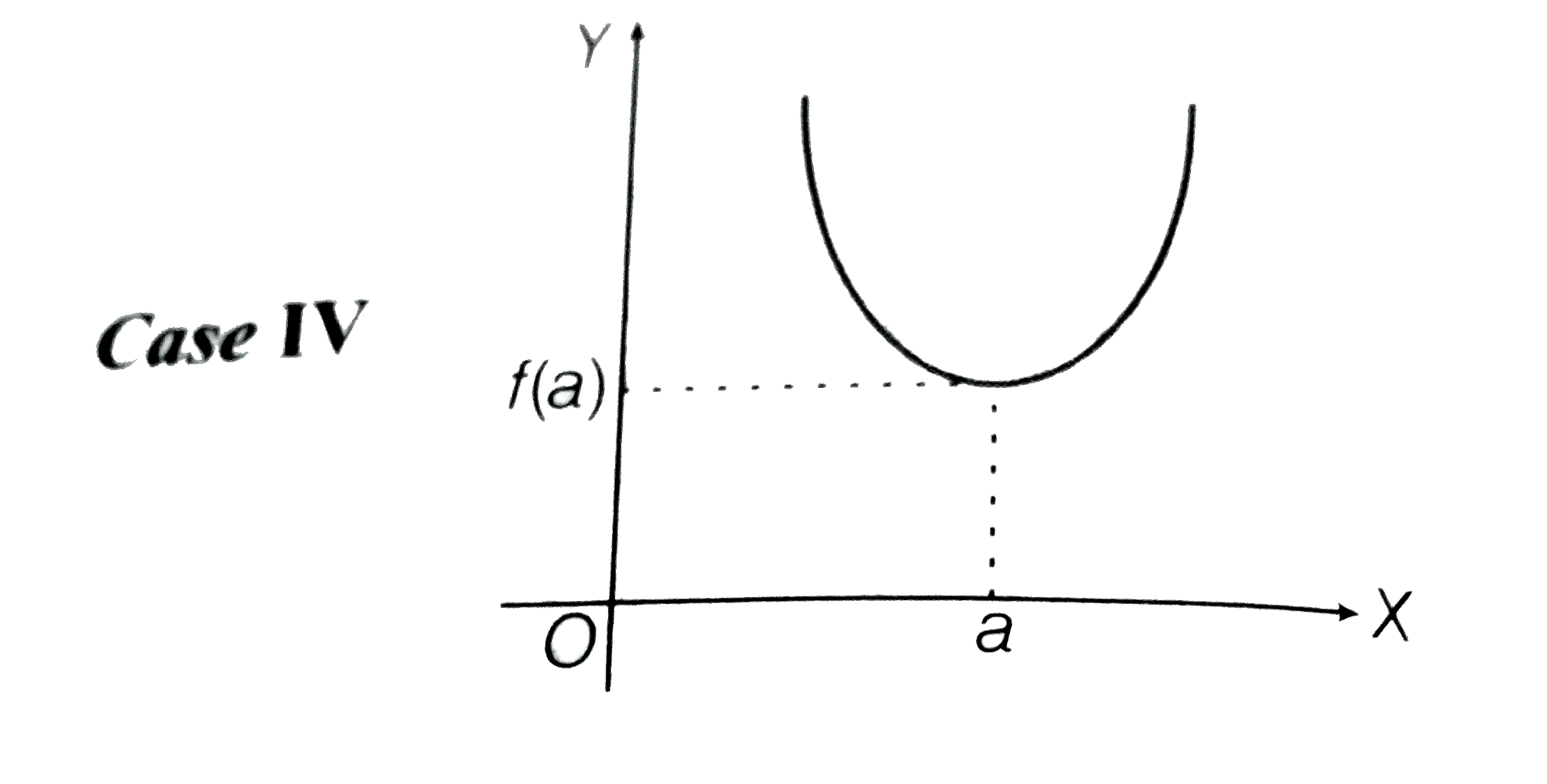
Iff(a) is an integer, the limit will exist in Case III and Case IV but not in Case I and Case II. If f(a) is not an integer, the limit exists in all the cases. ltbgt If f'(1)=−3 and limx→1[f(x)−12] does not exist, (where [.] denotes the greatest integer function), then
View Solutionlimx→0sin(πcos2(tan(sinx)))x2 is equal to
View SolutionIf α=3sin−1(611andβ=3cos−1(49), where the inverse trigonometric functions take only the principal values, then the correct options (s) is (are)
View SolutionIn Which quadrant Point (−2,−3) will be lie.
View SolutionIf S_1,S_2,S_3,...,S_n be the sums of first n terms of n G.P.'s whose first terms are each unity and the common ratios are 1, 2, 3,.....,n respectively, prove that S_1+S_2+2S_3+3S_4+.........+(n-1)S_n=1^n+2^n+3^n+....+n^n.
View SolutionIf alpha, beta are the roots of x^2 + x +1 = 0 and s_n=alpha^n+beta^n then |[3,1+s_1,1+s_2],[1+s_1,1+s_2,1+s_3],[1+s_2,1+s_3,1+s_4]|
View SolutionIf S1,S2,S3,………….Sn denote the sum of 1,2,3…………n terms of an A.P. having first term a andSkxSx is independent of x then S1+S2+S3+……..+Sn= (A) n(n+1)(2n+1)a6 (B) ^(n+2)C3a (C) ^(n+1)C3a (D) none of these
View SolutionLet alpha,beta , be the roots of the equation x^2+x-1=0 . Let S_n=alpha^n+beta^n "for" n ge 1 . Evaluate the determinant |{:(3,1+s_1,1+s_2),(1+s_1,1+s_2,1+s_3),(1+s_2,1+s_3,1+s_4):}|
View SolutionThe sequence of sets
S1,S2,S3,S4,... is defined as S1={1},S2={3,5},S3={7,9,11},S4={13,15,17,19}… etc.
The first element of the nth set Sn is:
View SolutionIf Sn denotes the sum of first n natural number then S1+S2x+S3x2+……+Snxn−1+……∞ terms =
View SolutionThe sequence of sets
S1,S2,S3,S4,... is defined as S1={1},S2={3,5},S3={7,9,11},S4={13,15,17,19}… etc.
The first element of the nth set Sn is:
View SolutionLet Sn(n≤1) be a sequence of sets defined by S1{0},S2={32,52},S3={154,194,234,274},.......then
View SolutionIf α,β are the roots of the equation ax2+bx+c=0 and Sn=αn+βn then evaluate ∣∣ ∣∣31+s1+s21+s11+s21+s31+s21+s31+s4∣∣ ∣∣
View SolutionIf S1,S2,S3,………….Sn denote the sum of 1,2,3…………n terms of an A.P. having first term a andSkxSx is independent of x then S1+S2+S3+……..+Sn= (A) n(n+1)(2n+1)a6 (B) ^(n+2)C3a (C) ^(n+1)C3a (D) none of these
View SolutionLet Sn=1+2+3++n and Pn=S2S2−1.S3S3−1.S4S4−1SnSn−1 Where n∈N,(n≥2). Then (lim)x→∞Pn=____
View SolutionLet Sn(n≥1) be a sequence of sets defined by S1={0},S2={32,52},S4={154,194,234,274},.......then
View Solution
