Let be matrix given by
Such that each horizontal row is arithmetic progression and each vertical column is a geometrical progression. It is known that each column in geometric progression have the same common ratio. Given that and .Let Di be the common difference of row
The value of is equal to Solution in Punjabi
The correct Answer is:D
Step by step video solution for Let A be nxxn matrix given by A=[(a_(11),a_(12),a_(13)……a_(1n)),(a_(21),a_(22),a_(23)…a_(2n)),(vdots, vdots, vdots),(a_(n1),a_(n2),a_(n3).a_("nn"))] Such that each horizontal row is arithmetic progression and each vertical column is a geometrical progression. It is known that each column in geometric progression have the same common ratio. Given that a_(24)=1,a_(42)=1/8 and a_(43)=3/16 .Let Di be the common difference of i^(th) row The value of lim_(nto oo)sum_(i=1)^(n)D_(i) is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LIMITSBook:ARIHANT MATHSChapter:LIMITSExercise:SOLVED EXAMPLES
Explore 1 Video
- LIMITSBook:ARIHANT MATHSChapter:LIMITSExercise:Exercise (Single Option Correct Type Questions)
Explore 38 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBook:ARIHANT MATHSChapter:INVERSE TRIGONOMETRIC FUNCTIONSExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 8 Videos
- LOGARITHM AND THEIR PROPERTIESBook:ARIHANT MATHSChapter:LOGARITHM AND THEIR PROPERTIESExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 4 Videos
Similar Questions
To evaluate limx→a[f(x)], we must analyse the f(x) in right hand neighbourhood as well as in left hand neighbourhood of x=a. E.g. In case of continuous function, we may come across followign cases.
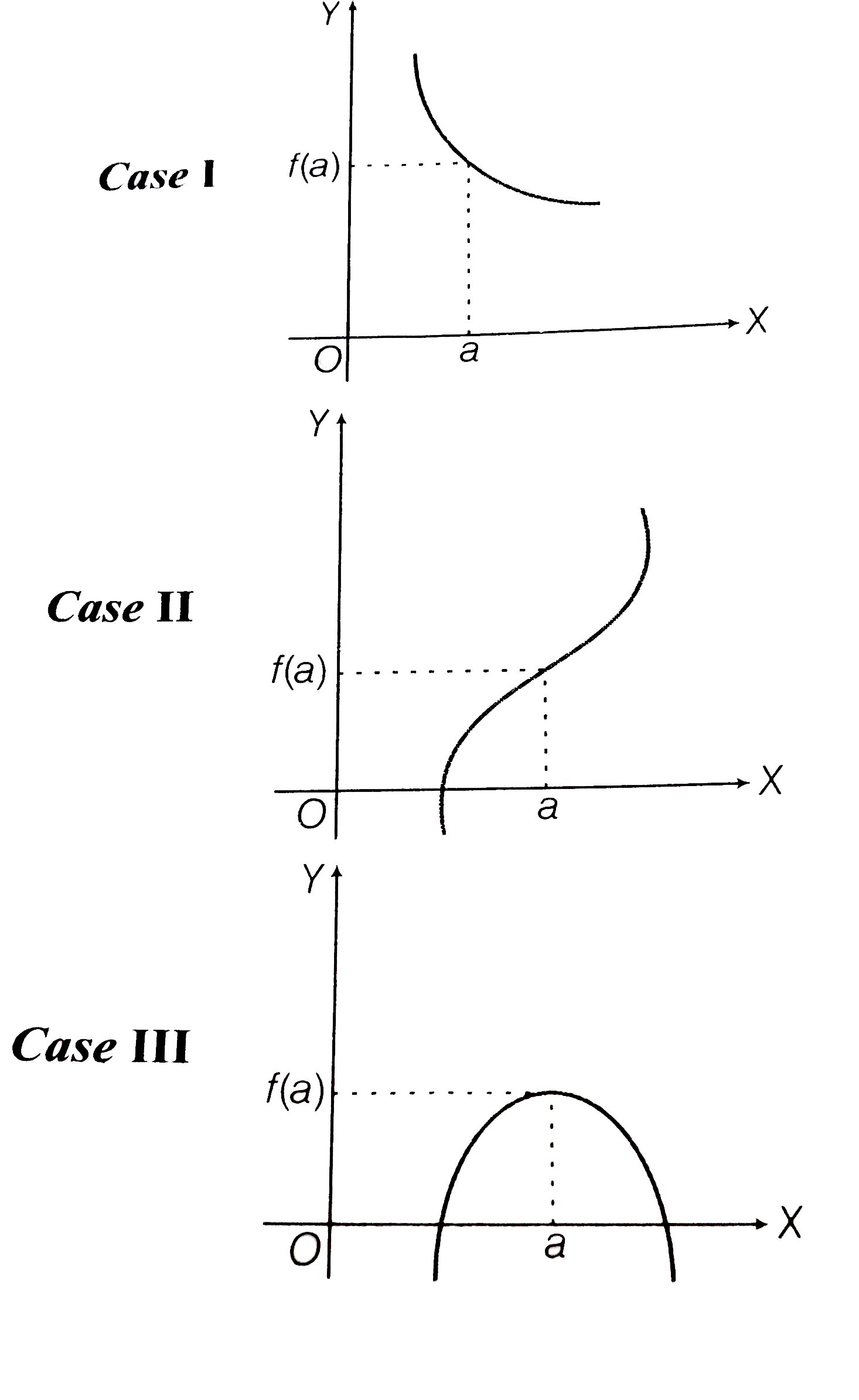
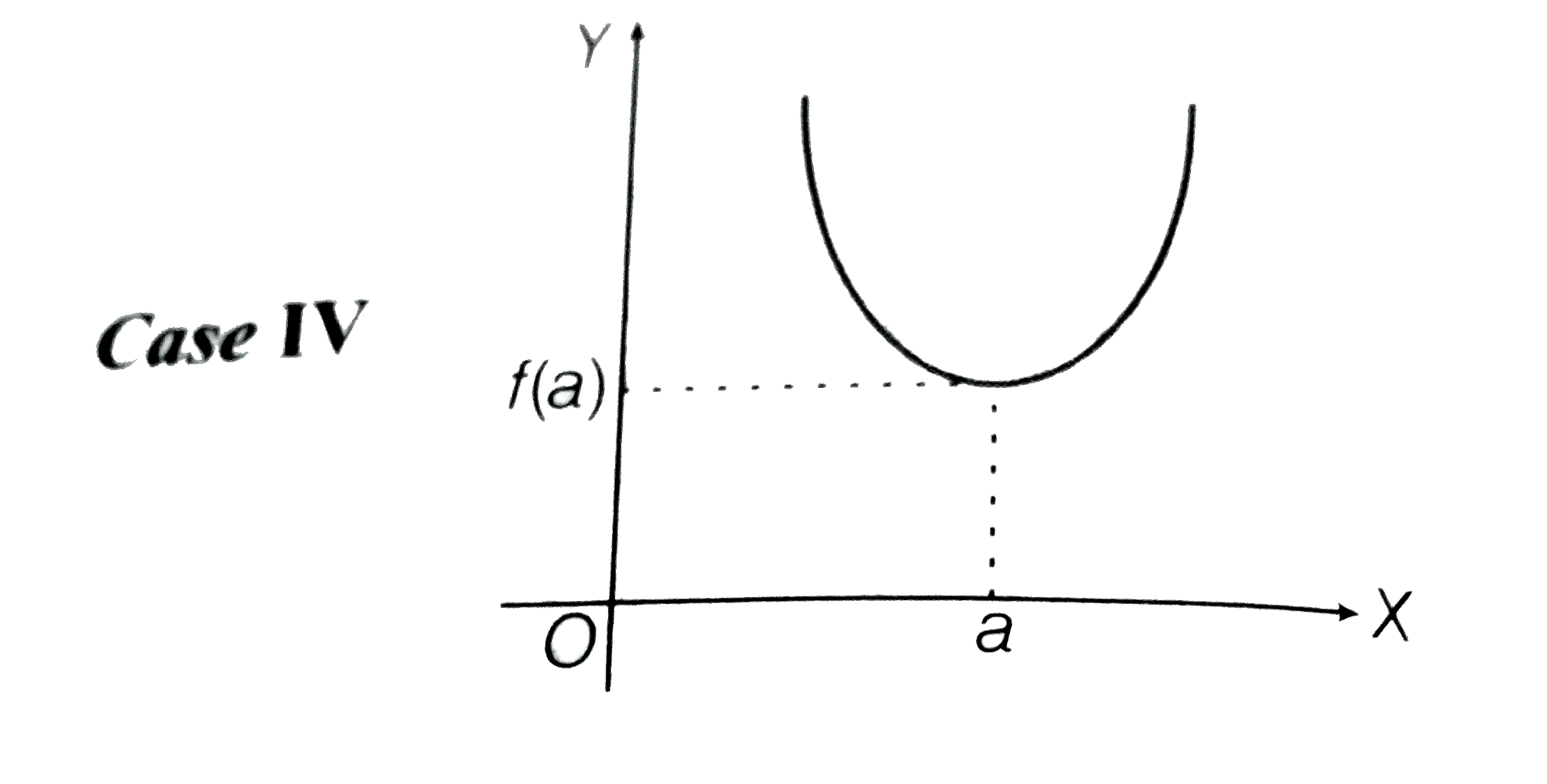
Iff(a) is an integer, the limit will exist in Case III and Case IV but not in Case I and Case II. If f(a) is not an integer, the limit exists in all the cases. ltbgt If f'(1)=−3 and limx→1[f(x)−12] does not exist, (where [.] denotes the greatest integer function), then
View Solutionlimx→0sin(πcos2(tan(sinx)))x2 is equal to
View SolutionIf α=3sin−1(611andβ=3cos−1(49), where the inverse trigonometric functions take only the principal values, then the correct options (s) is (are)
View SolutionIn Which quadrant Point (−2,−3) will be lie.
View SolutionIf Δ=∣∣ ∣∣a11a12a13a21a22a23a31a32a33∣∣ ∣∣ and Aij is cofactors of aij, then value of Δ is given by
View SolutionIf A=⎡⎢⎣110215121⎤⎥⎦ then a11A21+a12A22+a13A23 is equal to
View SolutionLet A be n×n matrix given by
A=⎡⎢ ⎢ ⎢ ⎢ ⎢⎣a11a12a13……a1na21a22a23…a2n⋮⋮⋮an1an2an3.ann⎤⎥ ⎥ ⎥ ⎥ ⎥⎦
Such that each horizontal row is arithmetic progression and each vertical column is a geometrical progression. It is known that each column in geometric progression have the same common ratio. Given that a24=1,a42=18 and a43=316
Let Sn=n∑j=1a4j,limn→∞Snn2 is equal to
View SolutionLet A be n×n matrix given by
A=⎡⎢ ⎢ ⎢ ⎢ ⎢⎣a11a12a13……a1na21a22a23…a2n⋮⋮⋮an1an2an3.ann⎤⎥ ⎥ ⎥ ⎥ ⎥⎦
Such that each horizontal row is arithmetic progression and each vertical column is a geometrical progression. It is known that each column in geometric progression have the same common ratio. Given that a24=1,a42=18 and a43=316
Let di be the common difference of the elements in with row then n∑i=1di is
View SolutionLet A be n×n matrix given by
A=⎡⎢ ⎢ ⎢ ⎢ ⎢⎣a11a12a13……a1na21a22a23…a2n⋮⋮⋮an1an2an3.ann⎤⎥ ⎥ ⎥ ⎥ ⎥⎦
Such that each horizontal row is arithmetic progression and each vertical column is a geometrical progression. It is known that each column in geometric progression have the same common ratio. Given that a24=1,a42=18 and a43=316
The value of limn→∞n∑i=1aii is equal to
View SolutionIf A=⎡⎢⎣a11a12a13a21a22a23a31a32a33⎤⎥⎦ and Aij denote the cofactor of element aij, then a11A21+a12A22+a13A23=
View SolutionIf a1,a2,a3,a4,a5 are consecutive terms of an arithmetic progression with common difference 3, then the value of ∣∣ ∣ ∣∣a23a2a1a24a3a2a25a4a3∣∣ ∣ ∣∣ is
View Solutionयदि A=∣∣ ∣∣10−1345067∣∣ ∣∣ का व्युत्क्रम A−1=∣∣ ∣∣a11a12a13a21a22a23a31a32a33∣∣ ∣∣ है, तब a23 का मान है
View SolutionIf Δ=∣∣ ∣∣a11a12a13a21a22a23a31a32a33∣∣ ∣∣ and Aij is cofactors of aij, then value of Δ is given by
A. a11A31+a12A32+a13A33
B. a11A11+a12A21+a13A31
C. a21A11+a22A12+a23A13
D. a11A11+a21A21+a31A31
View SolutionIf Δ=∣∣ ∣∣a11a12a13a21a22a23a31a32a33∣∣ ∣∣ and Aij Cofators of aij then value of Δ is given by ……..
View SolutionLet A be n×n matrix given by
A=⎡⎢ ⎢ ⎢ ⎢ ⎢⎣a11a12a13……a1na21a22a23…a2n⋮⋮⋮an1an2an3.ann⎤⎥ ⎥ ⎥ ⎥ ⎥⎦
Such that each horizontal row is arithmetic progression and each vertical column is a geometrical progression. It is known that each column in geometric progression have the same common ratio. Given that a24=1,a42=18 and a43=316
Let Sn=n∑j=1a4j,limn→∞Snn2 is equal to
View SolutionLet A be n×n matrix given by
A=⎡⎢ ⎢ ⎢ ⎢ ⎢⎣a11a12a13……a1na21a22a23…a2n⋮⋮⋮an1an2an3.ann⎤⎥ ⎥ ⎥ ⎥ ⎥⎦
Such that each horizontal row is arithmetic progression and each vertical column is a geometrical progression. It is known that each column in geometric progression have the same common ratio. Given that a24=1,a42=18 and a43=316
Let di be the common difference of the elements in with row then n∑i=1di is
View Solution
