If is a tangent to the hyperbola then which of the following CANNOT be sides of a right angled triangle?Solution in Punjabi
The correct Answer is:A, B, C
Step by step video & image solution for If 2x-y+1=0 is a tangent to the hyperbola (x^2)/(a^2)-(y^2)/(16)=1 then which of the following CANNOT be sides of a right angled triangle? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- HYPERBOLABOOK - ARIHANT MATHSCHAPTER - HYPERBOLAEXERCISE - Hyperbola Exercise 11 : Questions Asked in Previous 13 Years Exams
3 Videos
- HYPERBOLABOOK - ARIHANT MATHSCHAPTER - HYPERBOLAEXERCISE - Hyperbola Exercise 10 : Subjective Type Questions
4 Videos
- GRAPHICAL TRANSFORMATIONSBOOK - ARIHANT MATHSCHAPTER - GRAPHICAL TRANSFORMATIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
10 Videos
- INDEFINITE INTEGRAL BOOK - ARIHANT MATHSCHAPTER - INDEFINITE INTEGRAL EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
8 Videos
Similar Questions
which term of the following sequence : 312,3,332.....is 81?
View Solutionwhich term of given sequence: 2,232,22 ... is 32?
View SolutionFind the number of solutions of the equation x21−|x−2|=1, graphically.
View SolutionThe integral ∫sec2x(secx+tanx)92dx equals (for some arbitrary constant K).
View SolutionIf 2x−y+1=0 is a tangent to the hyperbola x2a2−y216=1 then which of the following CANNOT be sides of a right angled triangle? (a)a,4,2 (b) a,4,1 (c)2a,4,1 (d) 2a,8,1
View SolutionIf 2x−y+1=0 is a tangent to hyperbola x2a2+y216=1, then which of the following are sides of a right angled triangle ?
View SolutionThe area of triangle formed by the lines x−y=0,x+y=0 and any tangent to the hyperbola x2−y2=16 is equal to
View Solutionयदि 2x−y+1=0 अतिपरवलय x2a2−y216=1 की स्पर्शरेखा है तो निम्न में से कौनसी समकोणीय त्रिभुज की भुजायें नहीं हो सकती है (हैं)
View Solutionअतिपरवलय x29−y216=1 तथा y29−x216=1 की उभयनिष्ठ स्पर्श रेखा की प्रवणता है
View SolutionIf 3x+2y−1=0 is a tangent to a hyperbola x216−y29=1, then the point of contact is
View SolutionHyperbola x^2/(25)-y^2/(16)=1 The line that follows 2x+y=1 Is perpendicular to, the equation of is
View SolutionIf 2x−y+1=0 is a tangent to the hyperbola x2a2−y216=1 then which of the following CANNOT be sides of a right angled triangle?
View Solutionआकृति में यदि ABIICD,∠APQ=50∘और∠PRD=127∘ है, तो Y का मान है-
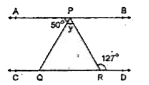
View Solutionयदि 2x−y+1=0 अतिपरवलय x2a2−y216=1 की स्पर्शरेखा है तो निम्न में से कौनसी समकोणीय त्रिभुज की भुजायें नहीं हो सकती है (हैं)
View SolutionTangents drawn from a point on the circle x2+y2=9 to the hyperbola x225−y216=1,
then tangents are at angle
View SolutionIf 2x−y+1=0 is a tangent to hyperbola x2a2+y216=1, then which of the following are sides of a right angled triangle ?
View Solution
