A vertical line passing through the point (h,0) intersects th ellipse x24+y29=1 at the point P and Q. Let the tangents to the ellipse at P and Q meet at the point R. If △(h)=area of the triangle PQR ,trianl≥1=12≤max△(h)and△2=12≤min△(h) then 8√5△1−8△2=Solution in Punjabi
The correct Answer is:9
Step by step video & image solution for A vertical line passing through the point (h,0) intersects th ellipse x^(2)/4+y^(2)/9=1 at the point P and Q. Let the tangents to the ellipse at P and Q meet at the point R. If triangle(h)=area of the triangle PQR ,trianlge_1=1/2 lemax triangle(h) and triangle_2=1/2lemintriangle(h) then 8/sqrt5triangle_1-8triangle_2= by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHSCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - MAXIMA AND MINIMA EXERCISE 6
2 Videos
- MATRICES BOOK - ARIHANT MATHSCHAPTER - MATRICES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
49 Videos
- PAIR OF STRAIGHT LINES BOOK - ARIHANT MATHSCHAPTER - PAIR OF STRAIGHT LINES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
2 Videos
Similar Questions
The figure shows a right triangle with its hypotenuse OB along the y-axis and its vertex A on the parabola y=x2.
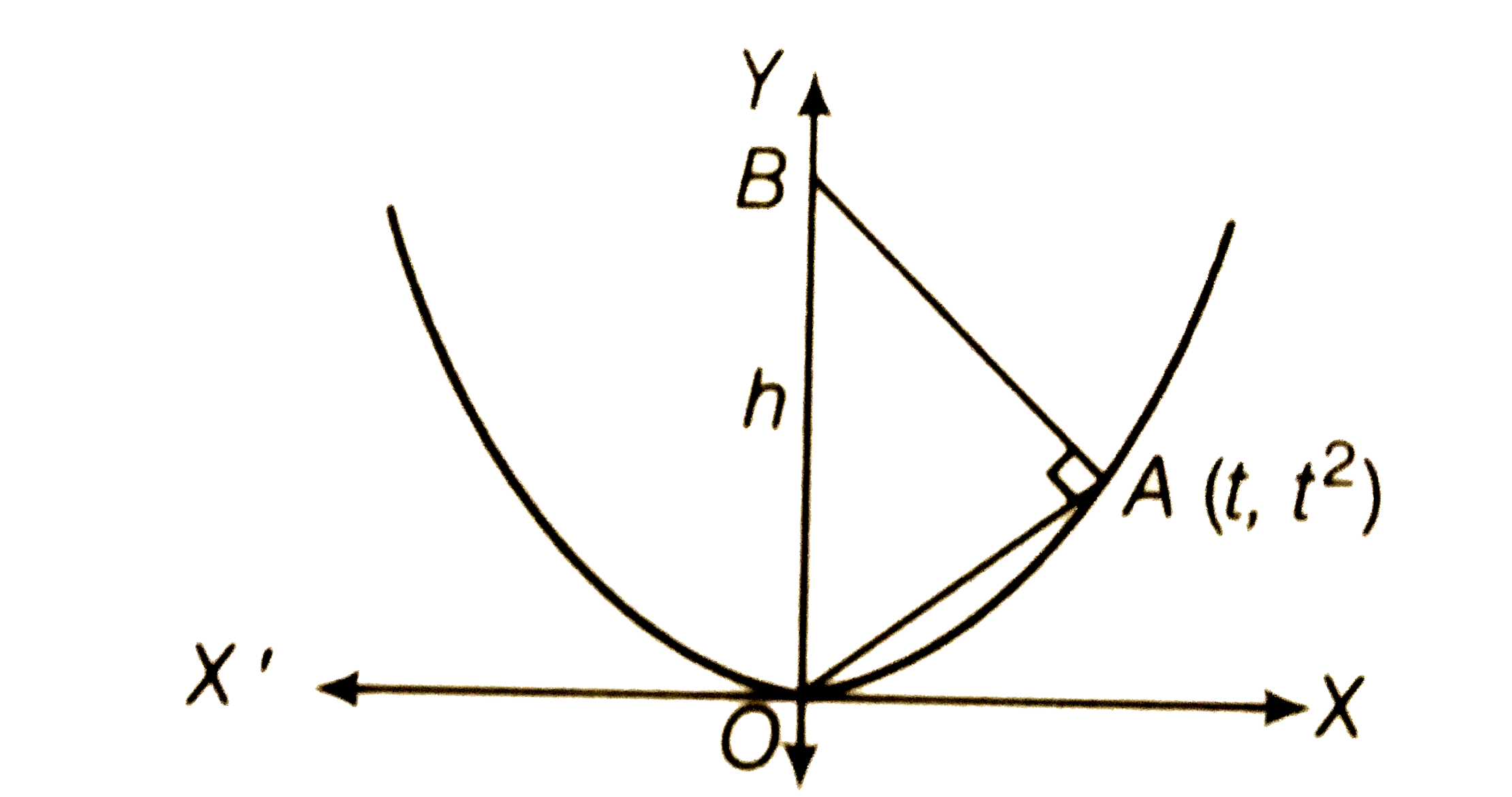
Let h represents the length of the hypotenuse which depends on the x-coordinate of the point A. The value of limt→o(h) is equal to
View SolutionLet A=⎡⎢⎣1000110−24⎤⎥⎦,I=⎡⎢⎣100010001⎤⎥⎦andA−1=[16(A2+cA+dI)] Then value of candd are (a) (−6,−11) (b) (6,11) (c) (−6,11) (d) (6,−11)
View SolutionIf the pair of lines ax2+2(a+b)xy+by2=0 lie along diameters of a circle and divide the circle into four sectors such that the area of one of the sectors is thrice the area of another sector then
View SolutionA vertical line passing through the point (h,0) intersects the ellipse (x^(2))/(4)+(y^(2))/(3)=1 at the points P and Q. Let the tangents to the ellipse at P and Q meet at R. If delta(h) Area of triangle delta PQR, and delta_(1)max_((1)/(2)<=h<=1)delta(h) A further delta_(2)min_((1)/(2)<=h<=1)delta(h) Then (8)/(sqrt(5))delta_(1)-8 delta_(2)
View SolutionLines x + y = 1 and 3y = x + 3 intersect the ellipse x2+9y2=9 at the points P , Q , R . The area of the triangle PQR is _
View SolutionThe straight line x4+y3=1 intersects the ellipse x216+y29=1 at two points A and B there is a point P on this ellipse such that the area of Delta PAB is equal to 6(√2−1) . Then the number of such point (P) is/are (A) 4 (B) 1 (C) 2 (D) 3
View SolutionIf the area (in sq. units) of the triangle formed by the intersection of a line parallel to the x - axis and passing through the point P(h,k) with the line y=x and x+y=2 is 4h2, then the locus of the point P is
View Solutionबिन्दु (h, 0) से गुजरने वाली एक ऊर्ध्वाधर रेखा दीर्घवृत्त x24+y23=1 को बिन्दुओं P तथा Q पर काटती है। माना कि बिन्दुओं P तथा Q पर दीर्घवृत्त की स्पर्शरेखाएँ बिन्दु R पर मिलती हैं । यदि Δ(h) = त्रिभुज PQR का क्षेत्रफल, Δ1=max1/2≤h≤1Δ(h) और Δ2=min1/2≤h≤1Δ(h) है तब 8√5Δ1−8Δ2=
View SolutionThe area of the triangle formed by the intersection of a line parallel to x-axis and passing through P(h,k), with the lines y=x and x+y=2 is h2. The locus of the point P is
View SolutionA vertical line passing through the point (h,0) intersects the ellipse x24+y23=1 at the points P and Q.Let the tangents to the ellipse at P and Q meet at R. If Δ(h) Area of triangle ΔPQR, and Δ1=max12≤h≤1Δ(h) and Δ2=min12≤h≤1Δ(h) Then 8√5Δ1−8Δ2
View Solutionदीर्घवृत्त x29+y24=1 तथा परवलय y2=2x विचार कीजिये। यह एक दूसरे को बिन्दु P तथा Q पर क्रमशः प्रथम तथा चतुर्थ, चतुर्थांश में प्रतिच्छेद करते है। दीर्घवृत्त के P तथा Q पर खींची गयी स्पर्श रेखाएं x-अक्ष को बिन्दु R पर मिलती है तथा परवलय के बिन्दु P तथा Q से खींची स्पर्श रेखाएँ x-अक्ष पर बिन्दु S पर मिलती है।
त्रिभुजों PQS तथा PQR के क्षेत्रफलों का अनुपात होगा: (i) 1:3 (ii) 1:2 (iii) 2:3 (iv) 3:4
View Solutionबिन्दु (h, 0) से गुजरने वाली एक ऊर्ध्वाधर रेखा दीर्घवृत्त x24+y23=1 को बिन्दुओं P तथा Q पर काटती है। माना कि बिन्दुओं P तथा Q पर दीर्घवृत्त की स्पर्शरेखाएँ बिन्दु R पर मिलती हैं । यदि Δ(h) = त्रिभुज PQR का क्षेत्रफल, Δ1=max1/2≤h≤1Δ(h) और Δ2=min1/2≤h≤1Δ(h) है तब 8√5Δ1−8Δ2=
View Solutionबिन्दु (h, 0) से गुजरने वाली एक ऊर्ध्वाधर रेखा दीर्घवृत्त x24+y23=1 को बिन्दुओं P तथा Q पर काटती है। माना कि बिन्दुओं P तथा Q पर दीर्घवृत्त की स्पर्शरेखाएँ बिन्दु R पर मिलती हैं । यदि Δ(h) = त्रिभुज PQR का क्षेत्रफल, Δ1=max1/2≤h≤1Δ(h) और Δ2=min1/2≤h≤1Δ(h) है तब 8√5Δ1−8Δ2=
View SolutionA line meets the axes at P and Q such that the centroid of the triangle OPQ is (h,h). The equation of the line PQ is
View SolutionIf the area (in sq. units) of the triangle formed by the intersection of a line parallel to the x - axis and passing through the point P(h,k) with the line y=x and x+y=2 is 4h2, then the locus of the point P is
View SolutionA vertical line passing through the point (h, 0) intersects the ellipse x^2/4+y^2/3=1 at the points P and Q .Let the tangents to the ellipse at P and Q meet at R . If delta (h) Area of triangle deltaPQR , and delta_1 max_(1/2<=h<=1)delta(h) A further delta_2 min_(1/2<=h<=1) delta (h) Then 8/sqrt5 delta_1-8delta_2
View Solution
