i) Area of the rectangle = (length × breadth)
ii) Perimeter of the rectangle = 2 (length + width)
Use the above information to solve the following
The Perimeter of rectangle is 20 cm. If the length of the rectangle is 6 cm. Find the width of the rectangle ?
To find the width of the rectangle given the perimeter and length, we can follow these steps:
1. Understand the formulas:
- The formula for the perimeter of a rectangle is given by:
Perimeter=2×(Length+Width)
2. Identify the known values:
- From the problem, we know:
- Perimeter = 20 cm
- Length = 6 cm
3. Set up the equation:
- Let the width of the rectangle be b.
- Substitute the known values into the perimeter formula:
20=2×(6+b)
4. Simplify the equation:
- First, simplify the right side:
20=2×6+2b
20=12+2b
5. Isolate the term with b:
- Subtract 12 from both sides:
20−12=2b
8=2b
6. Solve for b:
- Divide both sides by 2:
b=82
b=4
7. Conclusion:
- The width of the rectangle is 4 cm.
Final Answer:
The width of the rectangle is 4 cm.
- The formula for the perimeter of a rectangle is given by:
Perimeter=2×(Length+Width)
- From the problem, we know:
- Perimeter = 20 cm
- Length = 6 cm
- Let the width of the rectangle be b.
- Substitute the known values into the perimeter formula:
20=2×(6+b)
- First, simplify the right side:
20=2×6+2b
20=12+2b
- Subtract 12 from both sides:
20−12=2b
8=2b
- Divide both sides by 2:
b=82
b=4
- The width of the rectangle is 4 cm.
The width of the rectangle is 4 cm.
Topper's Solved these Questions
Similar Questions
i) Area of the rectangle = (length × breadth)
ii) Perimeter of the rectangle = 2 (length + width)
Use the above information to solve the following
The area of a rectangle is 24 cm2. The width is two less than the length. Find the length and width of rectangle.
View Solutioni) Area of the rectangle = (length × breadth)
ii) Perimeter of the rectangle = 2 (length + width)
Use the above information to solve the following
If the length and width of the rectangle is give as 10 cm and 6 cm respectively. Then find the area and perimeter of the rectangle?
View Solution
Knowledge Check
The perimeter of a rectangle is 50 cm. Its area and length are in the ratio of 5:1. Find the length of the rectangle?
A22 cmB18 cmC20 cmD15 cmThe difference between the length and the breadth of a rectangle is 7 Cms and the perimeter of the rectangle is 50 Cms What is the area of the rectangle ?
A144 Sq CmsB154 Sq CmsC288 Sq CmsD216 Sq CmsThe ratio of the length and breadth of a rectangle is 7 : 4. If the perimeter of the rectangle is 220 cm, then what is the breadth (in cm) of the rectangle?
A45B50C40D65
The perimeter of a rectangle is 130 cm. If the breadth of the rectangle is30 cm, find its length. Also find the area of the rectangle.
View SolutionThe perimeter of a rectangle is 40 m. The length of the rectangle is 4m less than 5 times its breadth. Find the length of the rectangle.
View Solution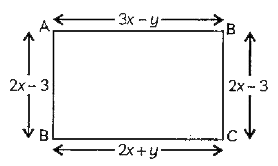
Given that the perimeter of the rectangle is 120cm, find:
the length and the breadth
View SolutionThe area of a rectangle is 630 cm2 and its length is 35 cm. find the perimeter of the rectangle.
View SolutionThe length of a rectangle is two times its width. The perimeter of the rectangle is 180 cm. Find the dimensions of the rectangle.
View Solution
