A swimmer start swimming from a sea shore in south direction and other one in east direction. Their distance becomes 100 km. in 2 hrs. If speed of one swimmer is 75% of another swimmer, find the speed of swimmer who swims faster ?
30 km./hr.
40 km./hr.
45 km./hr.
60 km./hr.
The correct Answer is:B
To solve the problem step by step, we will follow these steps:
Step 1: Understand the problem
We have two swimmers: one swims south and the other swims east. The distance between them after 2 hours is 100 km. The speed of one swimmer is 75% of the other swimmer's speed.
Step 2: Define variables
Let:
- The speed of the faster swimmer (swimmer B) be vB km/h.
- The speed of the slower swimmer (swimmer A) be vA km/h.
According to the problem, we have:
vA=0.75vB
or
vA=34vB
Step 3: Express the speeds in terms of a variable
Let’s denote the speed of swimmer B as 4x km/h. Then, the speed of swimmer A will be:
vA=3x km/h.
Step 4: Calculate the distance covered by each swimmer
In 2 hours, the distance covered by swimmer A is:
DistanceA=vA×2=3x×2=6x km.
The distance covered by swimmer B is:
DistanceB=vB×2=4x×2=8x km.
Step 5: Use the Pythagorean theorem
The total distance between the two swimmers after 2 hours is given as 100 km. According to the Pythagorean theorem:
(6x)2+(8x)2=1002
Step 6: Solve the equation
Calculating the squares:
36x2+64x2=10000
100x2=10000
x2=100
x=10
Step 7: Find the speeds of the swimmers
Now we can find the speeds:
- Speed of swimmer A:
vA=3x=3×10=30 km/h
- Speed of swimmer B:
vB=4x=4×10=40 km/h
Step 8: Conclusion
The speed of the faster swimmer (swimmer B) is:
40 km/h
---
We have two swimmers: one swims south and the other swims east. The distance between them after 2 hours is 100 km. The speed of one swimmer is 75% of the other swimmer's speed.
Let:
- The speed of the faster swimmer (swimmer B) be vB km/h.
- The speed of the slower swimmer (swimmer A) be vA km/h.
vA=0.75vB
or
vA=34vB
Let’s denote the speed of swimmer B as 4x km/h. Then, the speed of swimmer A will be:
vA=3x km/h.
In 2 hours, the distance covered by swimmer A is:
DistanceA=vA×2=3x×2=6x km.
DistanceB=vB×2=4x×2=8x km.
The total distance between the two swimmers after 2 hours is given as 100 km. According to the Pythagorean theorem:
(6x)2+(8x)2=1002
Calculating the squares:
36x2+64x2=10000
100x2=10000
x2=100
x=10
Now we can find the speeds:
- Speed of swimmer A:
vA=3x=3×10=30 km/h
- Speed of swimmer B:
vB=4x=4×10=40 km/h
The speed of the faster swimmer (swimmer B) is:
40 km/h
Topper's Solved these Questions
Similar Questions
A steamer goes downstream from one point to another in 7 hours. It covers the same distance upstream in 8 hours. If the speed of stream be 2 km/hr, find the speed of the steamer in still water and the distance between the ports.
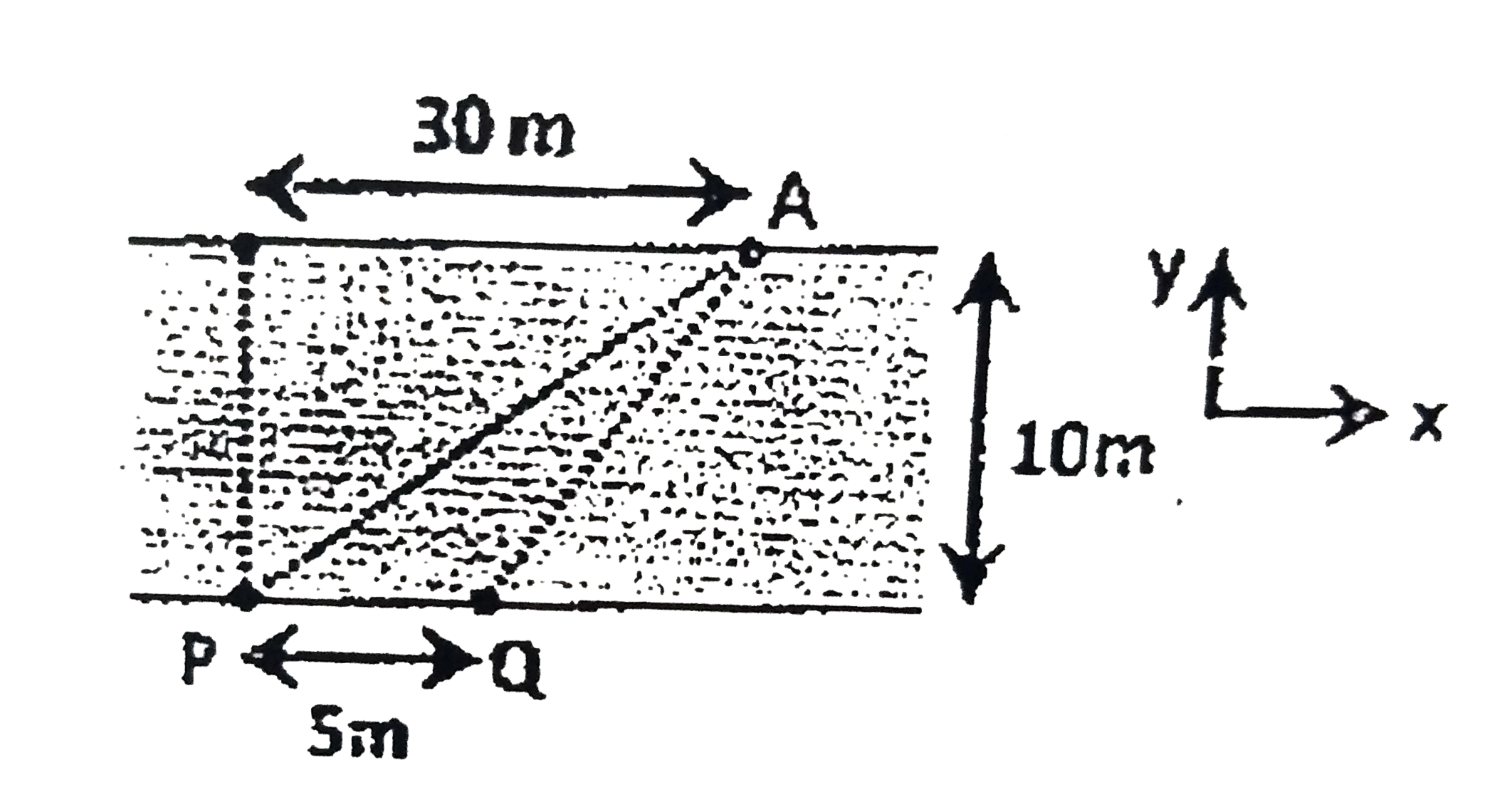
View SolutionTwo swimmers P and Q start swimming from different positions on the same bank as shown in the figure. The swimmer P swims at an angle 90∘ with respect to the river to reach point A. He takes 120 second to cross the river of width 10m. The swimmer Q also takes the same time to reach the point A. the velocity component of Q along x-axis with respect to ground is K/24. Find the value of K.
View Solution
Knowledge Check
A swimmer start swimming from a sea shore in south direction and other one in east direction. Their distance becomes 100 km. in 2 hrs. If speed of one swimmer is 75% of another swimmer, find the speed of swimmer who swims faster ?
A30 km./hr.B40 km./hr.C45 km./hr.D60 km./hr.A swimmer crosses a river with minimum possible time 10 Second. And when he reaches the other end starts swimming in the direction towards the point from where he started swimming. Keeping the direction fixed the swimmer crosses the river in 15 sec. The ratio of speed of swimmer with respect to water and the speed of river flow is (Assume contant speed of river & swimmer)
A32B94C2√5D√52To cross the river in shortest distance, a swimmer should swimming an angle theta with the upsteram. What is the ratio of the time taken to swim across in the shortest time to that in swimming across over shortest distance. [Asume that the speed of swimmer in still water is greater than the speed of river flow]
AcosθBsinθCtanθDcotθ
A swimmer starts to swim from point a to cross a river. He wants to reach point B on the opposite side of the river. The line AB makes an angle 60∘ with the river flow as hown. The velocity of the swimmer in still water is same as that of the water

(i) In what direction should he try to direct his velocity ? Calculate angle between his velocity anf river velocity.
(ii) Find the ratio of the time taken to cross the river in this situation to the minimum time in which he can cross this river.
View SolutionA swimmer starts to swim from point a to cross a river. He wants to reach point B on the opposite side of the river. The line AB makes an angle 60∘ with the river flow as shown. The velocity of the swimmer in still water is same as that of the water
(i) In what direction should he try to direct his velocity ? Calculate angle between his velocity ? Calculate angle between his velocity and river velocity.
(ii) Find the ratio of the time taken to cross the river in this situation to the minimum time in which he can cross this river.

View SolutionA swimmer S is in the sea at a distance dkm from the closest point A on a straight shore. The house of the swimmer is on the shore at distance Lkm from A. He can swin at speed oh ukm/h. walk at speed at vkm/h. At what point on shore should he land so that hhe reaches his house in the shortest time.
View SolutionRamu swims a distance of 3 km each upstream and downstream. The total time taken is one hour. If the speed of the stream is 4 km/h, then find the speed of Ramu in still water.
View SolutionTwo trains starting at the same time from two stations 200 km apart and going in opposite directions cross each other at a distance of 110 km from one of the stations. What is the ratio of their speeds? 9:20 b. 11:9 c. 11:20 d. none of these
View Solution