If A is 3/4 part of B,B is 1/2 part of C is 4/5 part of D then find value of A:B:C:D?
3:5:7:10
2:3:7:9
3:4:8:10
3:5:6:9
To find the value of A:B:C:D given the relationships between them, we can follow these steps:
Step 1: Define the relationships
We know:
- A is 34 of B
- B is 12 of C
- C is 45 of D
Step 2: Express A in terms of B
From the first relationship, we can express A as:
A=34B
This implies:
AB=34
Step 3: Express B in terms of C
From the second relationship, we can express B as:
B=12C
This implies:
BC=12
Step 4: Express C in terms of D
From the third relationship, we can express C as:
C=45D
This implies:
CD=45
Step 5: Find a common base for ratios
To find A:B:C:D, we can express all variables in terms of D.
1. From C=45D, we can express D as:
D=54C
2. Substitute C in terms of D into B:
B=12C=12(45D)=410D=25D
3. Substitute B in terms of D into A:
A=34B=34(25D)=620D=310D
Step 6: Write the ratios
Now we have:
- A=310D
- B=25D
- C=45D
- D=D
Now, we can express the ratios:
A:B:C:D=310D:25D:45D:D
Step 7: Remove D from the ratios
Since D is common in all terms, we can simplify:
A:B:C:D=310:25:45:1
Step 8: Convert to a common denominator
To express these ratios in whole numbers, we can convert them to a common denominator:
- The common denominator for 10,5,5,1 is 10.
Thus:
- A=310 becomes 3
- B=25=410 becomes 4
- C=45=810 becomes 8
- D=1=1010 becomes 10
Final Ratio
Thus, the final ratio is:
A:B:C:D=3:4:8:10
We know:
- A is 34 of B
- B is 12 of C
- C is 45 of D
From the first relationship, we can express A as:
A=34B
This implies:
AB=34
From the second relationship, we can express B as:
B=12C
This implies:
BC=12
From the third relationship, we can express C as:
C=45D
This implies:
CD=45
To find A:B:C:D, we can express all variables in terms of D.
D=54C
B=12C=12(45D)=410D=25D
A=34B=34(25D)=620D=310D
Now we have:
- A=310D
- B=25D
- C=45D
- D=D
A:B:C:D=310D:25D:45D:D
Since D is common in all terms, we can simplify:
A:B:C:D=310:25:45:1
To express these ratios in whole numbers, we can convert them to a common denominator:
- The common denominator for 10,5,5,1 is 10.
- A=310 becomes 3
- B=25=410 becomes 4
- C=45=810 becomes 8
- D=1=1010 becomes 10
Thus, the final ratio is:
A:B:C:D=3:4:8:10
Topper's Solved these Questions
Similar Questions
Knowledge Check
If A:B=2:3,B:C=3:4andC:D=3:5 then value of A:B:C:D is :
A6:9:15:20B2:3:4:5C2:3:5:12D6:9:12:20IfAB=23,BC=45andCD=32, then what is the ratio of A:B:C:D ?
A8 : 12 : 10 : 15B8 : 15 : 12 : 10C8 : 10 : 12 : 15D8 : 12 : 15 : 10If A:B=2:5,B:C=4:3 and C:D=2:1, what is the value of A:C:D?
A6:5:2B7:20:10C8:30:15D16:30:15
If A : B = 1 : 2, B : C = 3 : 4 and C : D = 5 : 6, then find the value of D : C : B.
View SolutionThe ratio of A:B=1:3,B:C=2:5,C:D=2:3. Find the value of A:B:C:D
View SolutionDivide Rs. , 800 amongst A,B,C and D so that A and B receives three times of the part of C and D both and B gets 4 times the share of C and C gets 1−12 times of D' s part , then the share of A is .
View SolutionLet a, b, c, d be positive integers such that logab=3/2andlogcd=5/4. If (a-c) = 9, then find the value of (b-d).
View Solution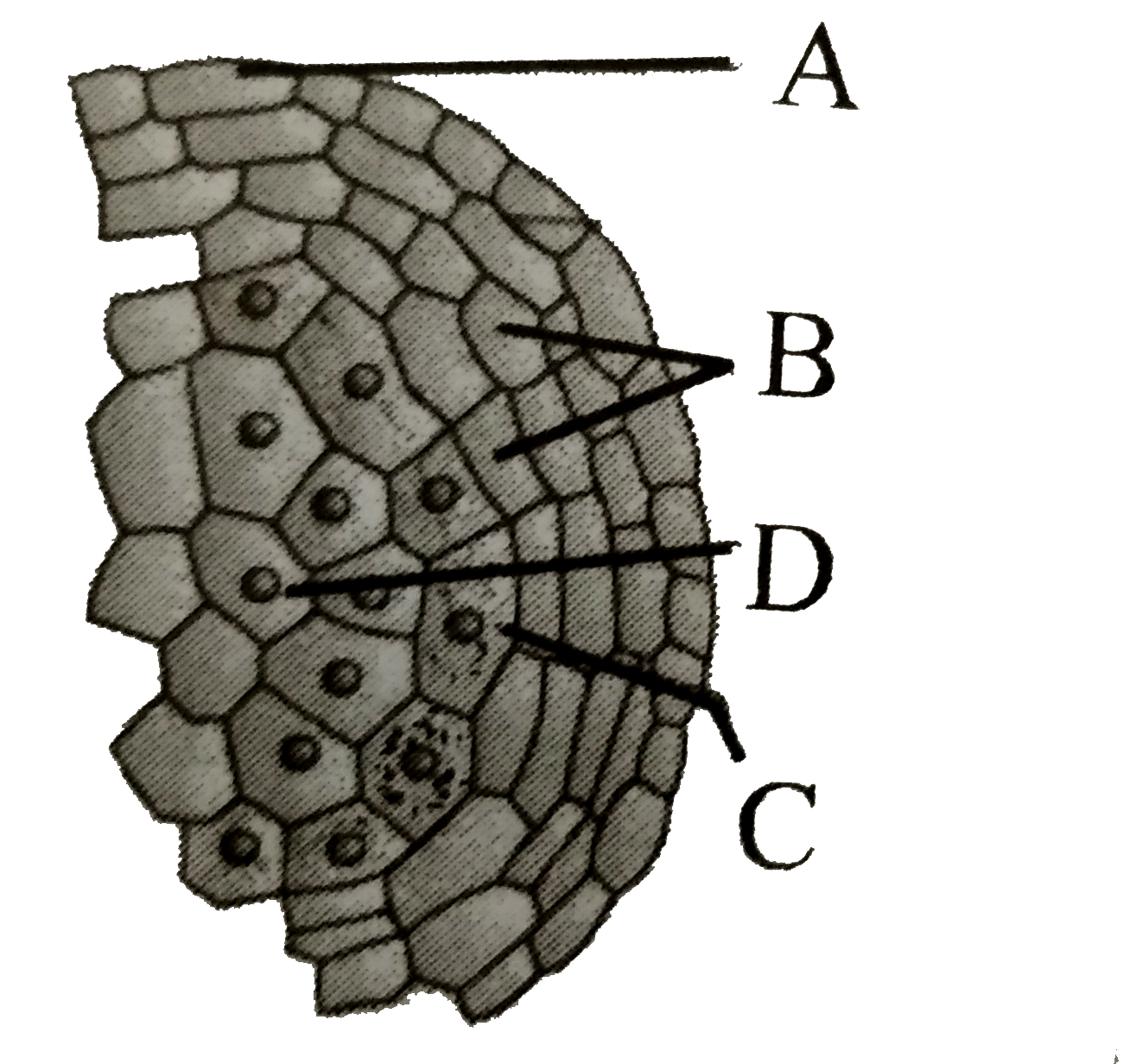
Write down the name of parts A, B, C and D
View Solution
