Two cog wheels having 20 and 25 cogs respectively. The bigger wheel makes 6 rotations per hour. Calculate how many times the specific cog of bigger wheel meet with the specific cog of smaller wheel in 15 hours?
33
23
22
24
The correct Answer is:B
Step by step video, text & image solution for Two cog wheels having 20 and 25 cogs respectively. The bigger wheel makes 6 rotations per hour. Calculate how many times the specific cog of bigger wheel meet with the specific cog of smaller wheel in 15 hours? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The number of teeth in the crank wheel and free wheel of a bicycle, connected by a chain are 48 and 24, respectively. Their diameters are 20 cm and 10 cm, respectively. The radius of the rear wheel to which the free wheel is fixed coaxially is 10 times that of the free wheel. If a cyclist pedals the bicycle at two rotations per second, find the speed of the cyclist.
View SolutionThe angular speed of a motor wheel is increased from 600rpm to 1200rpm in 4 seconds. Calculate how many revolutions does the engine make during this time.
View Solution
Knowledge Check
Two cogged wheels move together. One wheel has 25 cogs & another has 30 cogs. If bigger wheel rotates 75 times per minute, then how many rotations does smaller wheel take in 4 minutes ? (a) 720 (b) 1080 (c) 360 (d) 1200
A720B1080C360D1200A chain couples and rotates two wheels in a bicycle . The radii of bigger and smaller wheels are 0.5 m and 0.1 m respectively . The bigger wheel rotates at the rate of 200 rotations per minute , then the rate of rotation of smaller wheel will be :-
A1000 rpmB50/3 rpmC200 rpmD40 rpmA wheel makes 20 revolutions per hour. The radians turns through 25 minutes is __________.
A50πc7B250πc3C150πc7D50πc3
The wheels of a car are of diameter 80 cm each. How many complete revolutions does each wheel make in 10 minutes when the car is travelling at a speed of 66 km per hour?
View SolutionTwo thin rings of slightly different radii are joined together to make a wheel (see figure) of radius R. There is a very small smooth gap between the two ring. The wheel has a mass M and its centre of mass is at its geometrical centre. The wheel stands on a smooth surface and a small particle of mass m lies at the top (A) in the gap between the rings. The system is released and the particle begins to slide down along the gap. Assume that the ring does not lose contact with the surface.
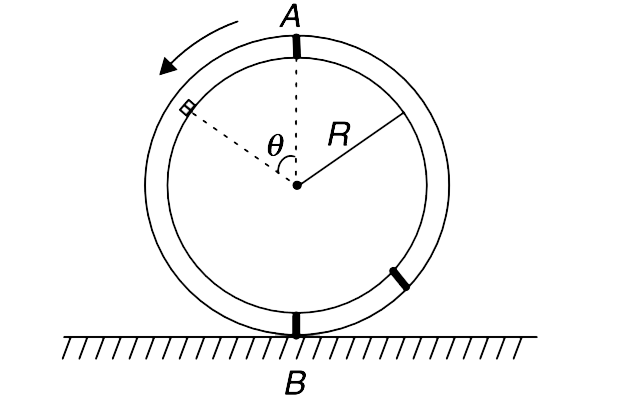
(a) As the particle slides down from top point A to the bottom point B, in which direction does the centre of the wheel move?
(b) Find the speed of centre of the wheel when the particle just reaches the bottom point B. How much force the particle is exerting on the wheel at this instant?
(c) Find the speed of the centre of the wheel at the moment the position vector of the particle with respect to the centre of the wheel makes an angle q with the vertical. Do this calculation assuming that the particle is in contact with the inner ring at desired value of θ
View SolutionA car is moving with a constant speed. The wheels of the car make 120 rotations per minute. The brakes are applied and the car comes to rest in 8 second. How many rotations are completed by the wheels, before the car is brought to rest? (Assume that the brakes produce a constant retarding force)
View SolutionTwo fly wheels A and B are mounted side by side with frictionless bearings on a common shaft. Their moments of inertia about the shaft are 5.0kgm2 and 20.0kgm2 respectively. Wheel A is made to rotate at 10 revolution per second. Wheel B, initially stationary, is now coupled to A with the help of a clutch. The rotation speed of the wheels will become
View SolutionTwo fly wheels A and B are mounted side by side with frictionless bearings on a common shaft. Their moments of inertia about the shaft are 5.0 kg m2 and 20.0 kg m2 respectively. Wheel A is made to rotate at 10 revolution per second. Wheel B, initially stationary, is now coupled to A with the help of a clutch. The rotation speed of the wheels will become
View Solution
