A ball having charge to mass ratio 8 μc/g is placed at a distance of 10 cm from a wall.Suddenly an electric field 100Nm−1 is switched on. Assuming collisions to be elastic.Find Time period of oscillations.
0.5 sec
1 sec
√2 sec
√3 sec
To solve the problem, we need to find the time period of oscillations of a charged ball placed in an electric field. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Given Data
- Charge to mass ratio QM=8μC/g=8×10−6C/10−3kg=8×10−3C/kg
- Electric field E=100N/m
- Distance from the wall s=10cm=0.1m
Step 2: Calculate the Acceleration
The force F acting on the ball due to the electric field is given by:
F=QE
Using Newton's second law, F=ma, we can express the acceleration a as:
a=Fm=QEM
Substituting the charge to mass ratio:
a=QM⋅E=(8×10−3Ckg)⋅(100N/m)
Calculating this gives:
a=8×10−1m/s2=0.8m/s2
Step 3: Use the Equation of Motion
We will use the equation of motion to find the time t it takes for the ball to reach the wall:
s=ut+12at2
Since the initial velocity u=0, the equation simplifies to:
s=12at2
Rearranging gives:
t2=2sa
Substituting s=0.1m and a=0.8m/s2:
t2=2×0.10.8=0.20.8=0.25
Taking the square root:
t=√0.25=0.5s
Step 4: Calculate the Time Period of Oscillation
The time period T of oscillation is twice the time taken to reach the wall (since it will take the same time to come back):
T=2t=2×0.5=1s
Final Answer
The time period of oscillation is T=1s.
---
- Charge to mass ratio QM=8μC/g=8×10−6C/10−3kg=8×10−3C/kg
- Electric field E=100N/m
- Distance from the wall s=10cm=0.1m
The force F acting on the ball due to the electric field is given by:
F=QE
Using Newton's second law, F=ma, we can express the acceleration a as:
a=Fm=QEM
Substituting the charge to mass ratio:
a=QM⋅E=(8×10−3Ckg)⋅(100N/m)
Calculating this gives:
a=8×10−1m/s2=0.8m/s2
We will use the equation of motion to find the time t it takes for the ball to reach the wall:
s=ut+12at2
Since the initial velocity u=0, the equation simplifies to:
s=12at2
Rearranging gives:
t2=2sa
Substituting s=0.1m and a=0.8m/s2:
t2=2×0.10.8=0.20.8=0.25
Taking the square root:
t=√0.25=0.5s
The time period T of oscillation is twice the time taken to reach the wall (since it will take the same time to come back):
T=2t=2×0.5=1s
The time period of oscillation is T=1s.
Topper's Solved these Questions
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-A
80 Videos
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-B
40 Videos
- JEE MAINBOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - All Questions
473 Videos
- JEE MAIN 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question
492 Videos
Similar Questions
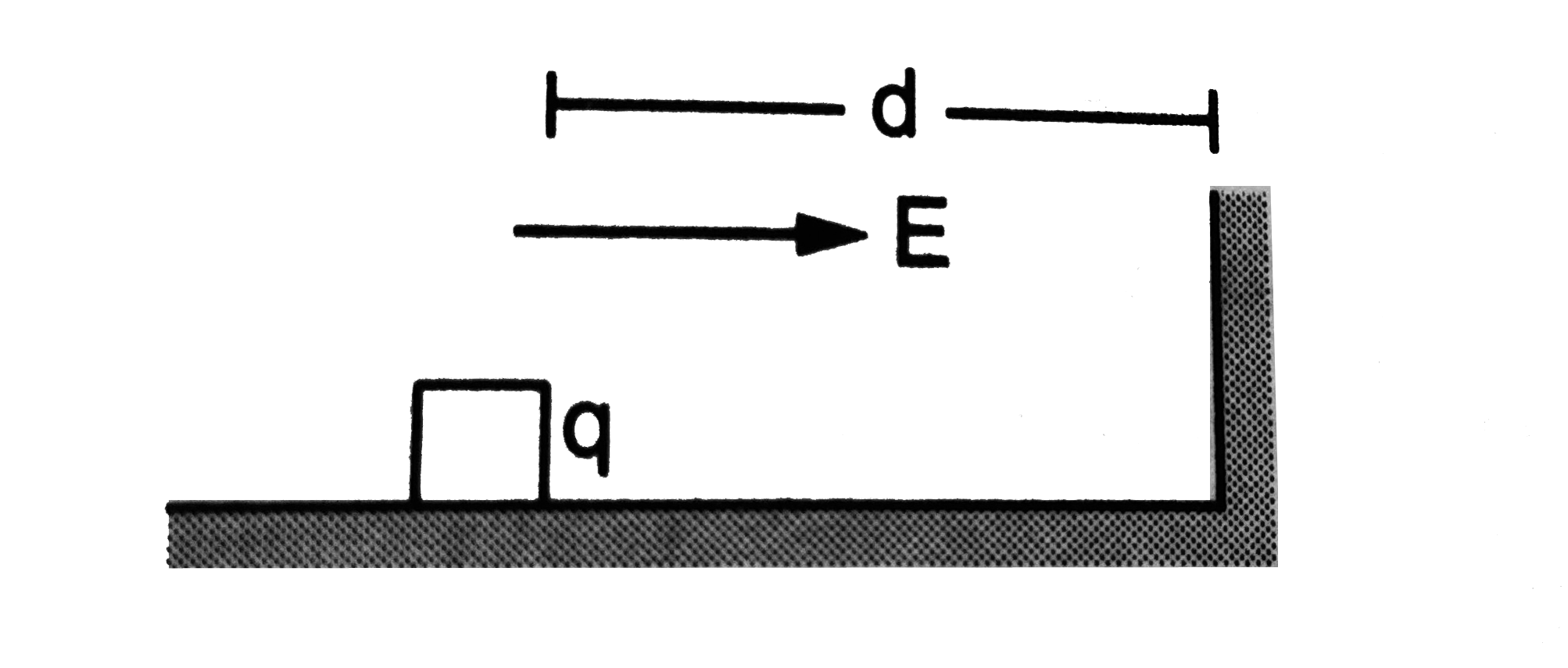
A block of mass n containing a net positive charge q is placed on a smooth horizontal table which terminates in a vertical wall as shown in figure(29-E2). The distance of the bolck from the wall is d. A horizontal electric field E to towards right is switched on. Assuming elastic collisions find the time period of the resulting oscillatory motio. Is it a simple harmonic motion?
View SolutionPositive charge Q is distributed uniformly over a circular ring of radius R. A particle having a mass m and a negative charge q, is placed on its axis at a distance x from the centre. Find the force on the particle. Assuming x ltltR, find the time period of oscillation of the particle if it is released from there.
View Solution
Knowledge Check
A particle of mass 'm' and charge 'q' is suspended from the ceiling with the help of an insulating wire of length 'l'. It is placed in an uniform electric field as shown in figure. Then the time period of oscillation is
_2019_CBT6_E01_041_Q01.png) A ⎷l√g2+(qEm)2B2π ⎷l√g2+(qEm)2C2π ⎷l√g2+(qEm)D2π ⎷l√g2−(qEm)2
A ⎷l√g2+(qEm)2B2π ⎷l√g2+(qEm)2C2π ⎷l√g2+(qEm)D2π ⎷l√g2−(qEm)2A ball A of mass 3m is placed at a distance d from the wall on a smooth horizontal surface Another ball B of mass m moving with velocity u collides with ball A. The coefficient of restitution between the balls and the wall and between the balls is e
(a) the velocity of ball B after collision is u(3e−1)4.
(b) the velocity of ball B after collision is u(2e−1)4.
( c) after collision, ball A will have away by distance d(2e−1)(2e−1) during the time ball B returns back to wall.
(d) after collision, ball A will move away by distance d(e−1)(3e−1) during the time ball B returns back to wall.Aa,dBa,cCb,dDc,dAn electric dipole cosnists of two opposite charges of magnitude 1μC separated by a distance of 2cm.The dipole is placed in an electric filled 10−5Vm−1.The maximum torque that the field exert on the dipole is
A10−3NmB2×10−13NmC3×10−3NmD4×10−3Nm
A ball having charge q and mass m is suspended from a string of length L between two parallel plates where a vertical electric field E is established . Find the time period of simple pendulum if electric field is directed (a) downward and (b) upward.
View SolutionA body having specific charge 8μC/g is resting on a frictionless plane at a distance 10 cm from the wall (as shown in the figure). It starts moving towards the wall when a uniform electric field of 100 V/m is applied horizontally towards the wall. If the collision of the body with the wall is perfectly elastic, then the time period of the motion will be ______ s.

View Solutionfind the electric field strength due to a point charge of 5μC at a distance of 80 cm from the charge.
View SolutionA block having mass 'm' ad carge q is resting on a fractionless plane at distance L from the wall as shown in fig.Discuss the motion of the block when a uniform electric field E is applied horizontally towards the wall assuming that collision of the block with the wall is perfectly elastic.
View SolutionA block having mass m and charge Q is resting on a frictionless plane at a distance d from fixed large non-conducting infinite sheet of uniform charge density −σ as shown in Figure. Assuming that collision of the block with the sheet is perfectly elastic, find the time period of oscillatory motion of the block. Is it SHM ?
View Solution
