PQ and PR are two infinite rays, QAR is an arc. Point lying in the shaded region excluding the boundary satisfies

$| z - 1 | > 2 : | a r g (z - 1) | < \frac{π}{4}$

$| z - 1 | > 2 : | a r g (z - 1) | < \frac{π}{2}$

$| z + 1 | > 2 : | a r g (z + 1) | < \frac{π}{4}$

$| z + 1 | > 2 : | a r g (z + 1) | < \frac{π}{2}$

Text Solution

Verified by Experts

The correct Answer is:C

Updated on:21/07/2023

P is any point inside or on the boundary of $Δ A B C$ having perimeter p and area $Δ$ R is any point in the plane of $Δ A B C$ such that PR $\leq$ 5. the area of the region in which the point R lies is
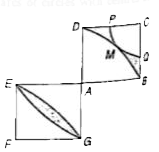
A $25 π + 5 p + Δ$
B $5 π + 25 P + Δ$
C $5 π + 5 P + Δ$
D $5 (π + p) + 25 Δ$
ABCD and EFGA are the squares of side 4 cm , each . In square ABCD, DMB and PMQ are the arcs of circles with centres at A and C respectively . In square AEFG , the shaded region is enclosed by two arcs of circles with centres at A and F , respectively . What is the ratio of the shaded regions of the squares ABCD and AEFG respectively .

A $\frac{2 + π (\sqrt{2} - 2)}{(π - 2)}$
B $\frac{(π - 2)}{2 (\sqrt{2} + 1 - π)}$
C $\frac{4}{3}$
Dnone of these