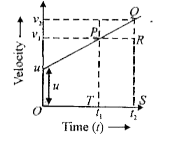
Show that the slope of velocity-time graph for uniformly acceleratied motion is equal to the acceleration of the body.Solution in Punjabi
In uniform accelerated motion, the valocity of the body increases at a constant rate. Therefore, v - t graph is a straight line inclined to the time axis with a postiive slope.
Change in veloity, when body moves from P to Q =v2−v1.
Time taken for change =t2−t1
Therefore, acceleration =v2−v1t2−t1=QRPR= slope of line PQ =tanθ
Thus, acceleration of uniformly accelerated motion of the body is equal to the slope of v−t graph.
Similar Questions
Represent the velocity-time graph for a uniformly accelerated motion when acceleration is positive.
View SolutionThe velocity - time graph of a body is given in figure below .
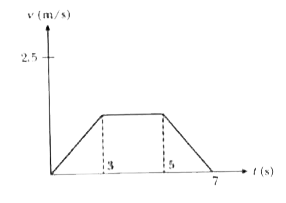
The acceleration-time graph of the motion of the body is
View SolutionDraw the velocity-time graph for uniformly accelerated motion. Show that slope of the velocity-time graph gives acceleration of the object.
View SolutionDerive the following equations of motion for uniformly accelerated motion from velocity-time graph : s = ut + 1/2 at^(2)
View SolutionDerive the following equations of motion for uniformly accelerated motion from velocity-time graph : v2−u2=2as
View SolutionAsserion : The acceleration of an object at a particular time is the slope of the velocity-time graph at that instant of time.
Reason : For uniform motion acceleration is zero.
View SolutionWhat will be the shape of velocity time graph for uniformly accelerated body ?
View SolutionFor a uniformly retarded motion, the velocity-time graph is :
View Solution
