The density of liquid mercury is 13.6g/cm3. How many moles of mercury are there in 1 litre of the metal? (Atomic mass of Hg=200).
The correct Answer is:68
To solve the problem, we need to determine how many moles of mercury are present in 1 liter of the metal, given its density and atomic mass. Here’s a step-by-step solution:
Step 1: Understand the given data
- Density of mercury (Hg) = 13.6 g/cm³
- Volume of mercury = 1 liter = 1000 mL
- Atomic mass of mercury (Hg) = 200 g/mol
Step 2: Convert volume from liters to cubic centimeters
1 liter is equivalent to 1000 mL, and since 1 mL is equal to 1 cm³, we have:
1 L=1000 cm3
Step 3: Calculate the mass of mercury in 1 liter
Using the density formula:
Density=MassVolume
We can rearrange this to find mass:
Mass=Density×Volume
Substituting the values:
Mass=13.6 g/cm3×1000 cm3=13600 g
Step 4: Calculate the number of moles of mercury
Using the formula for moles:
Moles=MassMolar Mass
Substituting the values:
Moles=13600 g200 g/mol=68 moles
Conclusion
The number of moles of mercury in 1 liter of the metal is 68 moles.
---
- Density of mercury (Hg) = 13.6 g/cm³
- Volume of mercury = 1 liter = 1000 mL
- Atomic mass of mercury (Hg) = 200 g/mol
1 liter is equivalent to 1000 mL, and since 1 mL is equal to 1 cm³, we have:
1 L=1000 cm3
Using the density formula:
Density=MassVolume
We can rearrange this to find mass:
Mass=Density×Volume
Substituting the values:
Mass=13.6 g/cm3×1000 cm3=13600 g
Using the formula for moles:
Moles=MassMolar Mass
Substituting the values:
Moles=13600 g200 g/mol=68 moles
The number of moles of mercury in 1 liter of the metal is 68 moles.
Topper's Solved these Questions
- MOLE CONCEPTBOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - Exercise - 04[B]
27 Videos
- MOLE CONCEPTBOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - Exercise - 05(A)
21 Videos
- MOLE CONCEPTBOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - Comprehension # 9
4 Videos
- IUPAC NOMENCLATUREBOOK - ALLENCHAPTER - IUPAC NOMENCLATUREEXERCISE - Exercise - 05(B)
7 Videos
- QUANTUM NUMBER & PERIODIC TABLEBOOK - ALLENCHAPTER - QUANTUM NUMBER & PERIODIC TABLEEXERCISE - J-ADVANCED EXERCISE
24 Videos
Similar Questions
Knowledge Check
The density of mercury is 13.6gmL−1. Calculate the approximate diameter of an atom of mercury assuming that each atom is occupying a cube of edge length equal to the diameter of the mercury atom.
A3.01 ÅB2.54 ÅC0.29 ÅD2.91 Å
Water rises to a height of 10cm in a capillary tube and mercury falls to a depth of 3.42cm in the same capillary tube. If the density of mercury is 13.6g/c.c. and the angles of contact for mercury and for water are 135∘ and 0∘, respectively, the ratio of surface tension for water and mercury is
View SolutionIf the density of wood is 0.6 g/cm3 , what is the mass of 1cm3 ?
View SolutionCinnabar (HgS) is a prominent ore of mercury. How many grams of mercury are present in 225g of pure HgS? Molar mass of Hg and S are 200.6 g mol−1 and 32gmol−1 respectively.
View SolutionA U -tube in which the cross - sectional area of the limb on the left is one quarter, the limb on the right contains mercury (density13.6g/cm3). The level of mercury in the narrow limb is at a distance of 36 cm from the upper end of the tube. What will be the rise in the level of mercury in the right limb if the left limb is filled to the top with water ?
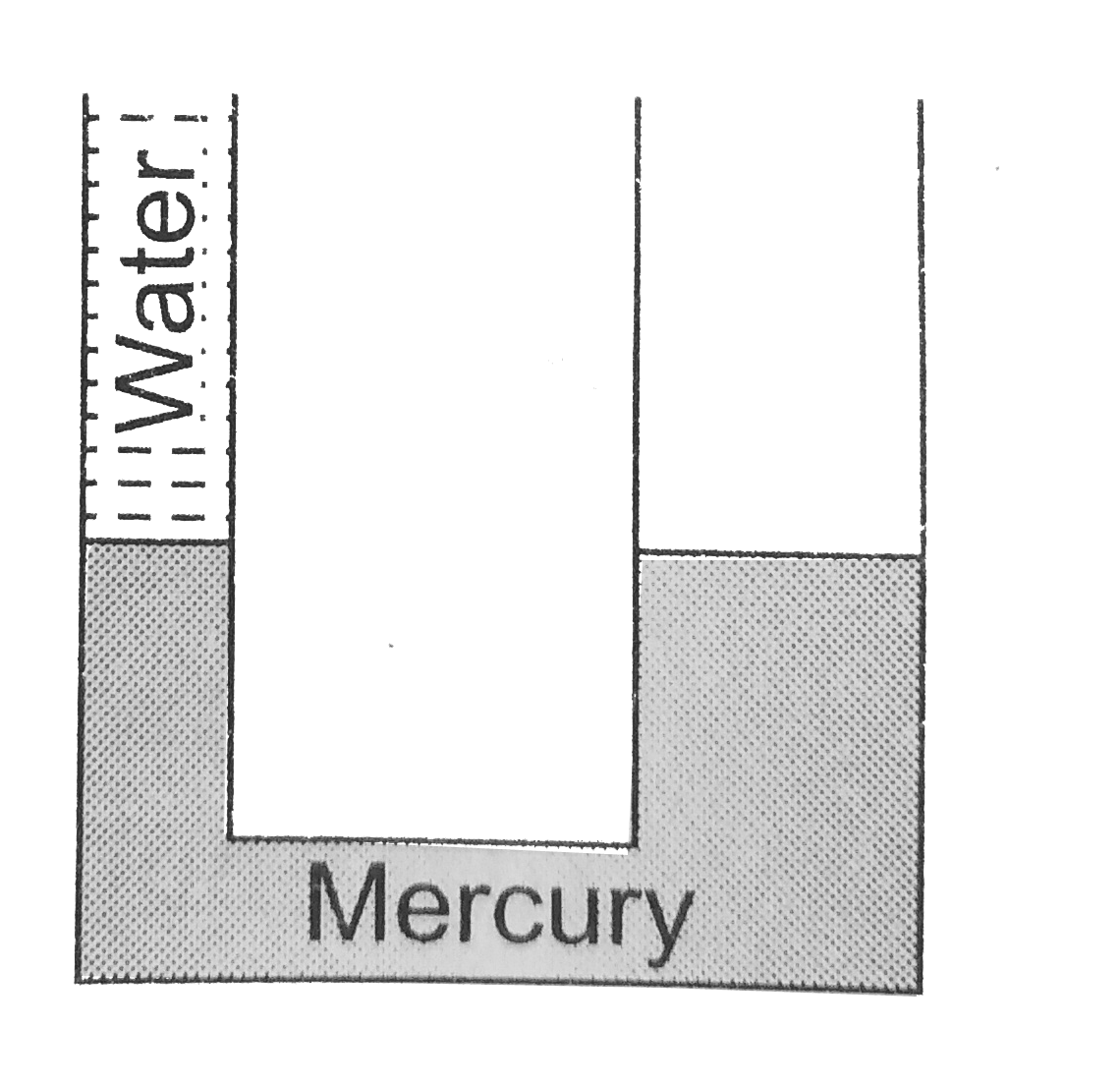
View SolutionA U-tube in which the cross-sectional area of the limb on the left is one-third of the limb on the right contains mercury (density 13.6g/cm3). The level of mercury in the narrow limb extends to a distance of 30cm from the upper end of the tube. What will be the rise in the level of mercury in the right limb, if the left limb is filled to the top with water (neglect surface tension effects)?

View SolutionA sphere is made of an alloy of Metal A (density 8g/cm3) and Metal B (density 6g/cm3). The sphere floats in mercury (density 13.6g/cm3) with half its volume submerged. The percentage of the total volume of the sphere that is occupied by metal A is ___________ .
View SolutionThe density of silver is 10gcm−3. Find the mass of a silver block of volume 200cm3 .
View Solution