Frequancy , Time period = T, Energy of orbit , radius of orbit , Atomic number = Z, Orbit number = n :
The correct Answer is:
To solve the matching question, we will analyze the relationships between the terms in Column I and Column II based on the principles of atomic structure.
Step 1: Analyze Frequency (f)
The frequency f is related to the time period T and can be expressed as:
f∝1T
From the relationship of time period, we know:
T∝n3Z2
Thus, substituting this into the frequency equation:
f∝Z2n3
This means that frequency f is proportional to Z2 and inversely proportional to n3. Therefore, we can match:
- f with Z2 (option Q).
Step 2: Analyze Time Period (T)
From the previous analysis, we have:
T∝n3Z2
This indicates that time period T is directly proportional to n3. Therefore, we can match:
- T with n3 (option P).
Step 3: Analyze Energy of nth Orbit (E_n)
The energy En of the nth orbit is given by:
En∝Z2n2
This means that energy En is proportional to Z2 and inversely proportional to n2. Therefore, we can match:
- En with Z2 (option Q).
Step 4: Analyze Radius of nth Orbit (r_n)
The radius rn of the nth orbit is given by:
rn∝n2Z
Taking the reciprocal gives:
1rn∝Zn2
This means that 1rn is proportional to Z and inversely proportional to n2. Therefore, we can match:
- 1rn with Z (option S).
Step 5: Final Matching
Now, let's summarize the matches:
- f matches with Z2 (Q)
- T matches with n3 (P)
- En matches with Z2 (Q)
- 1rn matches with Z (S)
Final Answers:
- A -> Q
- B -> P
- E -> Q
- D -> S
The frequency f is related to the time period T and can be expressed as:
f∝1T
From the relationship of time period, we know:
T∝n3Z2
Thus, substituting this into the frequency equation:
f∝Z2n3
This means that frequency f is proportional to Z2 and inversely proportional to n3. Therefore, we can match:
- f with Z2 (option Q).
From the previous analysis, we have:
T∝n3Z2
This indicates that time period T is directly proportional to n3. Therefore, we can match:
- T with n3 (option P).
The energy En of the nth orbit is given by:
En∝Z2n2
This means that energy En is proportional to Z2 and inversely proportional to n2. Therefore, we can match:
- En with Z2 (option Q).
The radius rn of the nth orbit is given by:
rn∝n2Z
Taking the reciprocal gives:
1rn∝Zn2
This means that 1rn is proportional to Z and inversely proportional to n2. Therefore, we can match:
- 1rn with Z (option S).
Now, let's summarize the matches:
- f matches with Z2 (Q)
- T matches with n3 (P)
- En matches with Z2 (Q)
- 1rn matches with Z (S)
- A -> Q
- B -> P
- E -> Q
- D -> S
Topper's Solved these Questions
- ATOMIC STRUCTUREBOOK - ALLENCHAPTER - ATOMIC STRUCTUREEXERCISE - Exercise - 04[A]
49 Videos
- ATOMIC STRUCTUREBOOK - ALLENCHAPTER - ATOMIC STRUCTUREEXERCISE - Exercise - 04[B]
10 Videos
- ATOMIC STRUCTUREBOOK - ALLENCHAPTER - ATOMIC STRUCTUREEXERCISE - Exercise - 02
59 Videos
- IUPAC NOMENCLATUREBOOK - ALLENCHAPTER - IUPAC NOMENCLATUREEXERCISE - Exercise - 05(B)
7 Videos
Similar Questions
Knowledge Check
The radius of nth orbit rn in the terms of Bohr radius (a0) for a hydrogen atom is given by the relation
Ana0B√na0Cn2a0Dn3a0In a hydrogen atom, the radius of nth bohr orbit is rn. The graph between log (rn/r1) and logn will be
A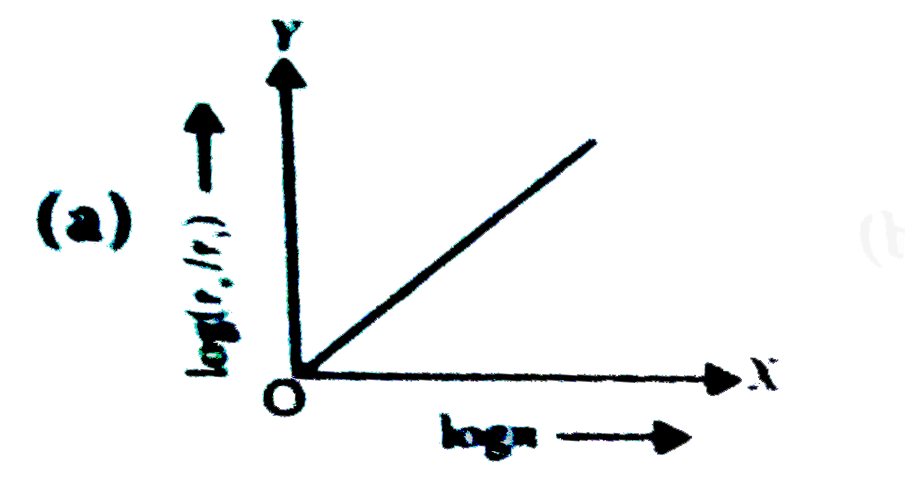 B
B C
C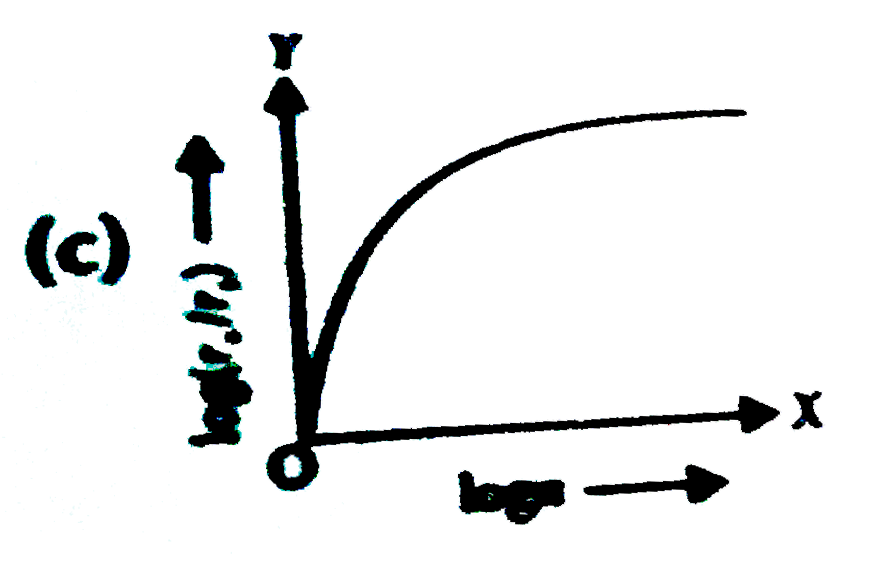 D
D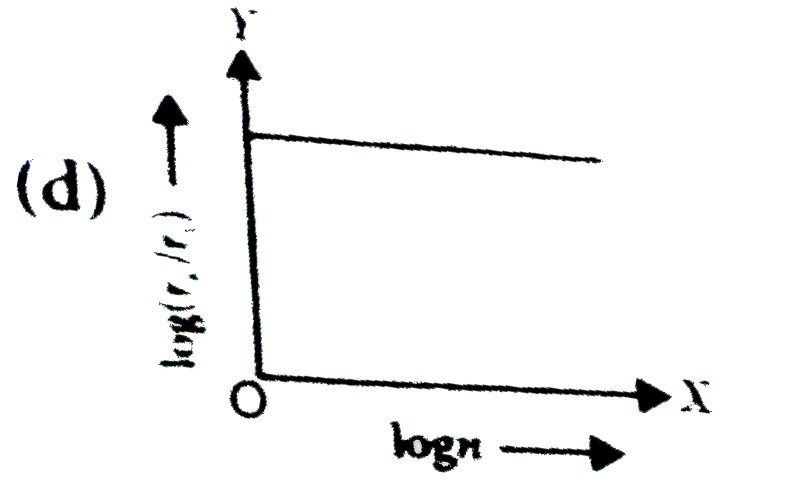
Radius (rn) of electron in nth orbit versus atomic number (Z) graph is
View SolutionIn Bohr's model, for aunielectronic atom, following symbols are used
rnz→ Potential energy n+th orbit with atomic number Z,
Un,z rarr Potantial energy of election , Kn,z→ Kinetic energy of electron ,
Vn,z→ Volocity of electron , Tn,z→ Time period of revolution
ColumnIColumnII(A)U1,2:K1,1(P)1:8(B)r2,1:r1,2(Q)−8:1(C)V1,3:V3,1(R)9:1(D)T1,2:T2,2(S)8:1
View SolutionCalculate the ratio of the radius of 1st orbit of H atom to that of 4thorbit.
Hint :rn∝(n)2
View SolutionLet Rt represents activity of a sample at an insant and Nt represent number of active nuclei in the sample at the instant. T1/2 represents the half life.
Column IColumn II(A)t=T1/2(p)Rt=R02(B)t=T1/2ln2(q)N0−Nt=N02(C)t=32T1/2(r)Rt−R0R0=1−ee(s)Nt=N02√2
View SolutionCalculate the ratio of the radius of 1st orbit of H atom to thatof4thorbit.
Hint :rn∝(n)2
View SolutionTime period of revolution of an electron in nth orbit in a hydrogen like atom is given by T=T0naZb,Z=atomic number
View Solution
