The electrochemical cell shown below is a concentration cell. M∣M2+( saturated solution of sparingly soluble salt, MX2)∣∣∣M2+(0.001moldm−3)∣∣M
The emf of the cell depends on the difference in the concentration of M2+ ions at the two electrodes. The emf of the cell at 298 is 0.059V.
The solubility product (Ksp,mol3dm−9) of MX2 at 298 based on the information available the given concentration cell is ( Take 2.303×R×298/F=0.059V)
1×10−15
4×10−15
1×10−12
1×10−12
The correct Answer is:B
To solve the problem of finding the solubility product (Ksp) of the sparingly soluble salt MX₂, we can follow these steps:
Step 1: Understand the Dissociation of MX₂
When the salt MX₂ dissolves in water, it dissociates into its ions:
MX2(s)⇌M2+(aq)+2X−(aq)
Let the solubility of MX₂ be s. Therefore, the concentration of M2+ ions will be s and the concentration of X− ions will be 2s.
Step 2: Write the Expression for Ksp
The solubility product (Ksp) can be expressed as:
Ksp=(M2+)(X−)2
Substituting the concentrations:
Ksp=(s)(2s)2=4s3
Step 3: Use the Nernst Equation
The Nernst equation for the concentration cell is given by:
Ecell=E∘cell−2.303RTnFlog((M2+)anode(M2+)cathode)
In this case, since it is a concentration cell, E∘cell=0.
Step 4: Substitute Known Values
Given:
- Ecell=0.059V
- (M2+)cathode=0.001mol/dm3
- n=2 (since 2 electrons are involved)
Now substituting into the Nernst equation:
0.059=0−0.0592log((M2+)anode0.001)
Step 5: Solve for the Concentration of M2+ at the Anode
Rearranging the equation:
0.059=−0.0295log((M2+)anode0.001)
Dividing both sides by -0.0295:
−2=log((M2+)anode0.001)
Taking the antilog:
(M2+)anode0.001=10−2
(M2+)anode=0.001×10−2=0.00001mol/dm3=10−5mol/dm3
Step 6: Calculate Ksp
Now substituting s=10−5 into the Ksp expression:
Ksp=4s3=4(10−5)3=4×10−15mol3/dm9
Final Answer
Thus, the solubility product Ksp of MX₂ at 298 K is:
Ksp=4×10−15mol3/dm9
Conclusion
The correct option is B: 4×10−15mol3/dm9.
---
When the salt MX₂ dissolves in water, it dissociates into its ions:
MX2(s)⇌M2+(aq)+2X−(aq)
Let the solubility of MX₂ be s. Therefore, the concentration of M2+ ions will be s and the concentration of X− ions will be 2s.
The solubility product (Ksp) can be expressed as:
Ksp=(M2+)(X−)2
Substituting the concentrations:
Ksp=(s)(2s)2=4s3
The Nernst equation for the concentration cell is given by:
Ecell=E∘cell−2.303RTnFlog((M2+)anode(M2+)cathode)
In this case, since it is a concentration cell, E∘cell=0.
Given:
- Ecell=0.059V
- (M2+)cathode=0.001mol/dm3
- n=2 (since 2 electrons are involved)
0.059=0−0.0592log((M2+)anode0.001)
Rearranging the equation:
0.059=−0.0295log((M2+)anode0.001)
−2=log((M2+)anode0.001)
(M2+)anode0.001=10−2
(M2+)anode=0.001×10−2=0.00001mol/dm3=10−5mol/dm3
Now substituting s=10−5 into the Ksp expression:
Ksp=4s3=4(10−5)3=4×10−15mol3/dm9
Thus, the solubility product Ksp of MX₂ at 298 K is:
Ksp=4×10−15mol3/dm9
The correct option is B: 4×10−15mol3/dm9.
Topper's Solved these Questions
Similar Questions
Consider the cell Zn∣∣Zn2+∣∣∣∣Cu2+∣∣Cu. If the concentration of Zn and Cu ions are doubled, the emf of the cell.
View SolutionThe e.m.f of cell Ag∣∣AgI(s),0.05MKI∣∣|0.05MAgNO3|Ag is 0.788V. Calculate solubility product of AgI.
View SolutionFind the EMF of the concentration cell represented as given below:
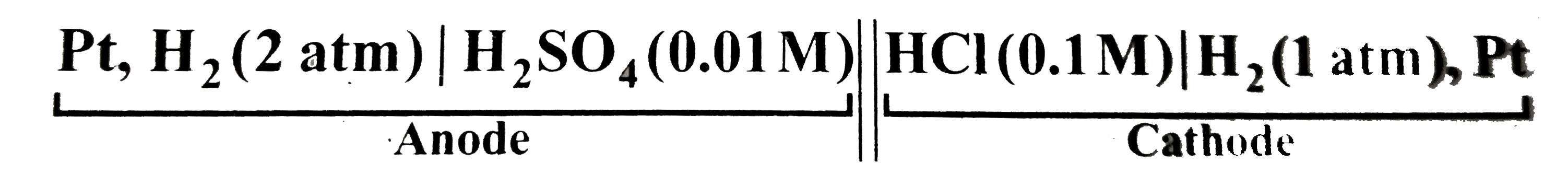View SolutionGiven concentration cell Zn/Zn2+(1M)/Zn2+(0.15M)/Z
Calculate E cell. As the cell discharges, does the difference in concentrations between the two solutions become smaller or larger?View SolutionThree sparigly soluble salts M2X,MX,and MX3 have the same solubility product. Their solubilities will be in the order
View SolutionIf the solubility product Ksp of a sparingly soluble salt MX2 at 25∘C is 1.0×10−11 , then the solubility of the salt in mole litre−1 at this temperature will be
View SolutionA cell contains two hydrogen electrodes. The negative electrode is in contact with a solution of 10−6M hydrogen ion. The EMF of the cell is 0.118 V at 298 K. The concentration of H+ ion at the positive electrode is 10−x, The value of 'x' is
View SolutionA3B2 is a sparingly soluble salt with molar mass M(gmol−1) and solubility x gm litre−1, the ratio of the molar concentration of B3− to the solubilty product of the salt is :−
View SolutionStatement-I: In the Daniel cell, if concentration of Cu2+ and Zn2+ ions are doubled the emf of the cell will not change.
Because Statement-II: If the concentration of ions in contact with the metals is doubled, the electrode potential is doubled.View SolutionA cell contains two hydrogen electrodes. The negative electrode is in contact with a solution of 10−6M hydrogen ions. The EMF of the cell is 0.118V at 25∘C. Calculate the concentration of hydrogen ions at the positive electrode.
View Solution