Question

There is a hole in the middle of a small thin circular converging lens of focal length f=4 cm. The diameter of the hole is half the aperture diameter of lens. There is a point like light source $A=9$ cm from a wall. Where should the lens be placed from object (in cm) in order to het a single. circular illuminated spot on the wall which also has a sharp edge?

Answer

6

Explanation

The light cone that passes the circular hole and the light cone that is formed from the beam refracted by the rest of the lens must meet exactly at the wall. Let us denote the radius of the bright circle formed on the wall with h, the radius of the hole on the lens with r and the radius of the lnes with `R=2r`. The distance between the light- source and the lens is the object distance O.

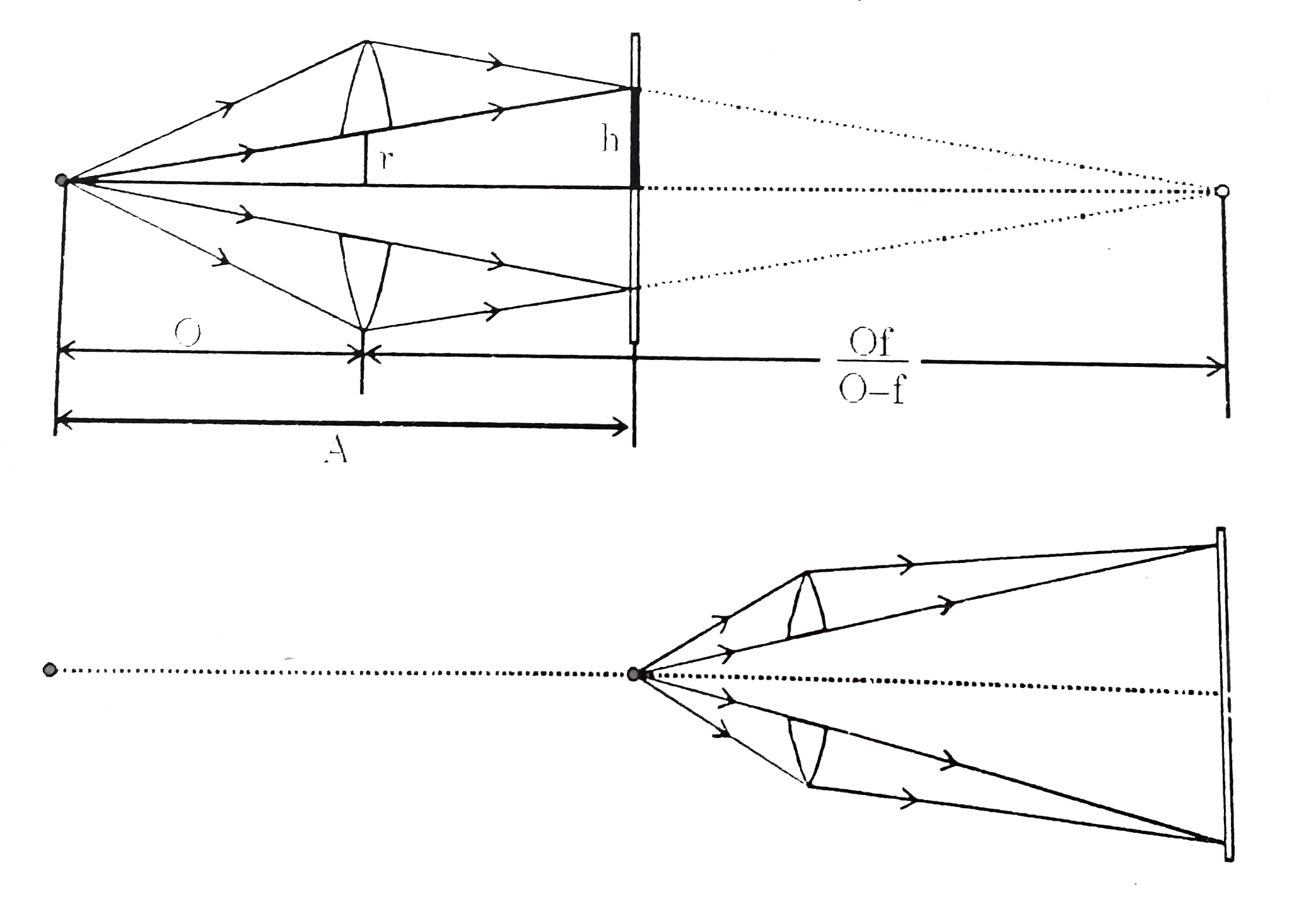
The distance between the lens and the image of the light-source is `i=(Of)/(O-f)`
From the dimilar traingles :
`(h)/(r)=(A)/(O)`
and `(h)/(R)=(h)/(2r)=(O+i-A)/(i)=(O+(Of)/(O-f)-A)/((Of)/(O-f))`
from the first equation `(h)/(r)` can be substituted and the following quadratic equation can be gained for O:
`2O^(2)-2AO+af=0`
Its solution are: `O =(A)/(2)pm (1)/(2) sqrt(A(A-2f))` ltb rgt In our case the following two solution are gained for the possible of the light-gained: `O_(1)=6cm` and `O_(2)=3cm`. In the first case, the lens must be placed `6cm` from the light source. The second solution is also a correct solution, though in this case the image is virtual `("A trivial solution is the" O=0)` The problem can only be solved if A gt 2f.