Path traced by a moving particle in space is called trajectory of the particle. Shape of trajectiry is decided by the forces acting on the particle. When a coordinate system is associated with a particle motion, the curve equation in which the particle moves [y=f(x)] is called equation of trajectory. It is just giving us the relation among x and y coordinates of the particle i.e. the locus of particle. To find equation of trajectory of a particle, find first x and y coordinates of the particle as a function of time eliminate the time factor.
The position vector of car w.r.t. its starting point is given as →r=atˆi−bt2ˆj where a and b are positive constants. The locus of a particle is:-
a2y+bx2=0
a2y=bx2
y=ba2
ay2=b2x
The correct Answer is:A
To find the equation of the trajectory of the particle given its position vector, we will follow these steps:
Step 1: Identify the position vector components
The position vector of the car with respect to its starting point is given as:
→r=at^i−bt2^j
From this, we can identify the x and y coordinates:
- x=at
- y=−bt2
Step 2: Express time in terms of x
From the equation for x, we can express time t in terms of x:
t=xa
Step 3: Substitute time into the y equation
Now, we will substitute the expression for t into the equation for y:
y=−bt2
Substituting t=xa:
y=−b(xa)2
This simplifies to:
y=−ba2x2
Step 4: Rearranging the equation
To express this in a standard form, we can rearrange it:
a2y=−bx2
or equivalently:
a2y+bx2=0
Conclusion
Thus, the locus of the particle is given by the equation:
a2y+bx2=0
The position vector of the car with respect to its starting point is given as:
→r=at^i−bt2^j
From this, we can identify the x and y coordinates:
- x=at
- y=−bt2
From the equation for x, we can express time t in terms of x:
t=xa
Now, we will substitute the expression for t into the equation for y:
y=−bt2
Substituting t=xa:
y=−b(xa)2
This simplifies to:
y=−ba2x2
To express this in a standard form, we can rearrange it:
a2y=−bx2
or equivalently:
a2y+bx2=0
Thus, the locus of the particle is given by the equation:
a2y+bx2=0
Topper's Solved these Questions
- MISCELLANEOUSBOOK - ALLENCHAPTER - MISCELLANEOUSEXERCISE - Comprehension 4
6 Videos
- MISCELLANEOUSBOOK - ALLENCHAPTER - MISCELLANEOUSEXERCISE - DATA SUFFICIENCY QUESTIONS
3 Videos
- MISCELLANEOUSBOOK - ALLENCHAPTER - MISCELLANEOUSEXERCISE - Comprehension 2
8 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLENCHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-IV
8 Videos
Similar Questions
Path traced by a moving particle in space is called trajectory of the particle. Shape of trajectiry is decided by the forces acting on the particle. When a coordinate system is associated with a particle motion, the curve equation in which the particle moves [y=f(x)] is called equation of trajectory. It is just giving us the relation among x and y coordinates of the particle i.e. the locus of particle. To find equation of trajectory of a particle, find first x and y coordinates of the particle as a function of time eliminate the time factor.
In above the velocity (i.e.d→rdt) at t=0 is, if r =at i ^ −bt 2 j ^ :-
View SolutionPath traced by a moving particle in space is called trajectory of the particle. Shape of trajectiry is decided by the forces acting on the particle. When a coordinate system is associated with a particle motion, the curve equation in which the particle moves [y=f(x)] is called equation of trajectory. It is just giving us the relation among x and y coordinates of the particle i.e. the locus of particle. To find equation of trajectory of a particle, find first x and y coordinates of the particle as a function of time eliminate the time factor.
In above question initial acceleration (i.e.d2→rdt2) of particle is, if r =at i ^ −bt 2 j ^:-
View SolutionA particle moves along the curve y=x2+2x. At what point(s) on the curve are the x and y coordinates of the particle changing at the same rate?
View SolutionA particle moves along the curve y=x2+2x. At what point(s) on the curve are the x and y coordinates of the particle changing at the same rate?
View SolutionConsider the motion of a particle described by x=acost,y=asintandz=t . The trajectory traced by the particle as a function of time is
View SolutionThe x and y coordinates of the center of mass of the three-particle system shown below are :
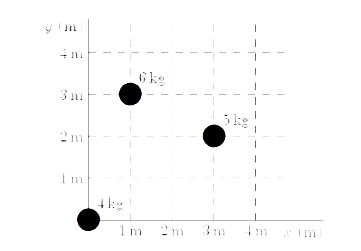
View SolutionThe coordinate of a moving particle at any instant of time t are x = at and y=bt2. The trajectory of the particle is
View SolutionThe momentum of a moving particle given by p=tlnt. Net force acting on this particle is defined by equation F=dpdt. The net force acting on the particle is zero at time
View SolutionThe position vector of a particle moving in x−y plane is given by
→r=(Asinωt)ˆi+(Acosωt)ˆj then motion of the particle is :
View SolutionA xandy co-ordinates of a particle are x=Asin(ωt)andy=Asin(ωt+π/2). Then, the motion of the particle is

View Solution
