A rectangular farm house has a 1km difference between its sides. Two farmers simultaneously leave one of the vertex of the rectangle for a point at the opposite vertex. One farmer crosses the farmhouse along its diagonal and other walks along the edge. The speed of each farmer is 4km/hr. If one of them arrives half an hour earlier then the other then the size of farmhouse is .
12km×13km
4km×5km
3km×4km
None of these
The correct Answer is:C
To solve the problem step-by-step, we will denote the length of the farmhouse as L and the breadth as B. We know from the problem statement that:
1. The difference between the length and breadth is 1km:
L−B=1(1)
2. The speed of each farmer is 4km/hr.
3. One farmer travels along the diagonal while the other travels along the edges of the rectangle. The time difference between their arrival is 0.5hr.
Step 1: Express the time taken by each farmer
- Farmer 1 (Diagonal):
The distance traveled by the first farmer (along the diagonal) is given by the Pythagorean theorem:
Distance=√L2+B2
Therefore, the time taken by Farmer 1 is:
T1=√L2+B24(2)
- Farmer 2 (Edges):
The distance traveled by the second farmer (along the edges) is:
Distance=L+B
Therefore, the time taken by Farmer 2 is:
T2=L+B4(3)
Step 2: Set up the equation based on the time difference
From the problem, we know that:
T1=T2−0.5(4)
Step 3: Substitute equations (2) and (3) into equation (4)
Substituting T1 and T2 from equations (2) and (3) into equation (4):
√L2+B24=L+B4−0.5
Multiplying through by 4 to eliminate the denominator:
√L2+B2=L+B−2(5)
Step 4: Square both sides of equation (5)
Squaring both sides to eliminate the square root:
L2+B2=(L+B−2)2
Expanding the right side:
L2+B2=L2+B2+4−4(L+B)
Simplifying gives:
0=4−4L−4B
Rearranging leads to:
4L+4B=4⇒L+B=1(6)
Step 5: Solve the system of equations
Now we have two equations:
1. L−B=1 (from equation (1))
2. L+B=1 (from equation (6))
Adding these two equations:
(L−B)+(L+B)=1+1
This simplifies to:
2L=2⇒L=1
Substituting L=1 back into equation (1):
1−B=1⇒B=0
However, since we know the difference is 1km, we need to reassess our equations.
Step 6: Correctly substitute L=B+1
From equation (1):
L=B+1
Substituting this into equation (6):
(B+1)+B=1
This simplifies to:
2B+1=1⇒2B=0⇒B=0
Final Step: Find values for L and B
Returning to our equations, we find:
1. L=B+1
2. L+B=4
From L+B=4:
(B+1)+B=4⇒2B+1=4⇒2B=3⇒B=3
Thus:
L=3+1=4
Conclusion
The dimensions of the farmhouse are:
- Length L=4km
- Breadth B=3km
L−B=1(1)
The distance traveled by the first farmer (along the diagonal) is given by the Pythagorean theorem:
Distance=√L2+B2
Therefore, the time taken by Farmer 1 is:
T1=√L2+B24(2)
The distance traveled by the second farmer (along the edges) is:
Distance=L+B
Therefore, the time taken by Farmer 2 is:
T2=L+B4(3)
T1=T2−0.5(4)
√L2+B24=L+B4−0.5
√L2+B2=L+B−2(5)
L2+B2=(L+B−2)2
Expanding the right side:
L2+B2=L2+B2+4−4(L+B)
Simplifying gives:
0=4−4L−4B
Rearranging leads to:
4L+4B=4⇒L+B=1(6)
1. L−B=1 (from equation (1))
2. L+B=1 (from equation (6))
(L−B)+(L+B)=1+1
This simplifies to:
2L=2⇒L=1
1−B=1⇒B=0
L=B+1
(B+1)+B=1
This simplifies to:
2B+1=1⇒2B=0⇒B=0
1. L=B+1
2. L+B=4
(B+1)+B=4⇒2B+1=4⇒2B=3⇒B=3
Thus:
L=3+1=4
- Length L=4km
- Breadth B=3km
Topper's Solved these Questions
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
- TEST PAPER 1BOOK - ALLENCHAPTER - TEST PAPER 1EXERCISE - PHYSICS
11 Videos
Similar Questions
One side of a rectangle lies along the line 4x+7y+5=0. Two of its vertices are (−3,1) and (1,1). Find the equations of the other three sides.
View SolutionTwo persons P and Q crosses the river starting from point A on one side to exactly opposite point B on the other bank of the river. The person P crosses the river in the shortest path. The person Q crosses the river in shortest time and walks back to point B. Velocity of river is 3 kmph and speed of each boat is 5 kmph w.r.t river. If the two persons reach the point B in the same time, then the speed of walk of Q is
View Solution
Knowledge Check
If a vertex of a square is at the point (1,−1) and one of its sides lie along the line 3x−4y−17=0, then the area of the square is
A4 sq. unitsB3 sq. unitsC12 sq. unitsD2 sq. unitsThe chemical formula of deoxyribonucleic acid
(DNA) is now well established. The molecule is a
very long chain, the backbone of which consists of a
regular alternation of sugar and phosphate groups.
5 To each sugar is attached a nitrogenous base, which
can be of four different types. Two of the possible
bases—adenine and guanine—are purines, and the
other two—thymine and cytosine—are pyrimidines.
So far as is known, the sequence of bases along the
10 chain is irregular. The monomer unit, consisting of
phosphate, sugar and base, is known as a nucleotide.
The first feature of our structure which is of
biological interest is that it consists not of one chain,
but of two. These two chains are both coiled around
15 a common fiber axis. It has often been assumed that
since there was only one chain in the chemical
formula there would only be one in the structural
unit. However, the density, taken with the X-ray
evidence, suggests very strongly that there are two.
20. The other biologically important feature is the
manner in which the two chains are held together.
This is done by hydrogen bonds between the bases.
The bases are joined together in pairs, a single base
from one chain being hydrogen-bonded to a single
25 base from the other. The important point is that only
certain pairs of bases will fit into the structure.
One member of a pair must be a purine and the other
a pyrimidine in order to bridge between the two
chains. If a pair consisted of two purines, for
30 example, there would not be room for it.
We believe that the bases will be present almost
entirely in their most probable forms. If this is true,
the conditions for forming hydrogen bonds are more
restrictive, and the only pairs of bases possible are:
35 adenine with thymine, and guanine with cytosine.
Adenine, for example, can occur on either chain, but
when it does, its partner on the other chain must
always be thymine.
The phosphate-sugar backbone of our model is
40 completely regular, but any sequence of the pairs of
bases can fit into the structure. It follows that in a
long molecule many different permutations are
possible, and it therefore seems likely that the precise
sequence of bases is the code which carries the
45 genetical information. If the actual order of the bases
on one of the pair of chains were given, one could
write down the exact order of the bases on the other
one, because of the specific pairing. Thus one chain
is, as it were, the complement of the other, and it is
50 this feature which suggests how the deoxyribonucleic
acid molecule might duplicate itself.
The table shows, for various organisms, the percentage of
each of the four types of nitrogenous bases in that
organism’s DNA.
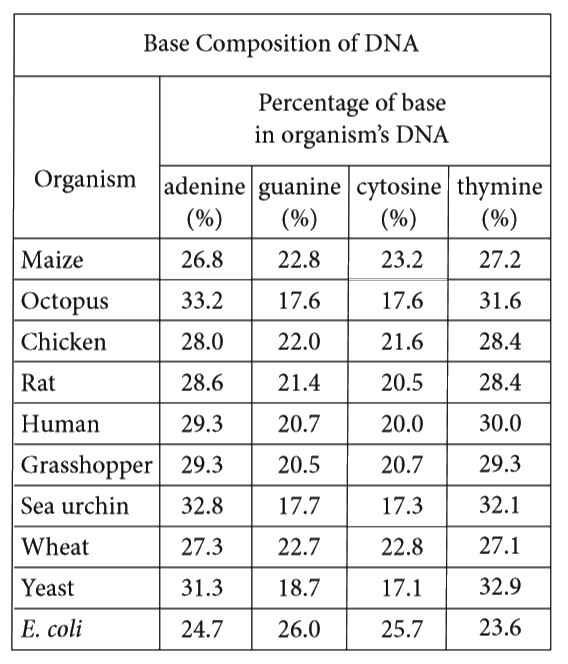
Adapted from Manju Bansal, “DNA Structure: Revisiting the
Watson-Crick Double Helix.” ©2003 by Current Science Association,
Bangalore
25. The authors’ main purpose of including the information about X-ray evidence and density is toAestablish that DNA is the molecule that carries the genetic information.Bpresent an alternate hypothesis about the composition of a nucleotide.Cprovide support for the authors’ claim about the number of chains in a molecule of DNA.Dconfirm the relationship between the density of DNA and the known chemical formula of DNA.
The distance between two stations is 340 km. Two trains start simultaneously from these stations on parallel tracks to cross each other. The speed of one of them is greater than that of the other by 5 km/hr. If the distance between the two trains after 2 hours of their start is 30 km, find the speed of each train.
View SolutionOne side of a rectangle lies along the line 4x+7y+5=0. Two of its vertices are (−3,1)and(1,1). Find the equations of the other three sides.
View SolutionOne side of a rectangle lies along the line 4x+7y+5=0. Two of its vertices are (−3,1)and(1,1). Find the equations of the other three sides.
View SolutionPlaces A and B are 100 km apart on a highway. One car starts from A and another from b at the same time. If the cars travel in the same direction at different speeds, they meet in 5 hours. If they travel towards each other, they meet in 1 hour. What are the speeds of the two cars
View SolutionPlaces A and B are 100 km apart on a highway. One car starts from A and another from b at the same time. If the cars travel in the same direction at different speeds, they meet in 5 hours. If they travel towards each other, they meet in 1 hour. What are the speeds of the two cars
View SolutionA boy walks uniformly along the sides of a rectangular park of size 400m×300m, starting from one corner to the other corner diagonally opposite. Which of the following statements is incorrect?
View Solution
