A particle is projected with velocity $2 (\sqrt{g h})$ , so that it just clears two walls of equal height h which are at a distance of 2h form each other. Show that the time of passing between the walls is $2 (\sqrt{\frac{h}{g}}) .$ [Hint : First find velocity at height h. Treat it as initial velocity and 2h as the range. ]

Video Solution

Text Solution

Verified by Experts

$s = u t + \frac{1}{2} a t^{2} \Rightarrow a = (u sin θ) t - \frac{1}{2} g t^{2}$

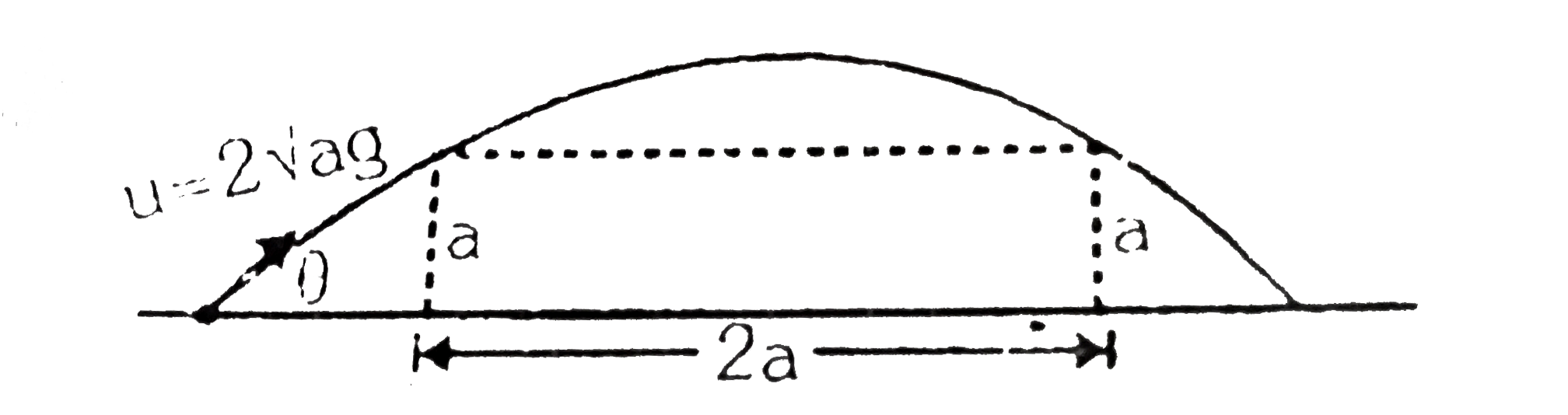
$\Rightarrow t = \frac{u sin θ \pm \sqrt{u^{2} {sin}^{2} θ - 2 a g}}{g}$
$Δ t = \frac{2 \sqrt{u^{2} {sin}^{2} θ - 2 a g}}{g}$
For horizontal motion : $2 a = u cos θ \times Δ t$
$\Rightarrow 2 a = \frac{u cos θ \times 2 \sqrt{u^{2} {sin}^{2} θ - 2 a g}}{g} \Rightarrow θ = 60^{\circ}$
$∴ Δ t = \frac{2 a}{u cos θ} = \frac{2 a}{2 \sqrt{a g} \times \frac{1}{2}} = 2 \sqrt{\frac{a}{g}}$

Updated on:21/07/2023