The reaction 2A+B→ product follow the mechanism :-
2A⇔A2(fast)
A2+B→P(slow)
The order of the reaction is
1.5
3
1
2
The correct Answer is:B
To determine the order of the reaction given the mechanism, we can follow these steps:
Step 1: Identify the Rate-Determining Step
The mechanism provided indicates that the second step (A2 + B → P) is the slow step, which means it is the rate-determining step. The rate law for the overall reaction will be based on this step.
Step 2: Write the Rate Law for the Slow Step
For the slow step, the rate law can be expressed as:
Rate=k(A2)(B)
where k is the rate constant, (A2) is the concentration of A2, and (B) is the concentration of B.
Step 3: Express (A2) in Terms of (A)
From the first step of the mechanism (2A ⇌ A2), we can derive the equilibrium expression. At equilibrium, we have:
Keq=(A2)(A)2
Thus, we can express (A2) as:
(A2)=Keq(A)2
where Keq is the equilibrium constant for the first step.
Step 4: Substitute (A2) into the Rate Law
Now, we substitute (A2) back into the rate law:
Rate=k(A2)(B)=k(Keq(A)2)(B)
This simplifies to:
Rate=kKeq(A)2(B)
Step 5: Determine the Overall Order of the Reaction
In the rate law Rate=kKeq(A)2(B), the order of the reaction is determined by the sum of the powers of the concentrations:
- The power of (A) is 2.
- The power of (B) is 1.
Thus, the overall order of the reaction is:
2+1=3
Final Answer
The order of the reaction is 3.
---
The mechanism provided indicates that the second step (A2 + B → P) is the slow step, which means it is the rate-determining step. The rate law for the overall reaction will be based on this step.
For the slow step, the rate law can be expressed as:
Rate=k(A2)(B)
where k is the rate constant, (A2) is the concentration of A2, and (B) is the concentration of B.
From the first step of the mechanism (2A ⇌ A2), we can derive the equilibrium expression. At equilibrium, we have:
Keq=(A2)(A)2
Thus, we can express (A2) as:
(A2)=Keq(A)2
where Keq is the equilibrium constant for the first step.
Now, we substitute (A2) back into the rate law:
Rate=k(A2)(B)=k(Keq(A)2)(B)
This simplifies to:
Rate=kKeq(A)2(B)
In the rate law Rate=kKeq(A)2(B), the order of the reaction is determined by the sum of the powers of the concentrations:
- The power of (A) is 2.
- The power of (B) is 1.
2+1=3
The order of the reaction is 3.
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - CHEMISTRY AT A GLANCEEXERCISE - INORGANIC CHEMISTRY300videos
CHEMISTRY AT A GLANCE
- BOOK - ALLENCHAPTER - CHEMISTRY AT A GLANCEEXERCISE - ORGANIC CHEMISTRY472videos
CHEMISTRY AT A GLANCE
- BOOK - ALLENCHAPTER - Chemical EquilibriumEXERCISE - All Questions30videos
Chemical Equilibrium
- BOOK - ALLENCHAPTER - ELECTROCHEMISTRYEXERCISE - EXERCISE -05 [B]38videos
ELECTROCHEMISTRY
Similar Questions
Knowledge Check
- Question 1 - Select One
A hypothetical reaction A2+B2→2AB follows the mechanism as given below:
A2⇔A+A(fast)
A+B2→AB+B (slow)
A+B→AB (fast)
The order of the overall reaction isA2B1C1.5Dzero - Question 2 - Select One
The reaction 2NO+Br2→2NOBr, obeys the following mechanism:
NO+Br2fast⇐⇒NOBr2,NOBr2+NOSlow−−→2NOBr The rate expression of the above reaction can be written asAr=k[NO]2[Br2]2Br=k[NO][Br2]Cr=k[NO][Br2]Dr=k[NOBr2]
2. The major product of the following reaction is
View SolutionFor a reaction, A+B→ Product, the rate law is given by r=k[A]12[B]2. What is the order of the reaction ?
View SolutionFor the reaction,, 2A+B→C+D, the order of reaction is
View Solution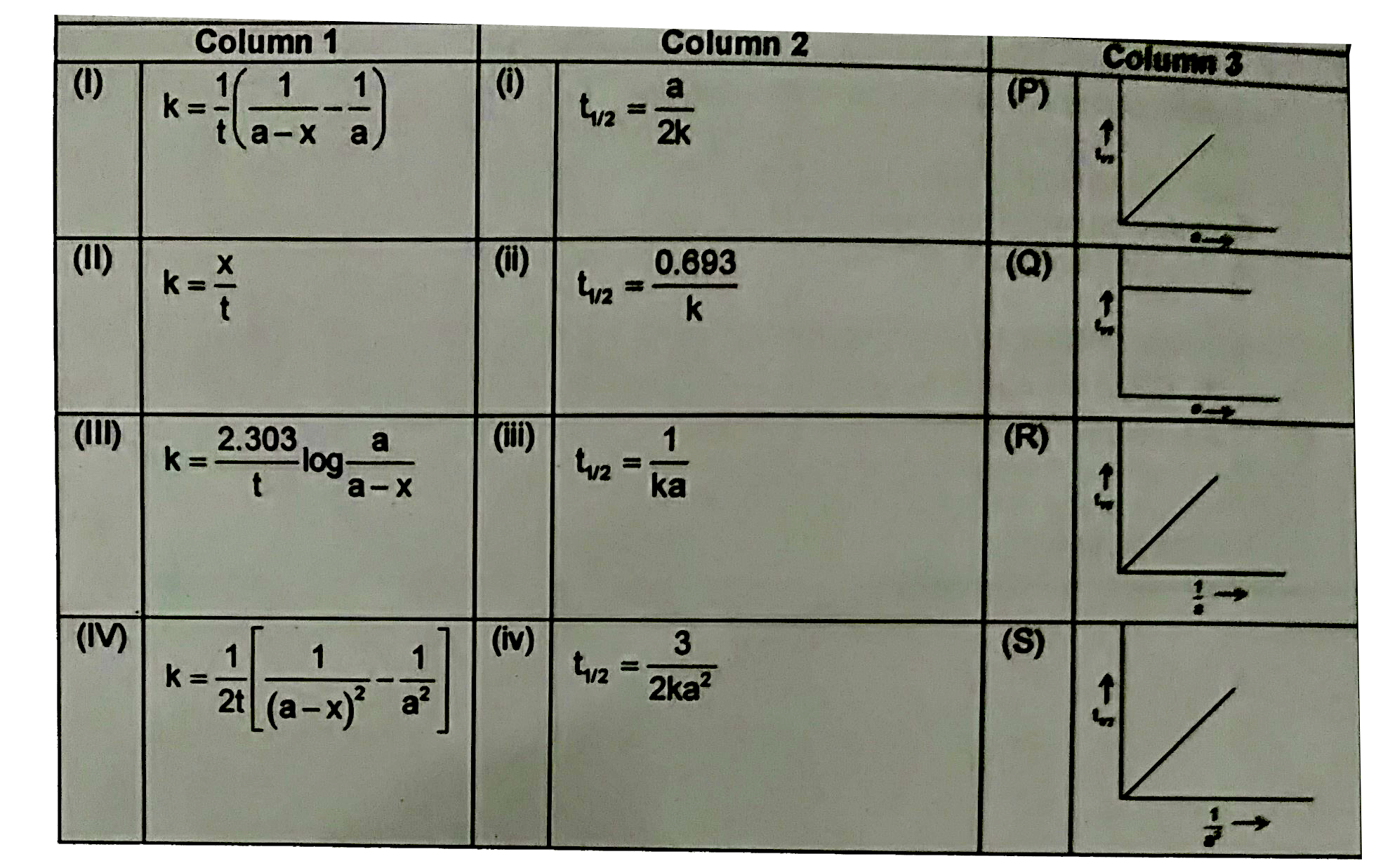
For the reaction 2A→Product, the rate equation is r=k[A]2
Which of the following is the only correct combination for the reaction?View SolutionA study of chemical kinetics of the reaction A+B→ products, gave the following data at 25∘C:
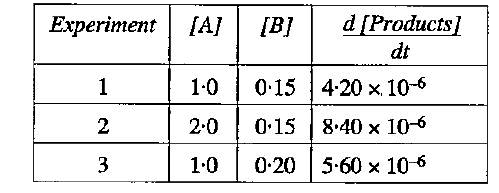
Find : (1) The order of reaction with respect to A. (2) The order of reaction with respect to B. (3) The rate law.View SolutionWrite the rate equation for the reaction 2A+B→C if the order of the reaction is zero .
View Solution