A body of mass 100g is sliding on a inclined plane with an inclination of 60∘. What is the frictional force experienced, if coeficient of friction is 1.7? (Take g=10m/s2)
0.85N
0.95N
1.05N
1.145N
The correct Answer is:A
To solve the problem of finding the frictional force experienced by a body sliding on an inclined plane, we can follow these steps:
Step 1: Identify the given values
- Mass of the body (m) = 100 g = 0.1 kg (conversion from grams to kilograms)
- Angle of inclination (θ) = 60°
- Coefficient of friction (μ) = 1.7
- Acceleration due to gravity (g) = 10 m/s²
Step 2: Calculate the weight of the body
The weight (W) of the body can be calculated using the formula:
W=m⋅g
Substituting the values:
W=0.1kg⋅10m/s2=1N
Step 3: Resolve the weight into components
The weight of the body can be resolved into two components:
- Perpendicular to the inclined plane: W_{\perpendicular} = W \cdot \cos(θ)
- Parallel to the inclined plane: W∥=W⋅sin(θ)
Calculating these components:
- W_{\perpendicular} = 1 \, \text{N} \cdot \cos(60°) = 1 \, \text{N} \cdot \frac{1}{2} = 0.5 \, \text{N}
- W∥=1N⋅sin(60°)=1N⋅√32≈0.866N
Step 4: Calculate the normal force
The normal force (N) acting on the body is equal to the perpendicular component of the weight:
N = W_{\perpendicular} = 0.5 \, \text{N}
Step 5: Calculate the frictional force
The frictional force (F) can be calculated using the formula:
F=μ⋅N
Substituting the values:
F=1.7⋅0.5N=0.85N
Conclusion
The frictional force experienced by the body is 0.85 N.
---
- Mass of the body (m) = 100 g = 0.1 kg (conversion from grams to kilograms)
- Angle of inclination (θ) = 60°
- Coefficient of friction (μ) = 1.7
- Acceleration due to gravity (g) = 10 m/s²
The weight (W) of the body can be calculated using the formula:
W=m⋅g
Substituting the values:
W=0.1kg⋅10m/s2=1N
The weight of the body can be resolved into two components:
- Perpendicular to the inclined plane: W_{\perpendicular} = W \cdot \cos(θ)
- Parallel to the inclined plane: W∥=W⋅sin(θ)
- W_{\perpendicular} = 1 \, \text{N} \cdot \cos(60°) = 1 \, \text{N} \cdot \frac{1}{2} = 0.5 \, \text{N}
- W∥=1N⋅sin(60°)=1N⋅√32≈0.866N
The normal force (N) acting on the body is equal to the perpendicular component of the weight:
N = W_{\perpendicular} = 0.5 \, \text{N}
The frictional force (F) can be calculated using the formula:
F=μ⋅N
Substituting the values:
F=1.7⋅0.5N=0.85N
The frictional force experienced by the body is 0.85 N.
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-I
52 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)
4 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
24 Videos
Similar Questions
Knowledge Check
A body of mass 100 g is sliding from an inclined plane of inclination 30∘. What is the frictional force experienced if μ=1.7 ?
A1.7×√2×(1/√3)NB1.7×√3×(1/2)NC1.7×√3 ND1.7×√2×(1/3) NA block of mass m=2kg is resting on a rough inclined plane of inclination of 30∘ as shown in figure. The coefficient of friction between the block and the plane is μ=0.5. What minimum force F should be applied perpendicular to the plane on the block, so that blocks does not slip on the plane? (g=10m/s2)
 A2.68 NBZeroC4.34 ND6.24 N
A2.68 NBZeroC4.34 ND6.24 N
A block slides down on inclined plane (angle of inclination 60∘ ) with an accelration g/2 Find the coefficient friction

View SolutionA block of mass m is kept on an inclined plane is angle inclination θ(<ϕ), where ϕ= angle of friction. Then :

View SolutionWhen a body rolls without sliding up an inclined plane the frictional force is :
View SolutionA body of mass M starts sliding down on the inclined plane where the critical angle is ∠ACB=30∘ as shown in figure. The coefficient of kinetic friction will be
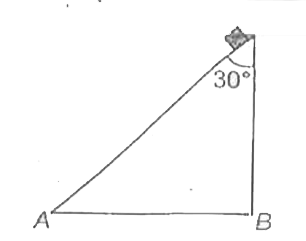
View SolutionA brick of mass 2kg begins to slide down on a plane inclined at an angle of 45∘ with the horizontal. The force of friction will be
View SolutionA body of mass 10kg is on a rough inclined plane having an inclination of 30° with the horizontal. If coefficient of friction between the surfaces of contact of the body and the plane is 0.5. Find the least force required to pull the body up the plane.
View Solution

