A barometer is faulty . When the true barometer reading are 73 cm and 75 cm of Hg , the faulty barometer reads 69 cm and 70 cm respectively (i) What is the total length of the barometer tube? (ii) What is the reading when the faulty barometer reads 69.5cm? (iii) What is the faulty barometer reading when the true barometer reads 74cm?
The correct Answer is:74 cm , 73.94 cm , 69.52cm
To solve the problem step by step, we will break it down into three parts as per the question:
Part (i): Finding the total length of the barometer tube
1. Understanding the problem:
- We have two true barometer readings: 73 cm and 75 cm.
- Corresponding faulty barometer readings are: 69 cm and 70 cm.
2. Setting up the equations:
- Let the height of trapped air in the barometer be x.
- For the first case (true reading = 73 cm, faulty reading = 69 cm):
P0=Pair+ρg(73)(1)
P0=P1+ρg(69)(2)
- For the second case (true reading = 75 cm, faulty reading = 70 cm):
P0=Pair+ρg(75)(3)
P0=P2+ρg(70)(4)
3. Finding the height of trapped air:
- From equations (1) and (2):
Pair+ρg(73)=P1+ρg(69)
Rearranging gives:
P1=Pair+ρg(4)(5)
- From equations (3) and (4):
Pair+ρg(75)=P2+ρg(70)
Rearranging gives:
P2=Pair+ρg(5)(6)
4. Using the constant temperature process:
- Since the trapped air follows a constant temperature process, we can write:
P1⋅V1=P2⋅V2
- Substituting from equations (5) and (6):
ρg(4)⋅A⋅x=ρg(5)⋅A⋅(x−1)
- Canceling A and ρg:
4x=5(x−1)
- Solving gives:
4x=5x−5⟹x=5 cm
5. Calculating the total length of the barometer tube:
- The total length of the barometer tube is the height of the mercury column plus the height of the trapped air:
Total Length=69 cm+5 cm=74 cm
Part (ii): Finding the reading when the faulty barometer reads 69.5 cm
1. Using the constant temperature process:
- Let h be the true reading when the faulty barometer reads 69.5 cm.
- The height of trapped air will be x−0.5 cm since the faulty reading is 0.5 cm less than 69 cm.
- Using the equation:
P1⋅V1=P3⋅V3
- Substituting:
ρg(4)⋅A⋅x=P3⋅A⋅(x−0.5)
- Canceling A and ρg:
4x=P3(x−0.5)
- Substituting x=5:
4(5)=P3(5−0.5)⟹20=P3(4.5)
- Solving gives:
P3=204.5≈4.44 cm
- The actual height:
Actual Reading=69.5+4.44=73.94 cm
Part (iii): Finding the faulty barometer reading when the true barometer reads 74 cm
1. Using the constant temperature process:
- Let y be the faulty reading when the true barometer reads 74 cm.
- The height of trapped air will be x cm.
- Using the equation:
P1⋅V1=P2⋅V2
- Substituting:
ρg(4)⋅A⋅x=ρg(74−y)⋅A⋅(74−x)
- Canceling A and ρg:
4x=(74−y)(74−x)
- Rearranging gives:
74−y=4x74−x
- Substituting x=5:
74−y=2069⟹y=74−2069≈69.528 cm
Final Answers:
1. Total length of the barometer tube: 74 cm
2. Actual reading when the faulty barometer reads 69.5 cm: 73.94 cm
3. Faulty barometer reading when the true barometer reads 74 cm: 69.528 cm
- We have two true barometer readings: 73 cm and 75 cm.
- Corresponding faulty barometer readings are: 69 cm and 70 cm.
- Let the height of trapped air in the barometer be x.
- For the first case (true reading = 73 cm, faulty reading = 69 cm):
P0=Pair+ρg(73)(1)
P0=P1+ρg(69)(2)
- For the second case (true reading = 75 cm, faulty reading = 70 cm):
P0=Pair+ρg(75)(3)
P0=P2+ρg(70)(4)
- From equations (1) and (2):
Pair+ρg(73)=P1+ρg(69)
Rearranging gives:
P1=Pair+ρg(4)(5)
- From equations (3) and (4):
Pair+ρg(75)=P2+ρg(70)
Rearranging gives:
P2=Pair+ρg(5)(6)
- Since the trapped air follows a constant temperature process, we can write:
P1⋅V1=P2⋅V2
- Substituting from equations (5) and (6):
ρg(4)⋅A⋅x=ρg(5)⋅A⋅(x−1)
- Canceling A and ρg:
4x=5(x−1)
- Solving gives:
4x=5x−5⟹x=5 cm
- The total length of the barometer tube is the height of the mercury column plus the height of the trapped air:
Total Length=69 cm+5 cm=74 cm
- Let h be the true reading when the faulty barometer reads 69.5 cm.
- The height of trapped air will be x−0.5 cm since the faulty reading is 0.5 cm less than 69 cm.
- Using the equation:
P1⋅V1=P3⋅V3
- Substituting:
ρg(4)⋅A⋅x=P3⋅A⋅(x−0.5)
- Canceling A and ρg:
4x=P3(x−0.5)
- Substituting x=5:
4(5)=P3(5−0.5)⟹20=P3(4.5)
- Solving gives:
P3=204.5≈4.44 cm
- The actual height:
Actual Reading=69.5+4.44=73.94 cm
- Let y be the faulty reading when the true barometer reads 74 cm.
- The height of trapped air will be x cm.
- Using the equation:
P1⋅V1=P2⋅V2
- Substituting:
ρg(4)⋅A⋅x=ρg(74−y)⋅A⋅(74−x)
- Canceling A and ρg:
4x=(74−y)(74−x)
- Rearranging gives:
74−y=4x74−x
- Substituting x=5:
74−y=2069⟹y=74−2069≈69.528 cm
1. Total length of the barometer tube: 74 cm
2. Actual reading when the faulty barometer reads 69.5 cm: 73.94 cm
3. Faulty barometer reading when the true barometer reads 74 cm: 69.528 cm
Topper's Solved these Questions
- GEOMETRICAL OPTICSBook:ALLENChapter:GEOMETRICAL OPTICSExercise:EXERCISE - 05 (A)
Explore 73 Videos
- GEOMETRICAL OPTICSBook:ALLENChapter:GEOMETRICAL OPTICSExercise:EXERCISE - 05 (B)
Explore 58 Videos
- GEOMETRICAL OPTICSBook:ALLENChapter:GEOMETRICAL OPTICSExercise:EXERCISE - 04 (A)
Explore 47 Videos
- CURRENT ELECTRICITYBook:ALLENChapter:CURRENT ELECTRICITYExercise:EX.II
Explore 66 Videos
- GRAVITATIONBook:ALLENChapter:GRAVITATIONExercise:EXERCISE 4
Explore 9 Videos
Similar Questions
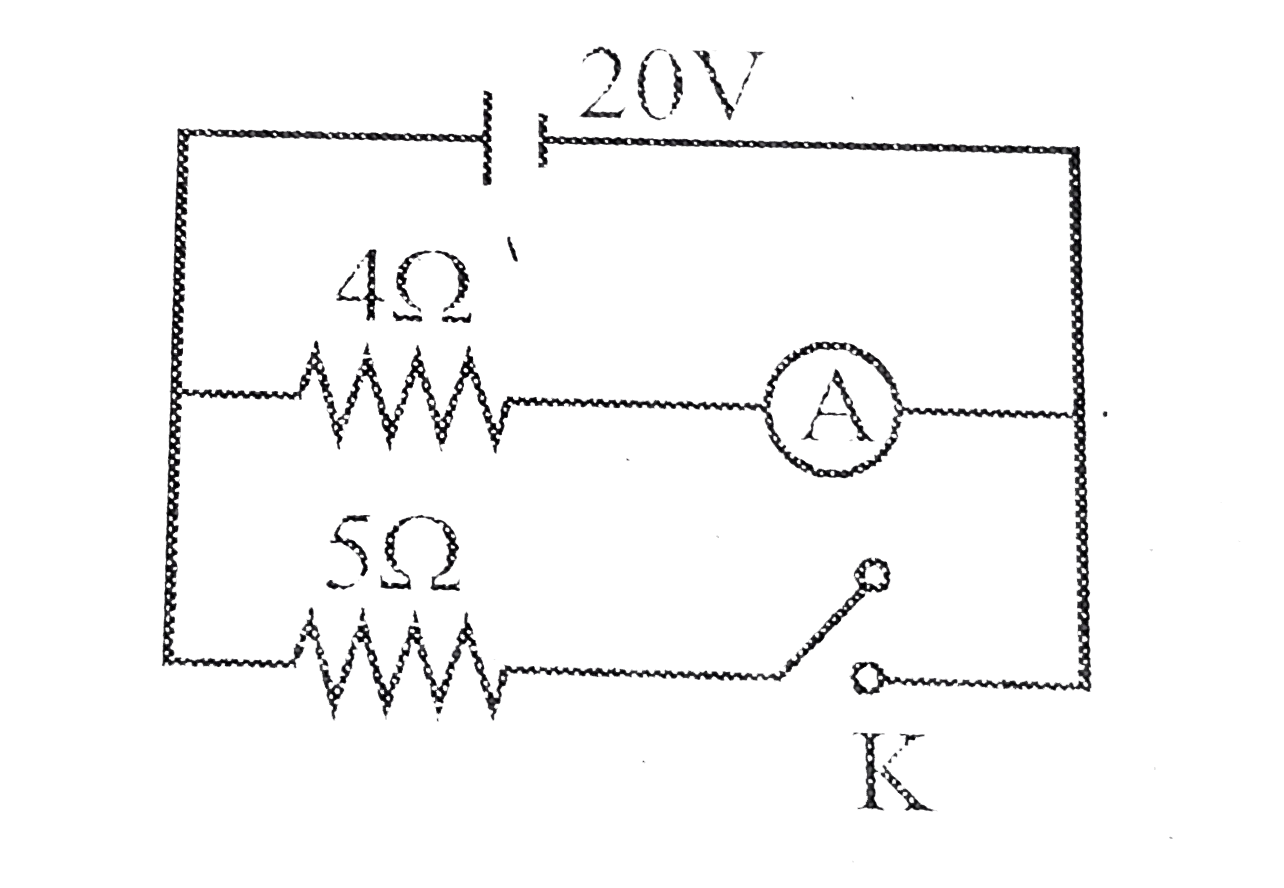
In the given figure when the key is open the ammeter reading is 5 amp. What will be the ammeter reading when the key is closed
View SolutionThe length and breadth of a table are (125±0.4)cm and (75±0.5)cm respectively. What is the area of the table?
View SolutionTwo objects A and B are of lengths 5 cm and 7cm determined with errors 0.1 cm and 0.2 cm respectively.What is the error in determing (a) the total length and (b) the difference in their lengths?
View SolutionA faulty thermometer reads freezing point and boiling point of water as −5∘C abd 95∘C respectively . What is the correct value of temperature as it reads 60∘C on faulty thermometer?
View SolutionA barometer kept in an elevator reads 76 cm when it is at rest. If the elevator goes up with increasing speed, the reading will be
View SolutionHow is the reading of a barometer affected when it is taken to (i) a mine, and (ii) a hill ?
View SolutionWhat is the barometric height of a liquid of density 3.4 g cm−3 at a place, where that for mercury barometer is 70 cm?
View SolutionThe most accurate reading of the length of a 6.28 cm long fibre is
View SolutionThe most accurate reading of the length of a 6.28 cm long fibre is
View SolutionA barometer kept in an elevator accelerating upward reads 76 cm. The air pressure in the elevator is
View Solution
