The P.E. of an oscillation particle at rest position is 10J and its average K.E. is 5J. The total energy of particle at any instant will be-
10J
20J
25J
5J
The correct Answer is:C
To solve the problem, we need to determine the total energy of an oscillating particle given its potential energy (P.E.) at the rest position and its average kinetic energy (K.E.).
1. Understand the Definitions:
- The potential energy (P.E.) of the particle at the rest position is given as 10J.
- The average kinetic energy (K.E.) of the particle is given as 5J.
2. Identify Energy Conservation:
- In simple harmonic motion, the total mechanical energy (E) of the system is conserved. This means that the total energy is the sum of the potential energy and kinetic energy at any point in time.
- Mathematically, this can be expressed as:
E=P.E.+K.E.
3. Calculate Total Energy:
- At the rest position, the potential energy is at its maximum, and the kinetic energy is zero. Therefore, the total energy at this position is:
E=P.E.+0=10J
4. Determine Total Energy at Any Instant:
- The total energy of the particle remains constant throughout its motion. Therefore, the total energy at any instant will also be 10J.
5. Conclusion:
- The total energy of the particle at any instant is 10J.
Final Answer:
The total energy of the particle at any instant will be 10J.
---
- The potential energy (P.E.) of the particle at the rest position is given as 10J.
- The average kinetic energy (K.E.) of the particle is given as 5J.
- In simple harmonic motion, the total mechanical energy (E) of the system is conserved. This means that the total energy is the sum of the potential energy and kinetic energy at any point in time.
- Mathematically, this can be expressed as:
E=P.E.+K.E.
- At the rest position, the potential energy is at its maximum, and the kinetic energy is zero. Therefore, the total energy at this position is:
E=P.E.+0=10J
- The total energy of the particle remains constant throughout its motion. Therefore, the total energy at any instant will also be 10J.
- The total energy of the particle at any instant is 10J.
The total energy of the particle at any instant will be 10J.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-02
19 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise- 3 Match The Column
1 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - SOME WORKED OUT EXAMPLES
29 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
24 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
The potential energy of a particle executing S.H.M. is 2.5 J, when its displacement is half of amplitude. The total energy of the particle will be
View SolutionThe potential energy of a particle executing S H M is 25 J. when its displacement is half of amplitude. The total energy of the particle is
View SolutionThe potential energy of a harmonic oscillation when is half way to its and end point is (where E it’s the total energy)
View SolutionForce acting on a particle is F=−8x in S.H.M. The amplitude of oscillation is 2 (in m) and mass of the particle is 0.5 kg. The total mechanical energy of the particle is 20 J. Find the potential energy of the particle in mean position (in J).
View SolutionVelocity of a particle at some instant is v=(3ˆi+4ˆj+5ˆk)m/s. Find speed of the particle at this instant.
View SolutionBinding energy of a particle on the surface of earth is E. Kinetic energy grater than E is given to this particle. Then total energy of particle will become
View SolutionPotential energy of a particle at mean position is 4 J and at extreme position is 20 J. Given that amplitude of oscillation is A. Match the following two columns
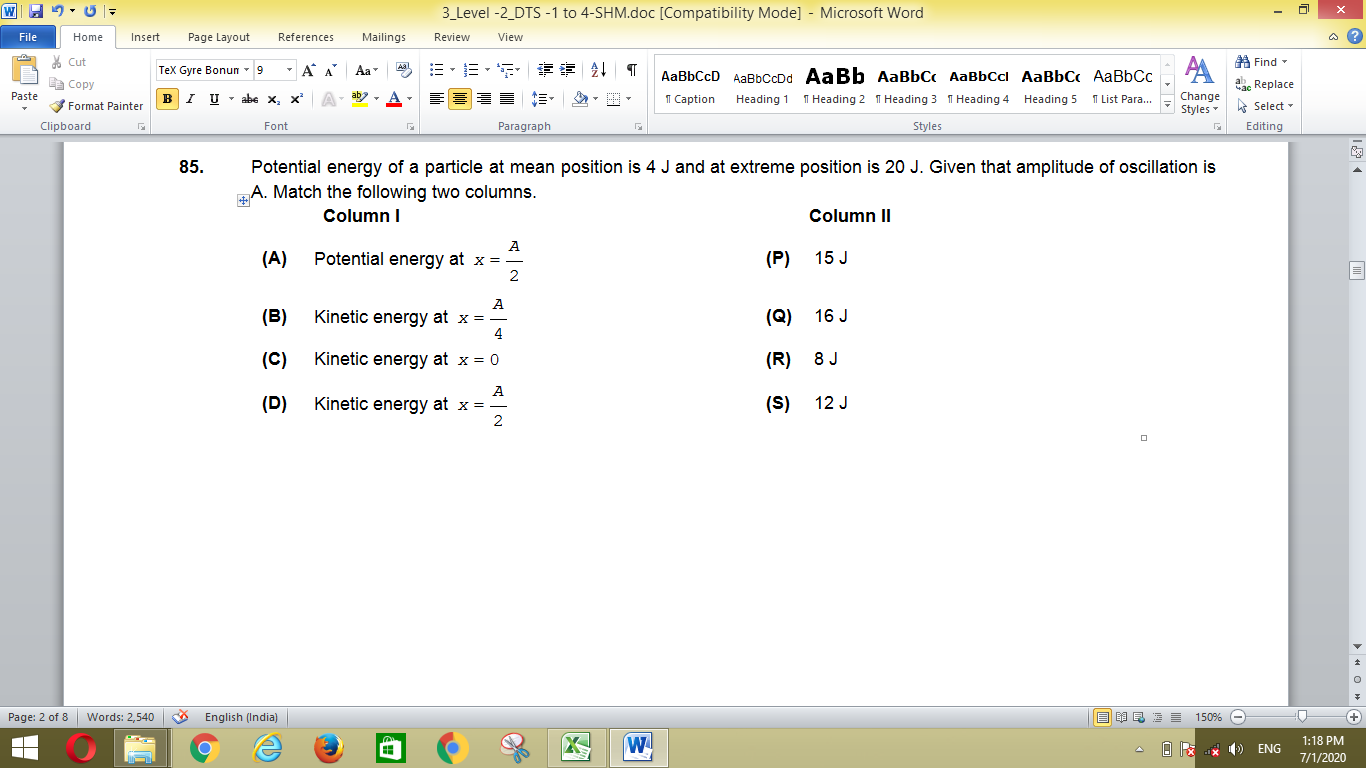
View SolutionThe potential energy of a particle execuring S.H.M. is 5 J, when its displacement is half of amplitude. The total energy of the particle be
View SolutionA 20g particle is oscillating simple harmonically with a period of 2sec and maximum kinetic energy 2J. The total mechanical energy of the particle is zero , find
a Amplitude of oscillation
b. potential energy as a function of displacement x relative to mean position.
View SolutionThe position of a particle at any point instant is x . Find the velocity and acceleration at any instant .
View Solution
