The half lives of radioactive elements X and Y are 3 mintue and 27 minute respectively. If the activities of both are same, then calculate the ratio of number of atoms of X and Y.
The correct Answer is:1/9
To solve the problem, we need to find the ratio of the number of atoms of radioactive elements X and Y, given that their activities are the same and their half-lives are different.
1. Understand the Concept of Activity:
The activity (A) of a radioactive substance is defined as the number of disintegrations per unit time. It is directly proportional to the number of active nuclei (n) and the decay constant (λ):
A=λn
2. Set Up the Equation for Activities:
Since the activities of elements X and Y are the same, we can write:
AX=AY
This implies:
λXnX=λYnY
3. Relate Decay Constant to Half-Life:
The decay constant (λ) is related to the half-life (t_half) by the formula:
λ=0.693thalf
Thus, we can express λ for both elements:
λX=0.693thalf,XandλY=0.693thalf,Y
4. Substitute the Half-Lives:
Given that the half-life of X is 3 minutes and that of Y is 27 minutes, we can substitute these values:
λX=0.6933andλY=0.69327
5. Substitute λ into the Activity Equation:
Now, substituting λ into the activity equation gives:
0.6933nX=0.69327nY
6. Cancel Out Common Terms:
The 0.693 cancels out from both sides:
nX3=nY27
7. Rearranging the Equation:
Rearranging the equation to find the ratio of the number of atoms:
nXnY=327
8. Simplify the Ratio:
Simplifying the ratio gives:
nXnY=19
Final Answer:
The ratio of the number of atoms of X to Y is:
nXnY=19
The activity (A) of a radioactive substance is defined as the number of disintegrations per unit time. It is directly proportional to the number of active nuclei (n) and the decay constant (λ):
A=λn
Since the activities of elements X and Y are the same, we can write:
AX=AY
This implies:
λXnX=λYnY
The decay constant (λ) is related to the half-life (t_half) by the formula:
λ=0.693thalf
Thus, we can express λ for both elements:
λX=0.693thalf,XandλY=0.693thalf,Y
Given that the half-life of X is 3 minutes and that of Y is 27 minutes, we can substitute these values:
λX=0.6933andλY=0.69327
Now, substituting λ into the activity equation gives:
0.6933nX=0.69327nY
The 0.693 cancels out from both sides:
nX3=nY27
Rearranging the equation to find the ratio of the number of atoms:
nXnY=327
Simplifying the ratio gives:
nXnY=19
The ratio of the number of atoms of X to Y is:
nXnY=19
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-05(B)
46 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - MCQ s
8 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise - 58
1 Video
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
24 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
Half - lives of two radioactive elements A and B are 20 minutes and 40 minutes respectively. . Initially . The samples have equal number of nuclie After 80 minutes ,the ratio of decyed number of AandB nuclei will be
View SolutionHalf - lives of two radioactive elements A and B are 20 minutes and 40 minutes respectively. Initially, The samples have equal number of nuclie. After 80 minutes ,the ratio of decayed number of AandB nuclei will be
View Solution
Knowledge Check
The half - life period of a radioactive element x is same as the mean life time of another radioactive element y Initially both of them have the same number of atoms. Then,n
AX and Y decay at same rate alwaysBX will decay faster than YCY will decay faster than XDX and Y have same decay rate initiallyMarks of X and Y are in the ratio 3 : 11 respectively. If the marks of X is 9, then find the marks of Y.
A55B22C33D44
Half lives of two radioactive nuclei A and B are 10 minutes and 20 minutes respectively, If initially a sample has equal number of nuclei, then after 60 minutes, the ratio of decayed numbers of nuclei A and B will be :
View SolutionTwo radioactive elements X and Y have half-life periods of 50 minutes and 100 minutes, respectively. Initially, both of them contain equal number of atoms. Find the ratio of atoms left NXNY after 200 minutes.
View SolutionThe half-life period of a radioactive element x is same as the mean life time of another radioactive element y. Initially, both of them have the same number of atoms. Then,
(a) x and y have the same decay rate initially
(b) x and y decay at the same rate always
(c) y will decay at a faster rate than x
(d) x will decay at a faster rate than y
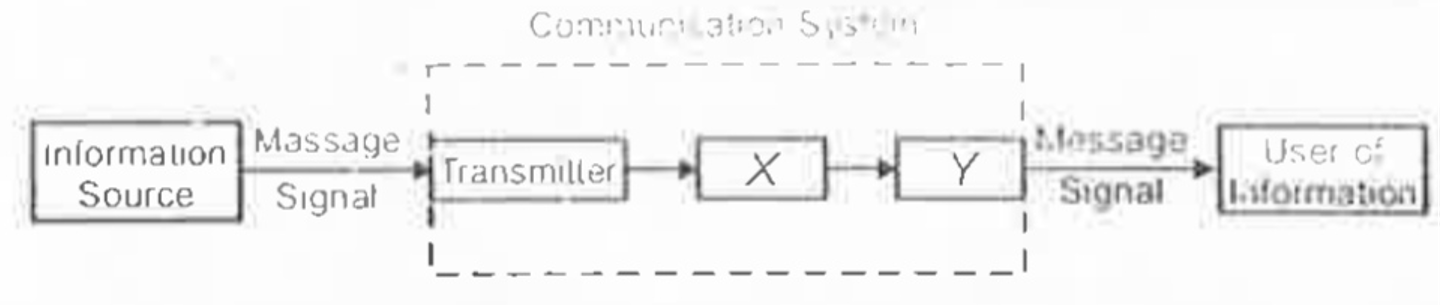
View SolutionThe element X and Y in the communication system (figure) are respectively
View SolutionThe half - line period a radioactive element X is same as the mean life time of another radioactive element Y. Initially both of them have the same number of atoms. Then:
View SolutionElements X, Y and Z have atomic numbers 6, 9 and 12 respectively. Which one :
forms a cation
View Solution
