Flourine reacts with uranium to produce uranium hexaflouride ,
UF6, as represented by this equation
U(s)+3F2(g)→UF6(g)
How many fluorine molecules are required to produce 7.04 mg of uranium hexafluoride ,
UF6 , from an excess of uranium? The molar mass of UF6 is 352 gm/mol (NA=6×1023)
The correct Answer is:3.6×1019
To solve the problem of how many fluorine molecules are required to produce 7.04 mg of uranium hexafluoride (UF₆), we will follow these steps:
Step 1: Convert mass from milligrams to grams
Given mass of UF₆ = 7.04 mg
To convert milligrams to grams:
Mass in grams=7.04 mg1000=0.00704 g
Step 2: Calculate moles of UF₆
Using the molar mass of UF₆, which is 352 g/mol, we can calculate the number of moles of UF₆ using the formula:
Moles of UF₆=Mass of UF₆Molar mass of UF₆=0.00704 g352 g/mol
Moles of UF₆=2×10−5 mol
Step 3: Calculate the number of molecules of UF₆
To find the number of molecules, we use Avogadro's number (NA=6×1023 molecules/mol):
Number of molecules of UF₆=Moles of UF₆×NA=2×10−5 mol×6×1023 molecules/mol
Number of molecules of UF₆=1.2×1019 molecules
Step 4: Determine the number of fluorine molecules required
From the balanced chemical equation:
U(s)+3F2(g)→UF6(g)
It shows that 1 mole of UF₆ requires 3 moles of F₂. Therefore, the number of moles of F₂ required is:
Moles of F₂=3×Moles of UF₆=3×2×10−5 mol=6×10−5 mol
Step 5: Calculate the number of molecules of F₂
Using Avogadro's number again:
Number of molecules of F₂=Moles of F₂×NA=6×10−5 mol×6×1023 molecules/mol
Number of molecules of F₂=3.6×1019 molecules
Final Answer
The number of fluorine molecules required to produce 7.04 mg of uranium hexafluoride (UF₆) is:
3.6×1019 molecules
Given mass of UF₆ = 7.04 mg
Mass in grams=7.04 mg1000=0.00704 g
Using the molar mass of UF₆, which is 352 g/mol, we can calculate the number of moles of UF₆ using the formula:
Moles of UF₆=Mass of UF₆Molar mass of UF₆=0.00704 g352 g/mol
Moles of UF₆=2×10−5 mol
To find the number of molecules, we use Avogadro's number (NA=6×1023 molecules/mol):
Number of molecules of UF₆=Moles of UF₆×NA=2×10−5 mol×6×1023 molecules/mol
Number of molecules of UF₆=1.2×1019 molecules
From the balanced chemical equation:
U(s)+3F2(g)→UF6(g)
It shows that 1 mole of UF₆ requires 3 moles of F₂. Therefore, the number of moles of F₂ required is:
Moles of F₂=3×Moles of UF₆=3×2×10−5 mol=6×10−5 mol
Using Avogadro's number again:
Number of molecules of F₂=Moles of F₂×NA=6×10−5 mol×6×1023 molecules/mol
Number of molecules of F₂=3.6×1019 molecules
The number of fluorine molecules required to produce 7.04 mg of uranium hexafluoride (UF₆) is:
3.6×1019 molecules
Topper's Solved these Questions
- MOLE CONCEPTBOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - S-II
18 Videos
- MOLE CONCEPTBOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - O-I
45 Videos
- MOLE CONCEPTBOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - Basic Exercise
29 Videos
- METALLURGYBOOK - ALLENCHAPTER - METALLURGYEXERCISE - EXERCISE-05 [B]
26 Videos
- p-Block Element BOOK - ALLENCHAPTER - p-Block Element EXERCISE - All Questions
20 Videos
Similar Questions
The number of F− ions in 2.1 g AlF3 is (NA=6×1023)
View SolutionAmmonia and oxygen combine to produce water vapour and nitric oxide as per the chemical equation: 4NH3(g)+5O2(g)→6H2O(g)+4NO.
(ii) How many moles of oxygen are required to burn 85 g of ammonia?
View Solution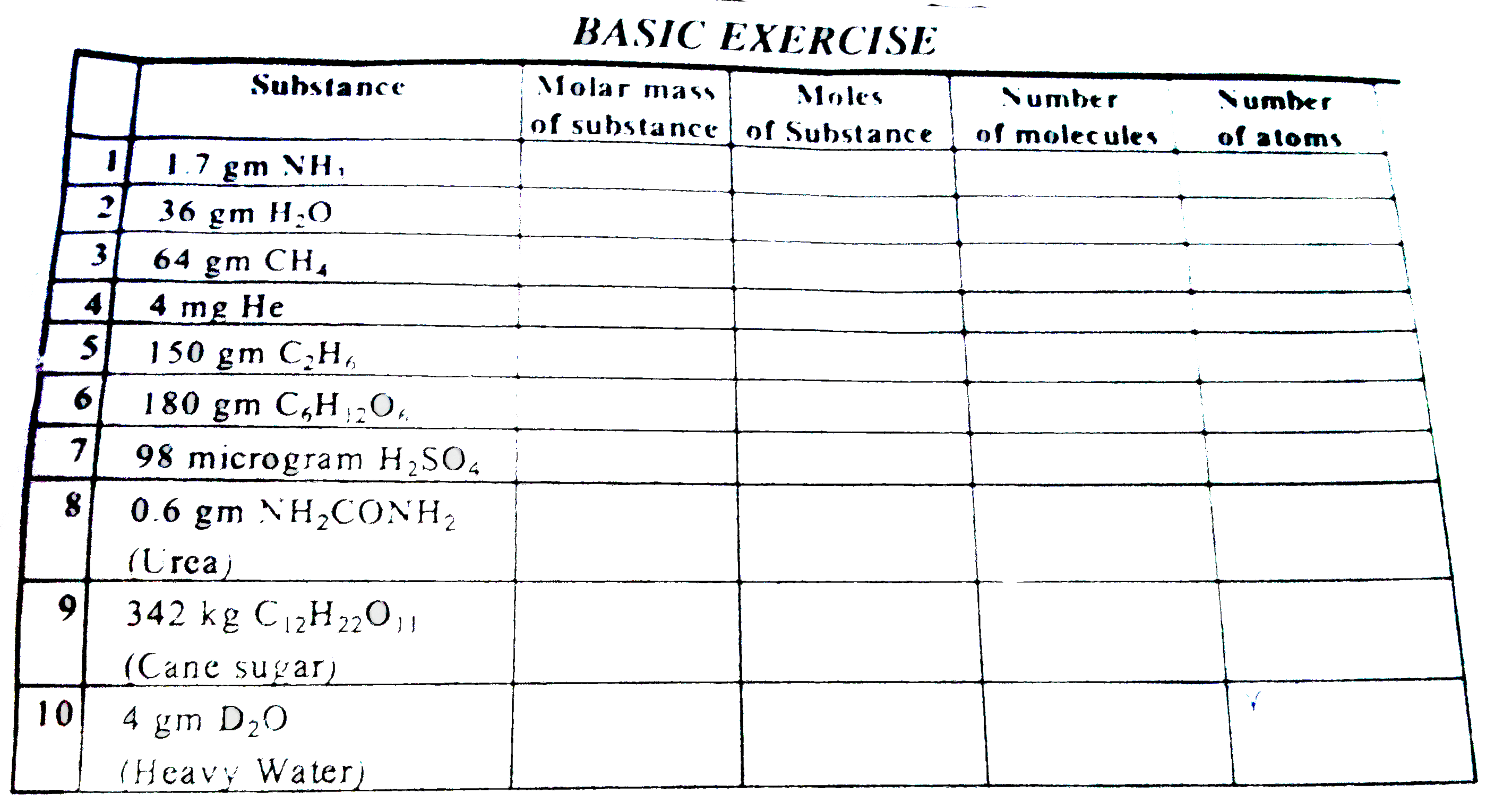
How many moles of O-atoms are in 2.7×1025 molecules of CO2(NA=6×1023)
View Solution0.250g of an element M, reacts with excess fluorine to produce 0.547g of the hexafluoride MF6. What is the elemejnt : [Cr=52,Mo=96,S=32,Te=127.6] .
View Solution41.6 g BaCl2 react with how many grams of sodium sulphate to produce 46.6 g of barium sulphate and 23.4 g of sodium chloride ?
View SolutionCalculate the mass of one molecule of oxygen. It is given that molar mass of O2 is 32 g and Avogadro's number is 6.022×1023
View SolutionAmmonia burns in oxygen as 2NH3(g)+2.5O2(g)→2NO(g)+3H2O(g)
What volume of O2 at STP is required to produce 10 mol of products (4NO+6H2O)?
View SolutionAn element with density 2.8 cm3 forms a f.c.c unit cell with edge length 4×10−8 cm. calculate the molar mass of the element. Given: (NA=6.022×1023)mol−1
View SolutionThe molecular mass of a compound is 75
(i) What is the mass of 6.022×1020 molecules , in gm
(ii) How many molecules are in its 2.5 mg
View SolutionConsider the following equation
H4P2O7+2NaOH→Na2H2P2O7+2H2O
If 534 gm of H4P2O7 is reacted with 3.0×1024 formula units of NaOH ,then total number of moles of H2O is produced is (NA = 6×1023 )
View Solution
