The density of a sample of SO3 gas is 2.5 g/L at 0∘C and 1 atm . It's degree of dissociation into SO2 and O2 gases is :
67
17
37
57
The correct Answer is:A
To find the degree of dissociation of SO3 gas into SO2 and O2, we can follow these steps:
Step 1: Understand the Reaction
The dissociation of sulfur trioxide can be represented as:
SO3⇌SO2+12O2
From this reaction, we can see that 1 mole of SO3 produces 1 mole of SO2 and 0.5 moles of O2, totaling 1.5 moles of products.
Step 2: Use the Density to Find Molecular Weight
We are given the density of the gas mixture at equilibrium:
Density=2.5g/L
Using the ideal gas law, we can relate density to molecular weight:
PV=nRT⟹P=nVRT⟹Density=nV⋅Molecular Weight
Thus, we can express it as:
Density=P⋅Molecular WeightRT
Where:
- P=1atm
- R=0.081L atm K−1mol−1
- T=273K
Rearranging gives:
Molecular Weight=Density⋅RTP
Step 3: Calculate the Molecular Weight of the Mixture
Substituting the values:
Molecular Weight=2.5g/L⋅0.081L atm K−1mol−1⋅273K1atm
Calculating this gives:
Molecular Weight=2.5⋅0.081⋅2731≈56g/mol
Step 4: Calculate the Vapor Density at Equilibrium
The vapor density d of the mixture can be calculated as:
d=Molecular Weight2=562=28g/mol
Step 5: Calculate the Initial Vapor Density of SO3
The molecular weight of SO3 is 80 g/mol, so its vapor density is:
D=802=40g/mol
Step 6: Use the Degree of Dissociation Formula
The degree of dissociation α can be calculated using the formula:
α=D−dn−1⋅d
Where n is the total number of moles of gas after dissociation. Here, n=32 (1 mole of SO3 gives 1 mole of SO2 and 0.5 moles of O2).
Substituting the values:
α=40−2832−1⋅28
Calculating the numerator:
40−28=12
Calculating the denominator:
32−1=12
Thus:
α=1212⋅28=12⋅2⋅28=24⋅28=6
Finally, we find:
α=1214=67
Conclusion
The degree of dissociation of SO3 into SO2 and O2 is:
α=67
The dissociation of sulfur trioxide can be represented as:
SO3⇌SO2+12O2
From this reaction, we can see that 1 mole of SO3 produces 1 mole of SO2 and 0.5 moles of O2, totaling 1.5 moles of products.
We are given the density of the gas mixture at equilibrium:
Density=2.5g/L
Using the ideal gas law, we can relate density to molecular weight:
PV=nRT⟹P=nVRT⟹Density=nV⋅Molecular Weight
Thus, we can express it as:
Density=P⋅Molecular WeightRT
Where:
- P=1atm
- R=0.081L atm K−1mol−1
- T=273K
Molecular Weight=Density⋅RTP
Substituting the values:
Molecular Weight=2.5g/L⋅0.081L atm K−1mol−1⋅273K1atm
Calculating this gives:
Molecular Weight=2.5⋅0.081⋅2731≈56g/mol
The vapor density d of the mixture can be calculated as:
d=Molecular Weight2=562=28g/mol
The molecular weight of SO3 is 80 g/mol, so its vapor density is:
D=802=40g/mol
The degree of dissociation α can be calculated using the formula:
α=D−dn−1⋅d
Where n is the total number of moles of gas after dissociation. Here, n=32 (1 mole of SO3 gives 1 mole of SO2 and 0.5 moles of O2).
α=40−2832−1⋅28
Calculating the numerator:
40−28=12
Calculating the denominator:
32−1=12
Thus:
α=1212⋅28=12⋅2⋅28=24⋅28=6
Finally, we find:
α=1214=67
The degree of dissociation of SO3 into SO2 and O2 is:
α=67
Topper's Solved these Questions
- MOLE CONCEPTBOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - O-II
11 Videos
- MOLE CONCEPTBOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - O-II (Assertion Reason:)
2 Videos
- MOLE CONCEPTBOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - S-II
18 Videos
- METALLURGYBOOK - ALLENCHAPTER - METALLURGYEXERCISE - EXERCISE-05 [B]
26 Videos
- p-Block Element BOOK - ALLENCHAPTER - p-Block Element EXERCISE - All Questions
20 Videos
Similar Questions
If a sample of pure SO4 gas is heated to 600∘C, it dissociates into SO2 and O2 gases upto 50% .What is the average molar mass of the final sample.
View SolutionCalculate the total number of atoms in 5.6 L of SO2 as at 0∘C and
1 atm :-
View Solution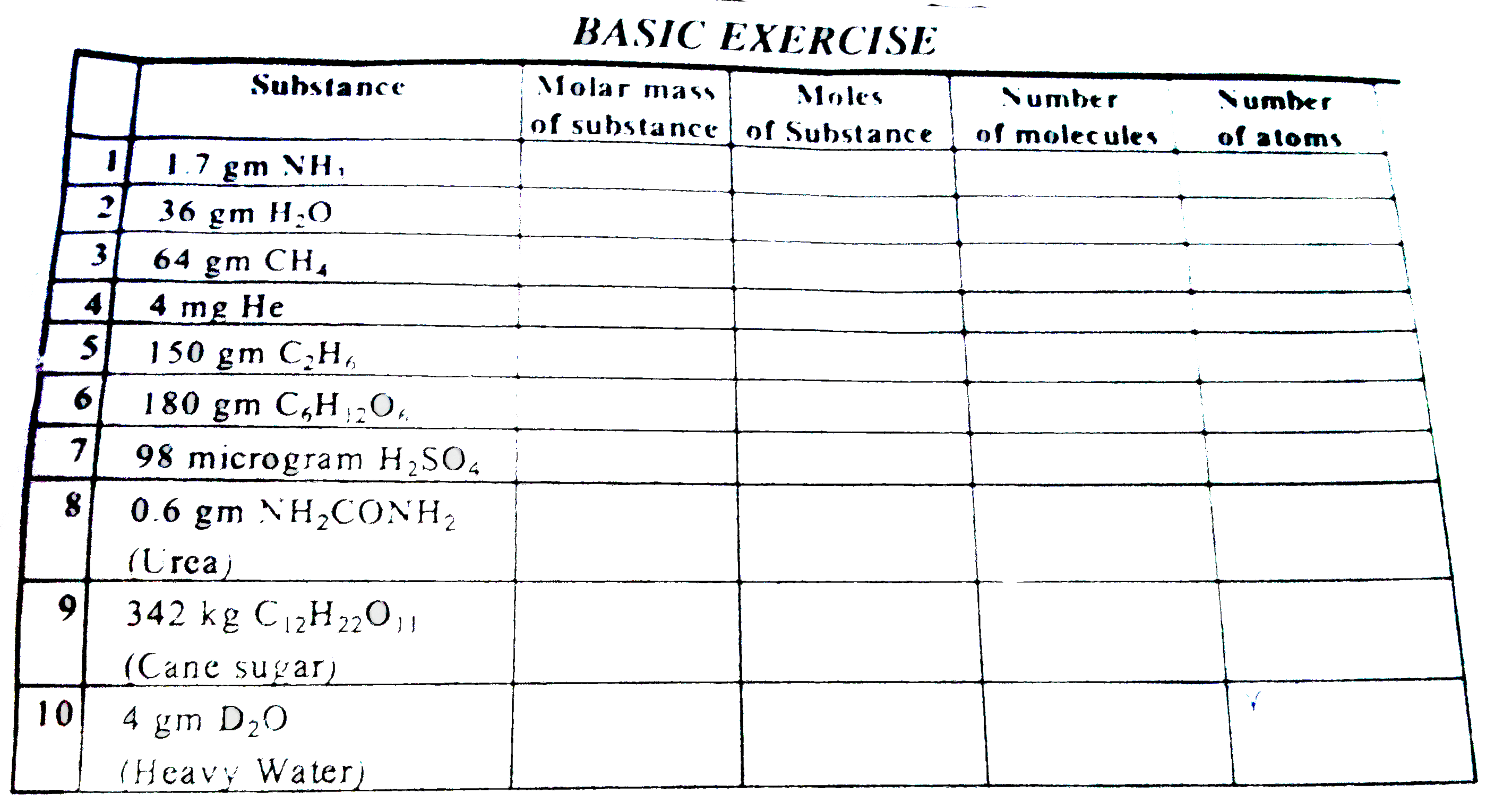
Find moles of O-atoms in 5.6 litres of SO3 at 0∘C ,1 atm ?
View SolutionWhen 0.004MNa2SO4 is an isotonic acid with 0.01Mglucose, the degree of dissociation of Na2SO4 is
View Solution16 g of SOx gas occupies 5.6 L at 1 atm and 273 K.What will be the value of x ?
View SolutionFor the dissociation reaction
N2O4(g)⇔2NO2(g),
the equilibrium constant KP is 0.120 atm at 298K and total pressure of system is 2 atm. Calculate the degree of dissociation of N2O4.
View SolutionCalculate the density of SO2 at 27∘C and 1.5 atm pressure.
View SolutionThe ratio of the rate of diffusion of a sample of N2O4 partially dissociated in to NO2 to pure hydrogen was found to be 1:5 . Calculate the degree of dissociation of N2O4.
View SolutionThe density of O2 gas at 127o C and 4.0 atm pressure is (R = 0.082 L atm K−1 mol−1)
View Solution0.2 g of a sample of H2O2 required 10 mL of 1 N KMnO4 in a titration in the presence of H2SO4 Purity of H2O2 is :
View Solution