Coefficient of friction between 5 kg and 10 kg block is 0.5. If friction between them is 20 N. What is the value of force being applied on 5 kg? The floor is frictionless
The correct Answer is:30 N
To solve the problem, we need to determine the force being applied on the 5 kg block when it is placed on top of the 10 kg block, given that the coefficient of friction between the two blocks is 0.5 and the friction force between them is 20 N.
1. Identify the Forces Acting on the 5 kg Block:
- The weight of the 5 kg block (W1) is given by:
W1=m1⋅g=5kg⋅9.8m/s2=49N
- The normal force (N) acting on the 5 kg block from the 10 kg block is equal to its weight:
N=W1=49N
2. Calculate the Maximum Static Friction:
- The maximum static friction (f_max) between the two blocks can be calculated using the coefficient of friction (μ):
fmax=μ⋅N=0.5⋅49N=24.5N
- Since the friction force given is 20 N, which is less than 24.5 N, the blocks do not slip relative to each other.
3. Determine the Common Acceleration:
- The total mass of the system (both blocks) is:
mtotal=m1+m2=5kg+10kg=15kg
- The net force acting on the system is the friction force (20 N) acting on the 10 kg block. Thus, we can find the common acceleration (a):
a=ffrictionmtotal=20N15kg=43m/s2≈1.33m/s2
4. Calculate the Force Applied on the 5 kg Block:
- The force (F) applied on the 5 kg block must overcome the friction force acting on the 10 kg block and also provide the necessary acceleration to both blocks:
F=m1⋅a+ffriction=5kg⋅43m/s2+20N
- Calculate:
F=203N+20N=20+603=803N≈26.67N
Final Answer:
The force being applied on the 5 kg block is approximately 26.67 N.
- The weight of the 5 kg block (W1) is given by:
W1=m1⋅g=5kg⋅9.8m/s2=49N
- The normal force (N) acting on the 5 kg block from the 10 kg block is equal to its weight:
N=W1=49N
- The maximum static friction (f_max) between the two blocks can be calculated using the coefficient of friction (μ):
fmax=μ⋅N=0.5⋅49N=24.5N
- Since the friction force given is 20 N, which is less than 24.5 N, the blocks do not slip relative to each other.
- The total mass of the system (both blocks) is:
mtotal=m1+m2=5kg+10kg=15kg
- The net force acting on the system is the friction force (20 N) acting on the 10 kg block. Thus, we can find the common acceleration (a):
a=ffrictionmtotal=20N15kg=43m/s2≈1.33m/s2
- The force (F) applied on the 5 kg block must overcome the friction force acting on the 10 kg block and also provide the necessary acceleration to both blocks:
F=m1⋅a+ffriction=5kg⋅43m/s2+20N
- Calculate:
F=203N+20N=20+603=803N≈26.67N
The force being applied on the 5 kg block is approximately 26.67 N.
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (S-2)9videos
NEWTON'S LAWS OF MOTION & FRICTION
- BOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (O-1)51videos
NEWTON'S LAWS OF MOTION & FRICTION
- BOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)4videos
NEWTON'S LAWS OF MOTION & FRICTION
- BOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE28videos
MOTION IN A PALNE
- BOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III28videos
NEWTONS LAWS OF MOTION
Similar Questions
Coefficient of friction between 5kg and 10 kg block is 0.5 and that between floor and 10 kg block is 0.8 if friction between floor and 10 kg block is 20 N what is the value of force (in N) being applied on 5 kg
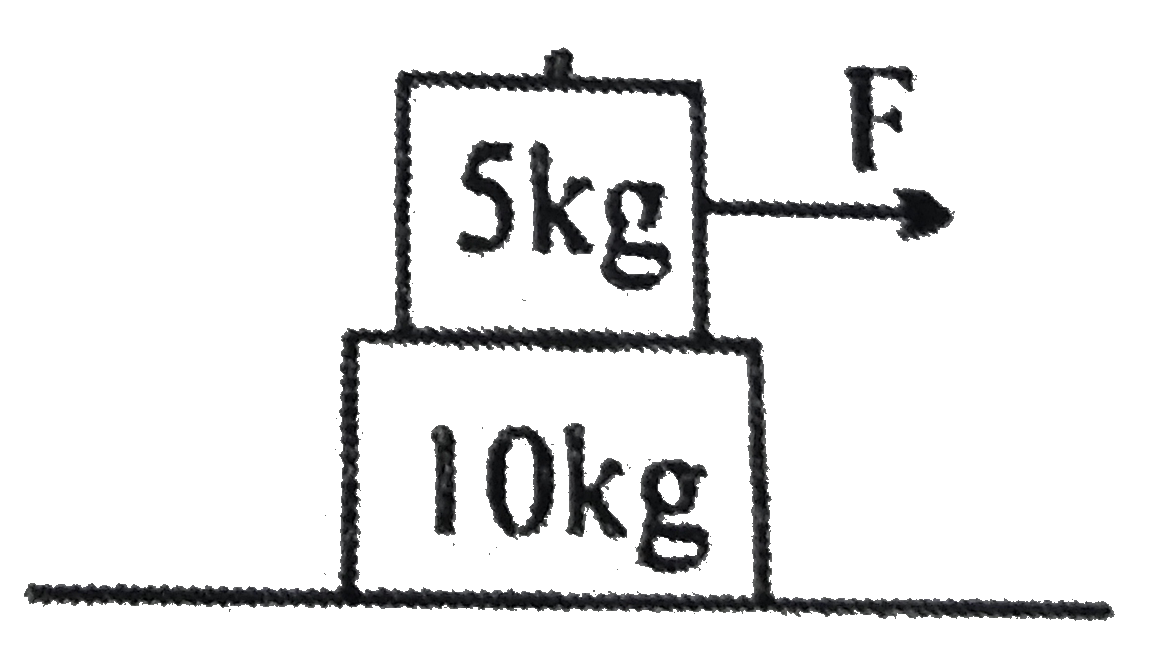 View Solution
View SolutionBlock A of mass 2kg is placed over a block B of mass 8kg . The combination is placed on a rough horizontal surface. If g=10ms−2 , coefficient of friction between B and floor =0.5 , coefficient of friction between B and floor =0.5 , coefficient of friction between A and B=0.4 and a horizontal force of 10N is applied on 8kg block, then the force of friction between A and B is.
 View Solution
View SolutionBlock A of mass 2kg is placed over a block B of mass 8kg . The combination is placed on a rough horizontal surface. If g=10ms−2 , coefficient of friction between B and floor =0.5 , coefficient of friction between B and floor =0.5 , coefficient of friction between A and B=0.4 and a horizontal force of 10N is applied on 8kg block, then the force of friction between A and B is.
 View Solution
View SolutionThe coefficient of friction between 4 kg and 5kg blocks is 0.2 and between 5kg block and grond is 0.1 respectively Choose the correct statements.
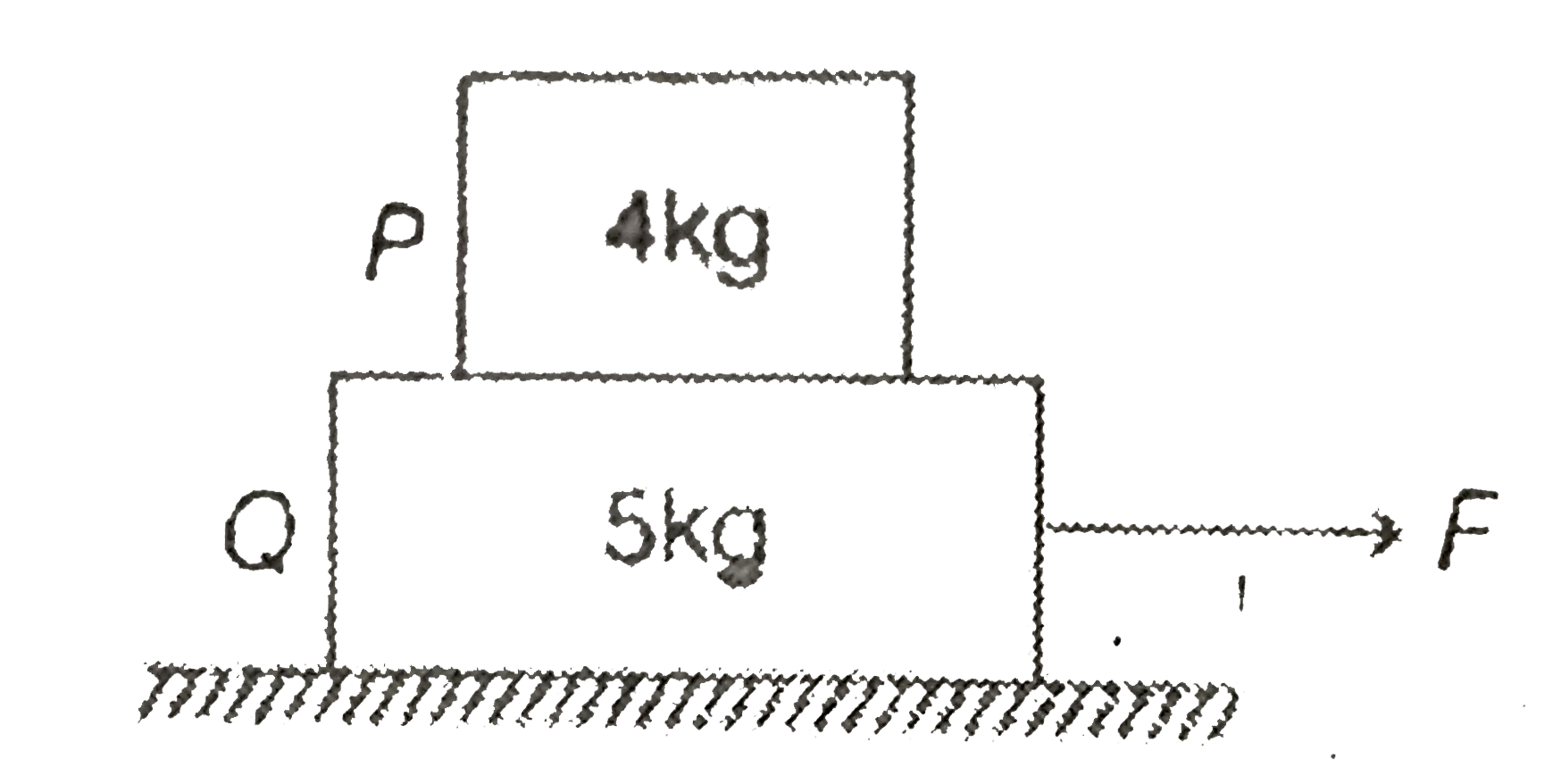 View Solution
View SolutionA block A of mass 2kg rests on another block B of mass 8kg which rests on a horizontal floor. The coefficient of friction between A and B is 0.2 while that between B and floor is 0.5.When a horizontal floor F of 25N is applied on the block B the force of friction between A and B is
View Solution
When F = 2N, the frictional force between 10 kg block 5 kg block isView SolutionThe coefficient of friction between 4kg and 5kg blocks is 0.2 and between 5kg block, ground is 0.1 respectively. The minimum force 'F' required for slipping between 4kg and 5kg blocks is n×3 the value of n is.
 View Solution
View SolutionIn the diagram shown coefficient of friction between 2 kg block and 4 kg block is μ=0.1 and between 4 kg and ground is μ=0.2 then answer the following quwstions .(tis time)
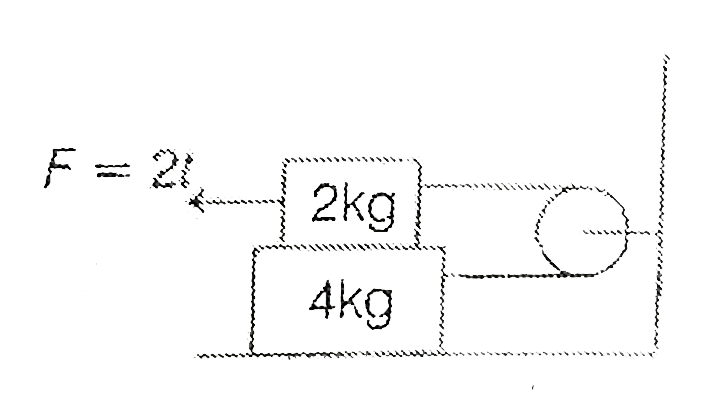
Force of friction on 2 kg block at t=12 s will beView SolutionIn the diagram shown coefficient of friction between 2 kg block and 4 kg block is μ=0.1 and between 4 kg and ground is μ=0.2 then answer the following quwstions .(tis time)

At t=4 s, the friction between 4 kg block and ground will beView SolutionA block of mass 2 kg is at rest on a floor . The coefficient of static friction between block and the floor is 0.54. A horizonatl force of 2.8 N is applied to the block . What should be the frictional force between the block and the floor ? ( take , g=10m/s2)
View Solution

