In a certain system of absoulte units the acceleration produced by gravity in a body falling freely is denoted by 5, the kinetic energy of a 500 kg shot moving with velocity 400 metres per second is denoted by 2000 & its momentum by 100
The unit of length is :-
15 m
50 m
25 m
100 m
The correct Answer is:B
To solve the problem, we need to find the unit of length in a certain system of absolute units based on the given information about acceleration due to gravity, kinetic energy, and momentum.
1. Understanding the Given Information:
- The acceleration due to gravity (g) is denoted as 5 units.
- The kinetic energy (KE) of a 500 kg shot moving with a velocity of 400 m/s is denoted as 2000 units.
- The momentum (p) of the same shot is denoted as 100 units.
2. Relating the Units:
- We know that in standard SI units:
- Acceleration g=10m/s2
- Kinetic Energy KE=12mv2
- Momentum p=mv
3. Setting Up Equations:
- For acceleration:
g=LT2=10m/s2(Equation 1)
Given that g is denoted by 5 units, we can write:
LT2=5(Equation 2)
- For kinetic energy:
KE=12mv2=12×500×(400)2
Calculating this gives:
KE=12×500×160000=40000000J(in SI units)
In the given system, this is denoted as 2000 units:
KE=12mv2=2000(Equation 3)
- For momentum:
p=mv=500×400=200000kg m/s(in SI units)
In the given system, this is denoted as 100 units:
p=mv=100(Equation 4)
4. Solving the Equations:
- From Equation 1, we can express L in terms of T:
L=10T2(from SI units)
- From Equation 2, we have:
L=5T2(in given units)
- Equating both expressions for L:
10T2=5T2
This implies:
T2=2⇒T=√2
5. Finding Length:
- Now substituting T back into the expression for L:
L=10T2=10×2=20units of length
6. Conclusion:
- The unit of length in this system is 20 units.
- The acceleration due to gravity (g) is denoted as 5 units.
- The kinetic energy (KE) of a 500 kg shot moving with a velocity of 400 m/s is denoted as 2000 units.
- The momentum (p) of the same shot is denoted as 100 units.
- We know that in standard SI units:
- Acceleration g=10m/s2
- Kinetic Energy KE=12mv2
- Momentum p=mv
- For acceleration:
g=LT2=10m/s2(Equation 1)
Given that g is denoted by 5 units, we can write:
LT2=5(Equation 2)
KE=12mv2=12×500×(400)2
Calculating this gives:
KE=12×500×160000=40000000J(in SI units)
In the given system, this is denoted as 2000 units:
KE=12mv2=2000(Equation 3)
p=mv=500×400=200000kg m/s(in SI units)
In the given system, this is denoted as 100 units:
p=mv=100(Equation 4)
- From Equation 1, we can express L in terms of T:
L=10T2(from SI units)
- From Equation 2, we have:
L=5T2(in given units)
- Equating both expressions for L:
10T2=5T2
This implies:
T2=2⇒T=√2
- Now substituting T back into the expression for L:
L=10T2=10×2=20units of length
- The unit of length in this system is 20 units.
Topper's Solved these Questions
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (O-1) ParagraphType Questions.
2 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (O-1) Matrix match type Questions.
2 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (O-1) Multiple Correct Type Questions.
3 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - MATHS
14 Videos
- WAVE OPTICSBOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 2 (Previous Year Questions)
7 Videos
Similar Questions
n a certain system of absoulte units the acceleration produced by gravity in a body falling freely is denoted by 5, the kinetic energy of a 500 kg shot moving with velocity 400 metres per second is denoted by 2000 & its momentum by 100
The unit of time is :-
View SolutionIn a certain system of absolute units the acceleration produced by gravity in a body falling freely is denoted by 5, the kinetic energy of a 500 kg shot moving with velocity 400 metres per second is denoted by 2000 & its momentum by 100
The unit of mass is :-
View SolutionIn a certain system of absolute units the acceleration produced by gravity in a body falling freely is denoted by 3, the kinetic energy of a 272.1kg shot moving with velocity 448 metres per second is denoted by 100, and its momentum by 10.
The unit of length is
View SolutionIn a certain system of absolute units the acceleration produced by gravity in a body falling freely is denoted by 3, the kinetic energy of a 272.1kg shot moving with velocity 448 metres per second is denoted by 100, and its momentum by 10.
the unit of mass is
View SolutionIn ancient time, deffernt system of units was following in which unit of mass was "ser", unit of length was "gaj" & unit of time was "kall" 1ser=900gm, 1gaj=90cm, 1kaal=3hr. A ball of mass 9kg is moving with velocity 10m/s. Then its momentum (mv) in ancient system will be :−
View SolutionThe word fluid means a substance having particles which readily of its magnitude (a small shear stress, which may appear to be of negligible will cause deformation in the fluid). Fluids are charactrised by such properties as density. Specific weight, specific gravity, viscosity etc. Density of a substance is defined as mass per unit volume and it is denoted by. The specific gravity represents a numerical ratio of two densities, and water is commonly taken as a reference substance. Specific gravity of a substance in written as the ratio of density of substance to the density of water. Specific weight represents the force exerted by gravity on a unit volume of fluid. It is related to the density as the product of density of a fluid and acceleration due to gravity. Viscosity is the most important and is recognized as the only single property which influences the fluid motion to a great extent. The viscosity is the property by virtue of which a fluid offers resistance to deformation under the influenece if shear force. The force between the layers opposing relative motion between them are known as forces of viscosity. When a boat moves slowly on the river remains at rest. Velocities of different layers are different. Let v be the velocity of the level at a distance y from the bed and V+dv be the velocity at a distance y+dy. The velocity differs by dv in going through a distance by perpendicular to it. The quantity dvdy is called velocity gradient. The force of viscosity between two layers of a fluid is proportional to velocity gradient and Area of the layer.
F∝A & F∝dvdy
F=−ηAdvdy (−ve sign shown the force is frictional in nature and opposes relative motion. η coefficient of dynamic viscosity
Shear stress FA=−ηdvdy
and simultaneously kinematic viscosity is defined as the dynamic viscosity divided by the density. If is denoted as v. The viscosity of a fluid depends upon its intermolecular structure. In gases, the molecules are widely spaced resulting in a negligible intermolecular cohesion, while in liquids the molecules being very close to each other, the cohesion is much larger with the increases of temperature, the cohesive force decreases rapidly resulting in the decreases of viscosity. In case of gases, the viscosity is mainly due to transfer of molecular momentum in the transerve direction brought about by the molecular agitation. Molecular agitation increases with rise in temperature. Thus we conclude that viscosity of a fluid may thus be considered to be composed of two parts, first due to intermolecuar cohesion and second due to transfer of molecular momentum.
If the velocity profile is given by
v=23y−y2v is velocity in m/secy is in meter above the bad. Determine shear stress at y=0.15m, & η=0.863Ns/m2
View SolutionThe word fluid means a substance having particles which readily of its magnitude (a small shear stress, which may appear to be of negligible will cause deformation in the fluid). Fluids are charactrised by such properties as density. Specific weight, specific gravity, viscosity etc. Density of a substance is defined as mass per unit volume and it is denoted by. The specific gravity represents a numerical ratio of two densities, and water is commonly taken as a reference substance. Specific gravity of a substance in written as the ratio of density of substance to the density of water. Specific weight represents the force exerted by gravity on a unit volume of fluid. It is related to the density as the product of density of a fluid and acceleration due to gravity. Viscosity is the most important and is recognized as the only single property which influences the fluid motion to a great extent. The viscosity is the property by virtue of which a fluid offers resistance to deformation under the influenece if shear force. The force between the layers opposing relative motion between them are known as forces of viscosity. When a boat moves slowly on the river remains at rest. Velocities of different layers are different. Let v be the velocity of the level at a distance y from the bed and V+dv be the velocity at a distance y+dy. The velocity differs by dv in going through a distance by perpendicular to it. The quantity dvdy is called velocity gradient. The force of viscosity between two layers of a fluid is proportional to velocity gradient and Area of the layer.
F∝A & F∝dvdy
F=−ηAdvdy (−ve sign shown the force is frictional in nature and opposes relative motion. η coefficient of dynamic viscosity
Shear stress FA=−ηdvdy
and simultaneously kinematic viscosity is defined as the dynamic viscosity divided by the density. If is denoted as v. The viscosity of a fluid depends upon its intermolecular structure. In gases, the molecules are widely spaced resulting in a negligible intermolecular cohesion, while in liquids the molecules being very close to each other, the cohesion is much larger with the increases of temperature, the cohesive force decreases rapidly resulting in the decreases of viscosity. In case of gases, the viscosity is mainly due to transfer of molecular momentum in the transerve direction brought about by the molecular agitation. Molecular agitation increases with rise in temperature. Thus we conclude that viscosity of a fluid may thus be considered to be composed of two parts, first due to intermolecuar cohesion and second due to transfer of molecular momentum.
Viscosity of liquids
View SolutionThree spheres, each of mass m, can slide freely on a frictionless, horizontal surface. Spheres A and B are attached to an inextensible, inelastic cord of length l and are at rest in the position shown where sphere B is struck by sphere C which is moving to the right with a velocity v0. Knowing that the cord is taut where sphere B is struck by sphere C and assuming 'head on' inelastic impact between B and C, we cannot conserve kinetic energy of the entire system.
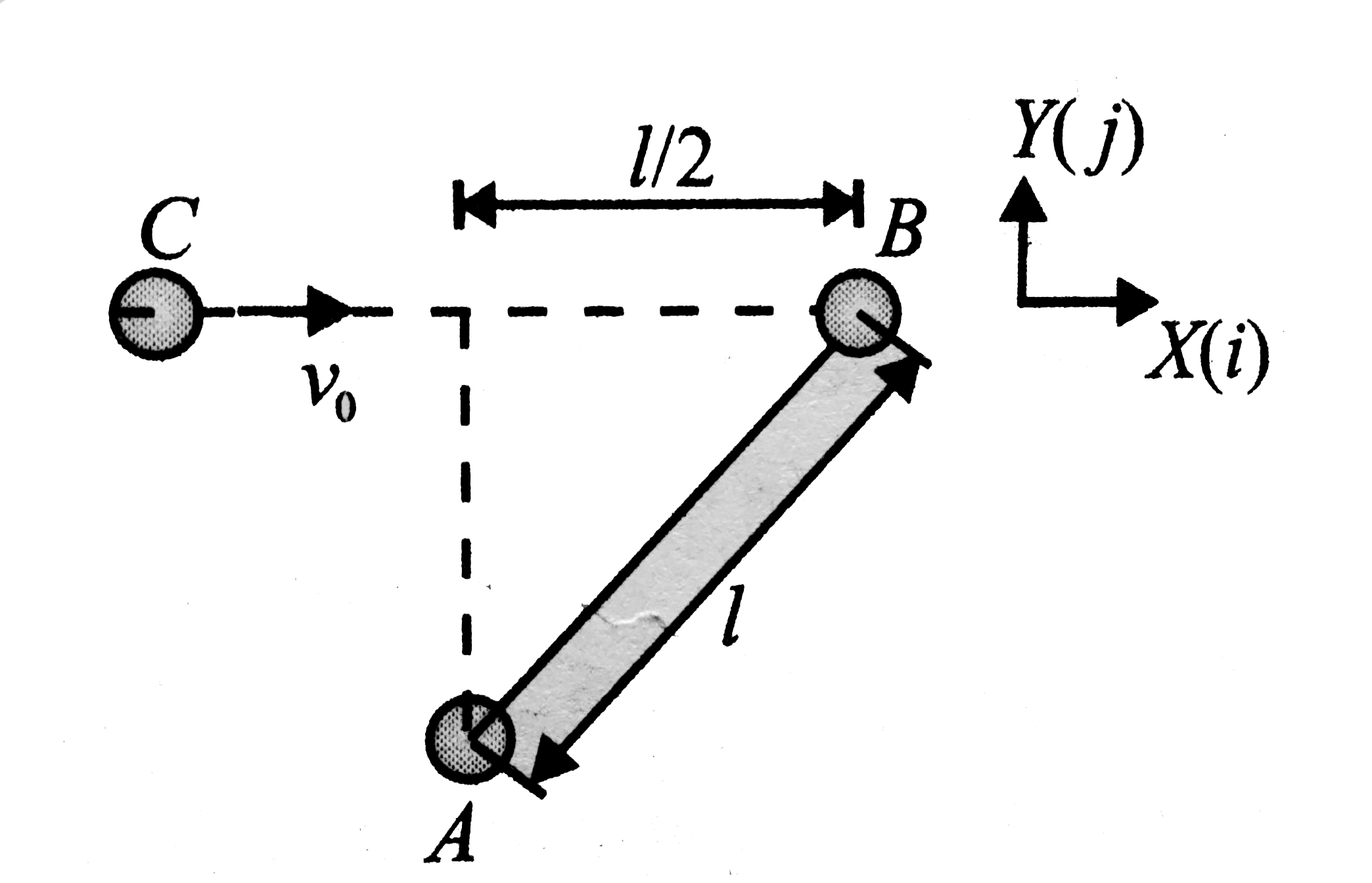 The velocity of B immediately after collision is along unit vector
The velocity of B immediately after collision is along unit vector
View SolutionA thin uniform bar lies on a frictionless horizonta surface and is free to move in any way on th surface. Its mass is 0.16 kg and length √3meters. Two particless, each of mass 0.08 kg, are moving on the same surface and towards the bar in a direction perpendicular to the bar, one with a velocity of 10 m/s, and other with 6m/s as shown in fig. The first particle strikes the bar at point A and the other at point B. Points A and B are at a distane of 0.5m from the centre of the bar. The particles strike the bar at the same instant of time and stick to the bar on collision. Calculate the loss of the kinetic energy of the system in the above collision process.
View SolutionIn a metal in the solid state, such as a copper wire, the atoms are strongly bound to one another and occupý fixed positions. Some electrons (called the conductor electrons) are free to move in the body of the metal while the other are strongly bound to their atoms. In good conductors, the number of free electrons is very large of the order of 1028 electrons per cubic metre in copper. The free electrons are in random motion and keep colliding with atoms. At room temperature, they move with velocities of the order of 105 m/s. These velocities are completely random and there is not net flow of charge in any directions. If a potential difference is maintained between the ends of the metal wire (by connecting it across a battery), an electric field is set up which accelerates the free electrons: These accelerated electrons frequently collide with the atoms of the conductor, as a result, they acquire a constant speed called the drift speed which is given by Ve= 1/enA where I = current in the conductor due to drifting electrons, e = charge of electron, n = number of free electrons per unit volume of the conductor and A = area of cross-section of the conductor. A uniform wire of length 2.0 m and cross-sectional area 10−7m2 carries a current of 1.6 A. If there are 1028 free electrons per m in copper, the drift speed of electrons in copper is
View Solution
