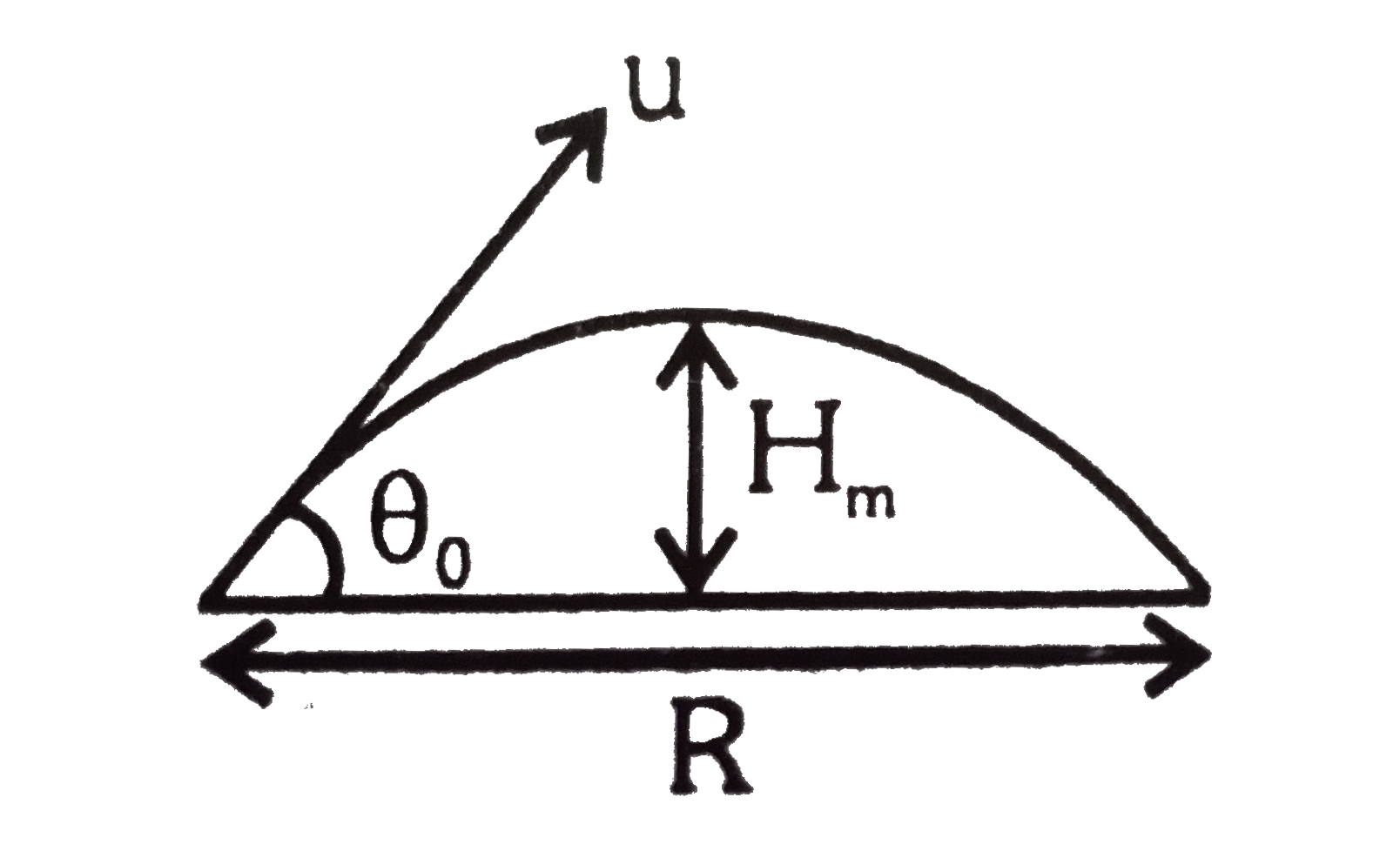
Show that projection angle 0o for a projectile launched from the origin is given by :
0o=tan−1J[4HmR] where Hm =Maximum height, R = Range.
Hm=u2sin2θ02g and R=2u2g×sinθ0×cosθ0
So, HmR=tanθ04⇒tanθ0=4HmR⇒=tan−1(4HmR)
Topper's Solved these Questions
Similar Questions
Show that projection angle θ0 for a projectile launched from the origin is given by:
θ0=tan−1[4HmR] Where Hm= Maximum height, R=Range
View SolutionShown that for a projectile the angle between the velocity and the x-axis as a function of time is given by
θ(t)=tan−1(voy−>vax)
(b) Show that the projection angle θ0 for a projectile lauched from the origin is given by
θ0=(tan−1(4hm)R)
Where the symbols have their usual meaning.
View Solution
Knowledge Check
Maximum range for a projectile motion is given as R, then height will be
ARB2 RCR/4DR/2
Show that for a projectile the angle between the velocity and the x-axis as function of time is given
by θt=tan−1(v0y−gtvox)
(b) Shows that the projection angle θ0 for a projectile launched from the origin is given by θt=tan−1(4hmR)
where the symbols have their usual meanings.
View SolutionThe position of a projectile launched from the origin at t = 0 is given by s=(40ˆi+50ˆj)mat t=2s. if the projectile was launched at an angle θ from the horizontal, then θis (take g = 10 ms–2
View SolutionFind the angle of projection for a projectile motion whose rang R is (n) times the maximum height H.
View SolutionShow that the maximum height reached by a projectile launched at an angle of 45∘ is one quarter of its range.
View SolutionFor a projectile 'R' is range and 'H' is maximum height

View SolutionA projectile is fired from a horizontal frictionless ground. Coefficient of restitution between the projectile and the ground is e. If T1,H1,R1,v1,T2,H2,R2,v2, are time flight, maximum height, range, horizontal velocities in first two collisions, then match the following.

View SolutionTwo particles projected form the same point with same speed u at angles of projection αandβ strike the horizontal ground at the same point. If h1andh2 are the maximum heights attained by the projectile, R is the range for both and t1andt2 are their times of flights, respectively, then incorrect option is :
View Solution
