A heterozygous individual ++ab is crossed to its revessive parent and has produced the offsprings in the following proportion.
++/ab−200
a+/ab−50
+b/ab−30
ab/ab−100
What is the expected distance between the two gene loci ?
82 cM
18 cM (cis)
20 cM
18 cM (trans)
The correct Answer is:D
Step by step video & image solution for A heterozygous individual ++ab is crossed to its revessive parent and has produced the offsprings in the following proportion. ++//ab-200 a+//ab-50 +b//ab-30 ab//ab-100 What is the expected distance between the two gene loci ? by Biology experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GENETICS & MOLECULAR BASIS OF INHERITANCE AND MUTATIONBOOK - ALLENCHAPTER - GENETICS & MOLECULAR BASIS OF INHERITANCE AND MUTATIONEXERCISE - EXERCISE-IV (ASSERTION & REASON)
144 Videos
- GENETICS & MOLECULAR BASIS OF INHERITANCE AND MUTATIONBOOK - ALLENCHAPTER - GENETICS & MOLECULAR BASIS OF INHERITANCE AND MUTATIONEXERCISE - EXERCISE-II (PREVIOUS YEAR QUESTIONS
104 Videos
- GENETICS BOOK - ALLENCHAPTER - GENETICS EXERCISE - Question
58 Videos
- GENETICS (PRINCIPLES OF INHERITANCE AND VARIATION) (MOLECULAR BASIS OF INHERITANCE)BOOK - ALLENCHAPTER - GENETICS (PRINCIPLES OF INHERITANCE AND VARIATION) (MOLECULAR BASIS OF INHERITANCE)EXERCISE - Question
53 Videos
Similar Questions
Knowledge Check
The percentage of ab gamete produced by AaBb parent will be
A25%B50%C75%D12.5%
Find the degrees of the following polynomials
5b3+2ab2−4ab+3
View SolutionIndividuals homozygous for ab genes were crossed with wild type (++). The F1 hybrid thus produced was test crossed. It produces progeny in following proportion.
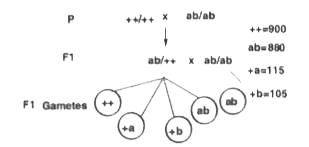
Now, calculate the distance between a and b genes.
View SolutionIf A and B are two matrices such that A+B and AB are both defind, then
View SolutionIf AB=A and BA=B then which of the following is/are true ?
View SolutionA and B genes are linked. What shall be the genotype of progeny in a cross between AB/ab and ab/ab?
View SolutionIf A.B=A×B, then angle between A and B is
View SolutionIf A and B are two matrices such that their product AB is
a null matrix, then
View Solution
