The graph of function y=1+cosx will be



None of these
The correct Answer is:A
To analyze the graph of the function y=1+cosx, we can follow these steps:
Step 1: Understand the basic graph of cosx
The cosine function, cosx, oscillates between -1 and 1. Its graph is a wave that crosses the y-axis at (0,1) and has a period of 2π.
Step 2: Shift the graph of cosx
Since we are looking at the function y=1+cosx, we need to shift the entire graph of cosx upwards by 1 unit. This means every point on the graph of cosx will be increased by 1.
Step 3: Determine the new range of the function
The original range of cosx is from -1 to 1. By adding 1 to each value, the new range becomes:
- Minimum value: −1+1=0
- Maximum value: 1+1=2
Thus, the range of y=1+cosx is from 0 to 2.
Step 4: Sketch the graph
1. Start by plotting the key points of cosx:
- At x=0, y=1+cos(0)=2
- At x=π, y=1+cos(π)=0
- At x=2π, y=1+cos(2π)=2
2. The graph will oscillate between the points (0, 2), (π, 0), and (2π, 2).
3. The graph will be a wave-like shape that touches the y-axis at 0 and 2, never going below 0.
Step 5: Confirm the characteristics of the graph
- The graph of y=1+cosx will not have any negative values since the minimum value is 0.
- The graph will repeat every 2π due to the periodic nature of the cosine function.
Final Graph Description
The graph of y=1+cosx is a wave that oscillates between 0 and 2, starting at the maximum value of 2 when x=0 and reaching the minimum value of 0 at x=π.
---
The cosine function, cosx, oscillates between -1 and 1. Its graph is a wave that crosses the y-axis at (0,1) and has a period of 2π.
Since we are looking at the function y=1+cosx, we need to shift the entire graph of cosx upwards by 1 unit. This means every point on the graph of cosx will be increased by 1.
The original range of cosx is from -1 to 1. By adding 1 to each value, the new range becomes:
- Minimum value: −1+1=0
- Maximum value: 1+1=2
1. Start by plotting the key points of cosx:
- At x=0, y=1+cos(0)=2
- At x=π, y=1+cos(π)=0
- At x=2π, y=1+cos(2π)=2
2. The graph will oscillate between the points (0, 2), (π, 0), and (2π, 2).
3. The graph will be a wave-like shape that touches the y-axis at 0 and 2, never going below 0.
- The graph of y=1+cosx will not have any negative values since the minimum value is 0.
- The graph will repeat every 2π due to the periodic nature of the cosine function.
The graph of y=1+cosx is a wave that oscillates between 0 and 2, starting at the maximum value of 2 when x=0 and reaching the minimum value of 0 at x=π.
Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Co-ordinate & Algebra)
37 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (VECTORS)
60 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Integration)
12 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
Knowledge Check
What is the period of the graph of the function y=sinx1+cosx ?
A4πB2πCπDπ2
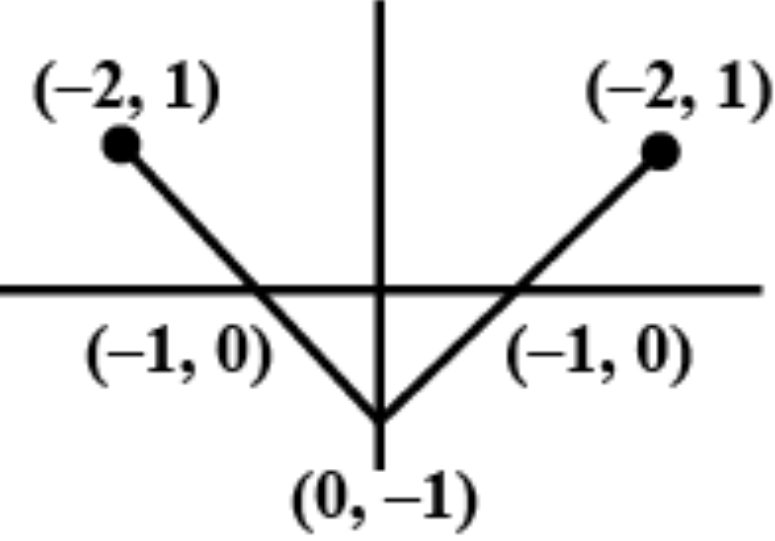
If the graph of the function y=f(x) is as shown :

the graph of y=12(|f(x)|−f(x)) is
View SolutionThe graph of the function y=f(x). Then which of the following curve represent the graph of the function y=|f(x)|
View SolutionThe graph of the function y=f(x) passing through the point (0,1) and satisfying the differential equation dydx+ycosx=cosx is such that (a) it is a constant function. (b) it is periodic (c) it is neither an even nor an odd function. (d) it is continuous and differentiable for all f(x)
View SolutionThe graph of the function y=f(x) is shown.

Find the number of solutions to the equation ||f(x)|−1|=12.
View SolutionDraw the graph of the function y=[x] in −2<x<4
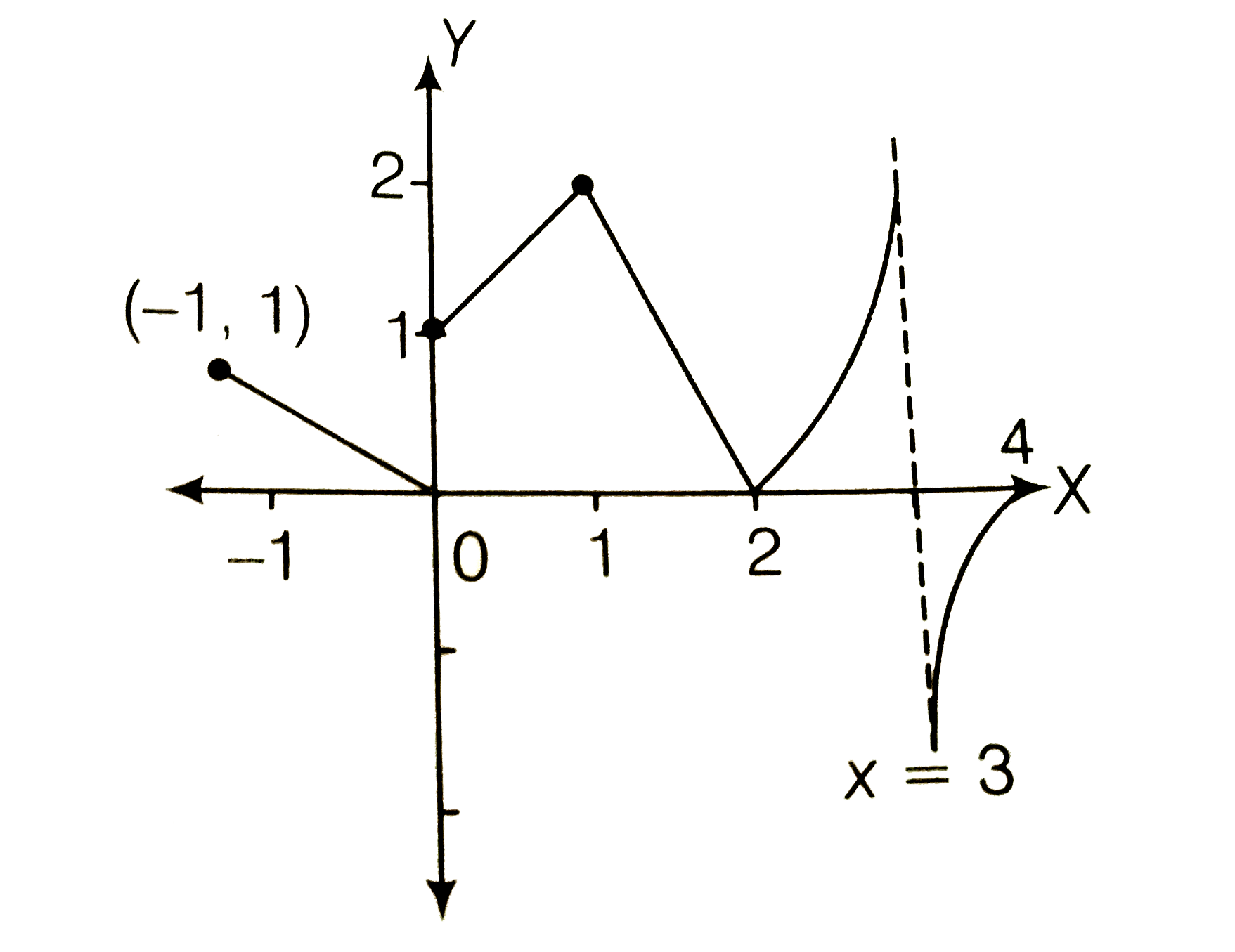
View SolutionThe graph of the function y=f(x) is shown in the adjacent figure then correct statement is
View SolutionDraw the graph of function y=|2−|x−2∣∣.
View Solution
