The area of cross section of a steel wire (Y=2.0×1011N/m2) is 0.1cm2. The force required to double is length will be
2×1012 N
2×1011 N
2×1010 N
2×106 N
The correct Answer is:D
To solve the problem of finding the force required to double the length of a steel wire, we can follow these steps:
1. Understand the Given Data:
- Young's Modulus (Y) = 2.0×1011N/m2
- Area of Cross Section (A) = 0.1cm2=0.1×10−4m2 (conversion from cm² to m²)
- Original Length (L) = L (let's denote it as L for simplicity)
- New Length = 2L (since we want to double the length)
2. Calculate Change in Length:
- Change in Length (ΔL) = New Length - Original Length = 2L−L=L
3. Use the Formula for Young's Modulus:
- The formula for Young's Modulus is given by:
Y=StressStrain
- Stress is defined as:
Stress=FA
- Strain is defined as:
Strain=ΔLL
4. Rearranging the Young's Modulus Formula:
- We can express the force (F) in terms of Young's Modulus, area, change in length, and original length:
Y=F/AΔL/L
- Rearranging gives:
F=Y⋅A⋅ΔLL
5. Substituting Values:
- Substitute the known values into the equation:
F=(2.0×1011N/m2)⋅(0.1×10−4m2)⋅LL
- Notice that L cancels out:
F=(2.0×1011)⋅(0.1×10−4)=2.0×1011⋅10−5=2.0×106N
6. Final Answer:
- The force required to double the length of the steel wire is:
F=2.0×106N
- Young's Modulus (Y) = 2.0×1011N/m2
- Area of Cross Section (A) = 0.1cm2=0.1×10−4m2 (conversion from cm² to m²)
- Original Length (L) = L (let's denote it as L for simplicity)
- New Length = 2L (since we want to double the length)
- Change in Length (ΔL) = New Length - Original Length = 2L−L=L
- The formula for Young's Modulus is given by:
Y=StressStrain
- Stress is defined as:
Stress=FA
- Strain is defined as:
Strain=ΔLL
- We can express the force (F) in terms of Young's Modulus, area, change in length, and original length:
Y=F/AΔL/L
- Rearranging gives:
F=Y⋅A⋅ΔLL
- Substitute the known values into the equation:
F=(2.0×1011N/m2)⋅(0.1×10−4m2)⋅LL
- Notice that L cancels out:
F=(2.0×1011)⋅(0.1×10−4)=2.0×1011⋅10−5=2.0×106N
- The force required to double the length of the steel wire is:
F=2.0×106N
Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Properties of Matter & Fluid Mechanics)(Fluid Dynamics + Viscosity)
18 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Properties of Matter & Fluid Mechanics)(Hydrostatics)
20 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (GRAVITATION)
25 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
Young 's modulus of steel is 2.0×1011Nm/(2). Express it is dynecm2.
View SolutionThe area of cross - section of a wire is 1 mm2 and it length is 2 m. How much work will be done to increase its length by 0.1 mm ? The Young.s modulus of elaticity for the material of the wire is 2×1011 Nm−2.
View Solution
Knowledge Check
The area of a cross-section of steel wire is 0.1cm−2 and Young's modulus of steel is 2x1011Nm−2. The force required to stretch by 0.1% of its length is
A1000 NB2000 NCÂ 4000 ND5000N
A 6kg weight is fastened to the end of a steel wire of un stretched length 60cm. It is whirled in a vertical circle and has an angular velocity of 2rev/s at the bottom of the circle. The area of cross - section of the wire is 0.05cm2 . Calculate the elongation of the wire when the weight is at the lowest point of the path . Young's modulus of steel =2×1011N/m2 .
View SolutionA copper wire (Y=1011Nm−2) of length 8 m and a steel wire (Y=2×1011Nm−2) of length 4 m, each of 0.5cm2 cross-section are fastened end to end and stretched with a tension of 500 N. choose the correct option.
View SolutionThe resistivity of a potentiometer wire is 40×10−8Ω−m and its area of cross section is 8×10−6m2. If 0.2 A current is flowing through the wire the potential gradient will be
View SolutionA steel wire 4.0m in length is stretched through 2.0mm.The cross -sectional area of the wire is 2.0mm2.If young's modulus of steel is 2.0×1011N/m2
(a) the energy density of wire,
(b) the elastic potential energy stored in the wire.
View SolutionFind the load on wire of cross section 16 mm to increase its length by 0.3%(Y=5×109N/m2,g=10m/s2)
View SolutionThe length of a rod is 20cm and area of cross-section 2cm2. The Young's modulus of the material of wire is 1.4×1011N/m2. If the rod is compressed by 5kg−wt along its length, then increase in the energy of the rod in joules will be
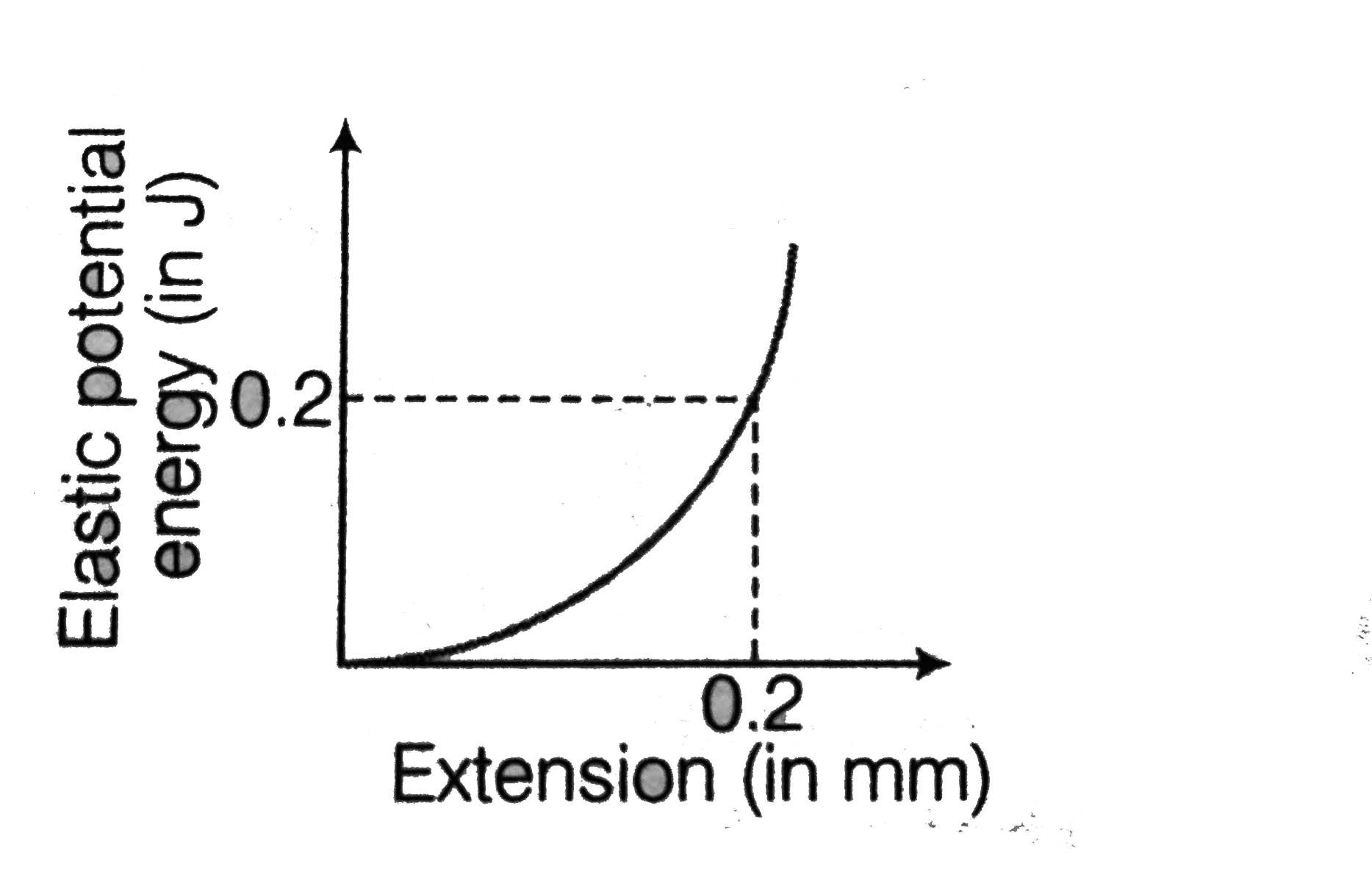
View SolutionFigure shows the graph of elastic potential energy (U) stored versus extension, for a steel wire (Y=2×1011Pa) of volume 200cc. If area of cross- section A and original length L, then
View Solution
